A ring of raidus 5m is lying in the x-y plane is carrying current of 1A in anti-clockwise sence. If uniform magnetic field of magnetic induction →B=3ˆi+4ˆj is switched on in space, then the co-ordinates of point about which the loop will have a tendency to lift up is:
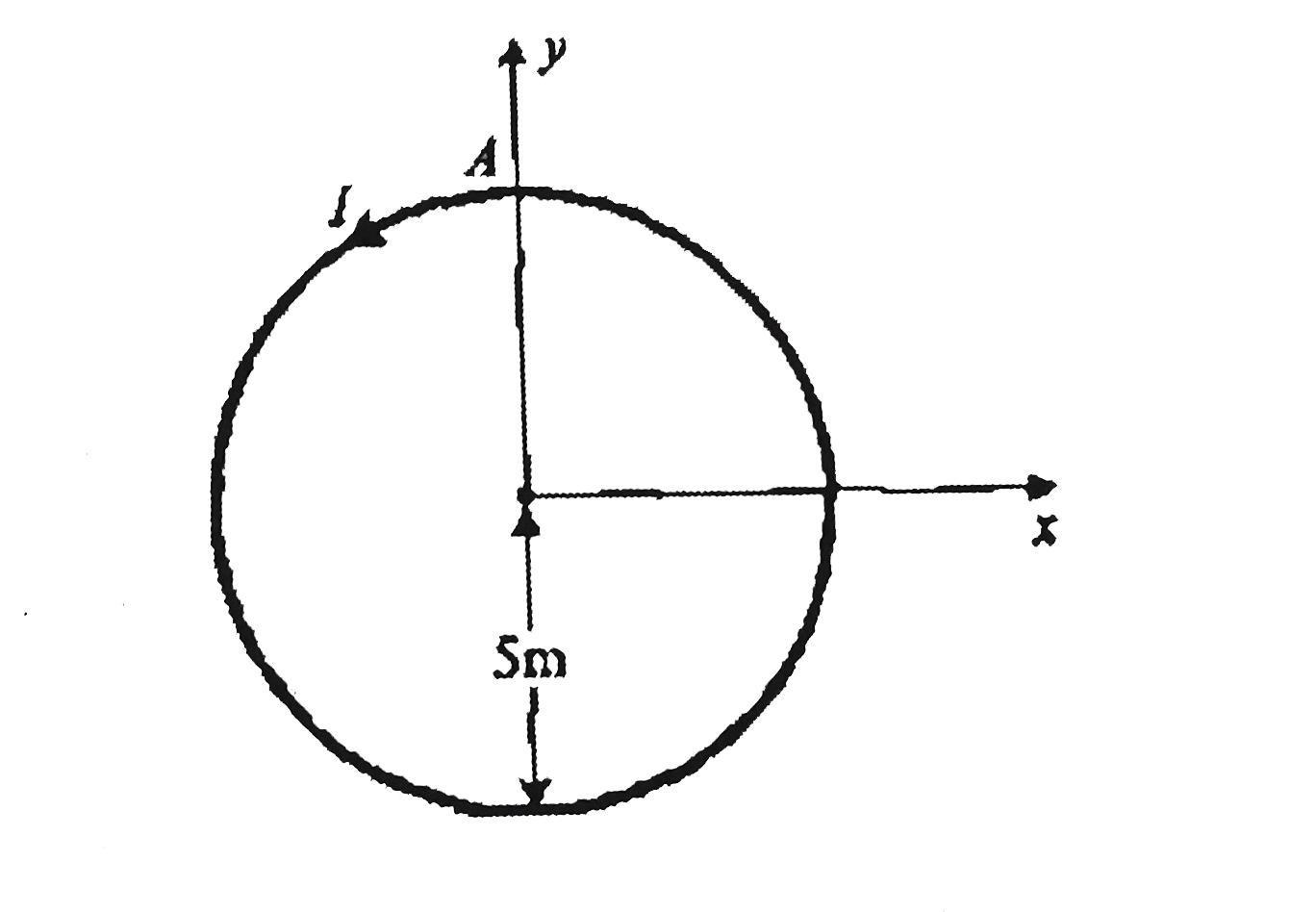
(3,4)
(4,3)
(3,0)
(0,3)
Step by step video & image solution for A ring of raidus 5m is lying in the x-y plane is carrying current of 1A in anti-clockwise sence. If uniform magnetic field of magnetic induction vecB=3hati+4hatj is switched on in space, then the co-ordinates of point about which the loop will have a tendency to lift up is: by Physics experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Topper's Solved these Questions
- MAGNETIC EFFECTS OF CURRENT AND MAGNETISMBOOK - PHYSICS GALAXY - ASHISH ARORACHAPTER - MAGNETIC EFFECTS OF CURRENT AND MAGNETISMEXERCISE - Advance MCQ
29 Videos
- MAGNETIC EFFECTS OF CURRENT AND MAGNETISMBOOK - PHYSICS GALAXY - ASHISH ARORACHAPTER - MAGNETIC EFFECTS OF CURRENT AND MAGNETISMEXERCISE - U.N.P
79 Videos
- MAGNETIC EFFECTS OF CURRENT AND MAGNETISMBOOK - PHYSICS GALAXY - ASHISH ARORACHAPTER - MAGNETIC EFFECTS OF CURRENT AND MAGNETISMEXERCISE - Conceptual MCQ
37 Videos
- Kinetic Theory of Gases and Gas LawsBOOK - PHYSICS GALAXY - ASHISH ARORACHAPTER - Kinetic Theory of Gases and Gas LawsEXERCISE - Unsolved Numerical Problems for Preparation of NSEP, INPhO & IPhO
64 Videos
- NUCLEAR PHYSICS AND RADIOACTIVITYBOOK - PHYSICS GALAXY - ASHISH ARORACHAPTER - NUCLEAR PHYSICS AND RADIOACTIVITYEXERCISE - Concptual MCQs
48 Videos
Similar Questions
A wire loop carrying current I is placed in the x−y plane as shown in the figure. If an external uniform magnetic field →B=Bˆi is switched on, then the torque acting on the loop is
View SolutionA circular loop of radius 0⋅2m carrying a current of 1A is placed in a uniform magnetic field of 0⋅5T. The magnetic field is perpendicular to the plane of the loop. What is the force experienced by the loop?
View Solution
Knowledge Check
A ring of radius 5 m is lying in the x-y plane and is carrying current of 1 A in anticlockwise sense. If a uniform magnetic field −B=3ˆi+4ˆj is switched on, then the coordinates of point about which the loop will lift up is:
 A(3,4)B(4,3)C(3,0)D(0,3)
A(3,4)B(4,3)C(3,0)D(0,3)A circular loop with N turns has radius r . It lies in the x-y plane carrying current I in the anti-clockwise direction . If the magnetic field in the region is →B=B0ˆi , then find the torque (→r) acting on the loop .
AB0NIπr2ˆjBB0Iπr2ˆjCB0NIπr2ˆkDB0πr2atkA conducting loop carrying a current I is placed in a uniform magnetic field ponting into the plane of the paper as shown. The loop will have a tendency to
 AcontractBexpandCmove towards +vex−aξsDmove towards −vex−aξs
AcontractBexpandCmove towards +vex−aξsDmove towards −vex−aξs
A circular loop of area 20cm2 is placed on x-y plane. Containing uniform magnetic field of induction
→B=0.4ˆi+0.3ˆj Tesla
then the magnetic flux linked with the coil is ?
View SolutionA circular loop of radius 4.0 cm is placed in a horizontal plane and carries an electric current of 5.0 A in the clockwise direction as seen from above. Find the magnetic field (a) at a point 3.0 cm above the centre of the loop (b) at a point 3.0 cm below the centre of the loop.
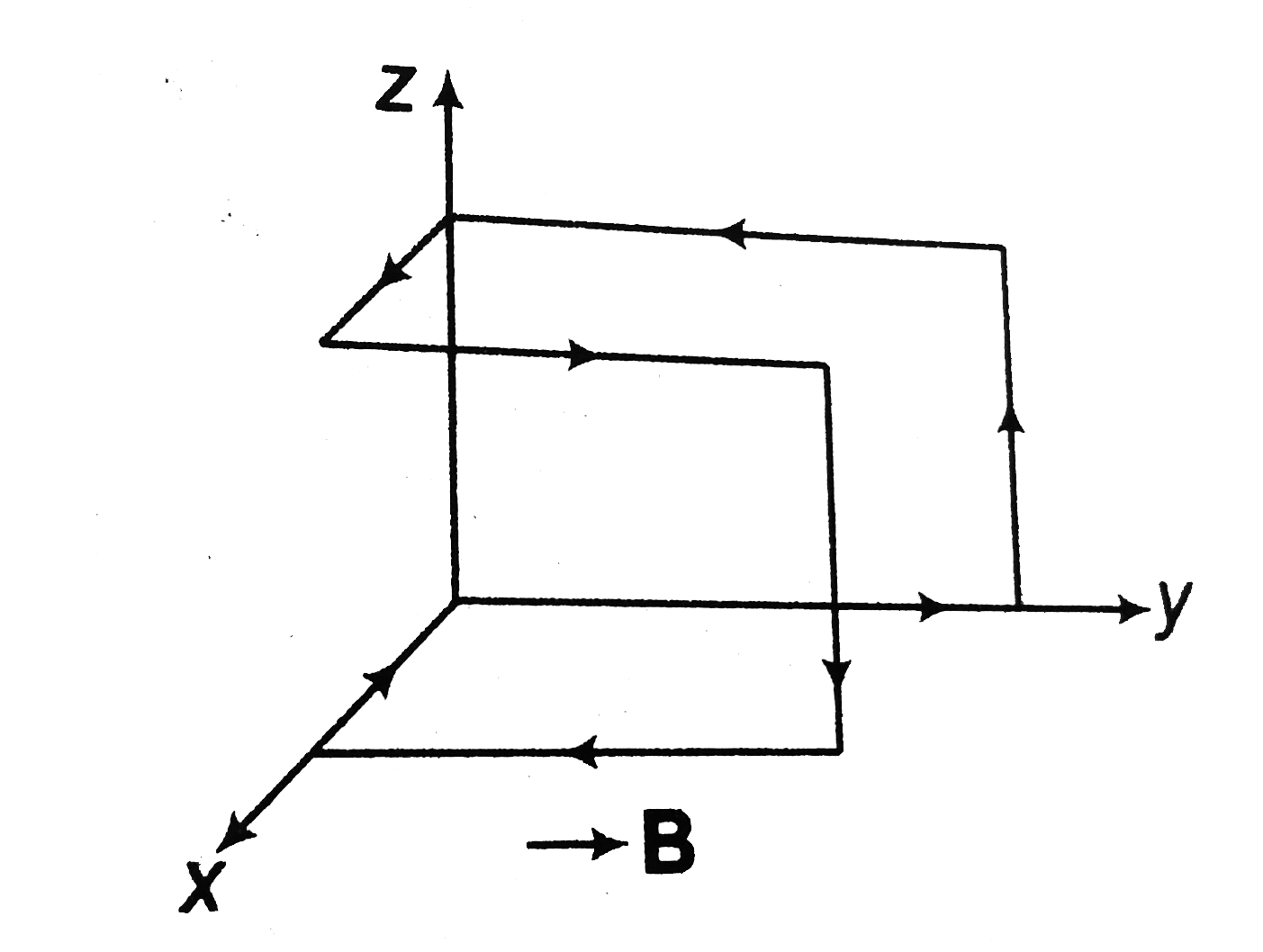
View SolutionGiven figure shows a coil bent with all edges of length 1m and carrying a current of 1A. There exists in space a uniform magnetic field of 2T in positive y-direction. Find the torque on the loop.
View SolutionA ring of mass 'm' and radius 'r' carrying current i0 is lying in the X-Y plane with centre at origin. A uniform magnetic field of strength B=B0(2ˆi−3ˆj+5ˆk)T is applied in the region. If the ring rotates about the axid initial magnetic energy stored in the ring will be. (In joules)
View SolutionA straight wire of length 0.5 m carrying a current of 1.6 ampere is placed in a uniform magnetic field of induction 2 T. If the magnetic field is perendicular to the length of the wire, then force on the wire is
View Solution
