Two identical semicircular discs of mass 'm' each and radius 'R' are placed in the XY (horizontal) plane and the YZ (vertical) plane, respectively. They are so placed that they have their common diameter along the y-axis. Then, the moment of inertia of the system about the appropriate axis is given by ( refers to moment of inertia about axis n-where n is X, Y, Z)
The correct Answer is:B, C
To find the moment of inertia of the system consisting of two identical semicircular discs, we will calculate the moment of inertia about the X, Y, and Z axes step by step.
Step 1: Understanding the Configuration
We have two semicircular discs:
- The first semicircular disc is in the XY plane (horizontal).
- The second semicircular disc is in the YZ plane (vertical).
Both discs have a common diameter along the Y-axis.
Step 2: Moment of Inertia about the X-axis (I_x)
1. For the first disc (in the XY plane):
- The moment of inertia of a semicircular disc about an axis perpendicular to its plane and passing through its center is given by:
Ix1=mR22
2. For the second disc (in the YZ plane):
- The moment of inertia about the X-axis can be found using the perpendicular axis theorem. The moment of inertia about the Z-axis (perpendicular to the plane) is:
Iz2=mR22
- Using the perpendicular axis theorem:
Iz2=Iy2+Ix2⇒Ix2+Iy2=mR22
- Since Ix2=Iy2 (both axes are along the diameter of the disc):
2Ix2=mR22⇒Ix2=mR24
3. Total moment of inertia about the X-axis:
Ix=Ix1+Ix2=mR22+mR24=3mR24
Step 3: Moment of Inertia about the Y-axis (I_y)
1. For the first disc (in the XY plane):
- The Y-axis is in the plane of the first disc, so:
Iy1=mR24
2. For the second disc (in the YZ plane):
- The Y-axis is also in the plane of the second disc, so:
Iy2=mR24
3. Total moment of inertia about the Y-axis:
Iy=Iy1+Iy2=mR24+mR24=mR22
Step 4: Moment of Inertia about the Z-axis (I_z)
1. For the first disc (in the XY plane):
- The Z-axis is in the plane of the first disc:
Iz1=mR24
2. For the second disc (in the YZ plane):
- The Z-axis is perpendicular to the plane of the second disc:
Iz2=mR22
3. Total moment of inertia about the Z-axis:
Iz=Iz1+Iz2=mR24+mR22=mR24+2mR24=3mR24
Summary of Results
- Moment of inertia about the X-axis:
Ix=3mR24
- Moment of inertia about the Y-axis:
Iy=mR22
- Moment of inertia about the Z-axis:
Iz=3mR24
We have two semicircular discs:
- The first semicircular disc is in the XY plane (horizontal).
- The second semicircular disc is in the YZ plane (vertical).
Both discs have a common diameter along the Y-axis.
1. For the first disc (in the XY plane):
- The moment of inertia of a semicircular disc about an axis perpendicular to its plane and passing through its center is given by:
Ix1=mR22
- The moment of inertia about the X-axis can be found using the perpendicular axis theorem. The moment of inertia about the Z-axis (perpendicular to the plane) is:
Iz2=mR22
- Using the perpendicular axis theorem:
Iz2=Iy2+Ix2⇒Ix2+Iy2=mR22
- Since Ix2=Iy2 (both axes are along the diameter of the disc):
2Ix2=mR22⇒Ix2=mR24
Ix=Ix1+Ix2=mR22+mR24=3mR24
1. For the first disc (in the XY plane):
- The Y-axis is in the plane of the first disc, so:
Iy1=mR24
- The Y-axis is also in the plane of the second disc, so:
Iy2=mR24
Iy=Iy1+Iy2=mR24+mR24=mR22
1. For the first disc (in the XY plane):
- The Z-axis is in the plane of the first disc:
Iz1=mR24
- The Z-axis is perpendicular to the plane of the second disc:
Iz2=mR22
Iz=Iz1+Iz2=mR24+mR22=mR24+2mR24=3mR24
- Moment of inertia about the X-axis:
Ix=3mR24
- Moment of inertia about the Y-axis:
Iy=mR22
- Moment of inertia about the Z-axis:
Iz=3mR24
Topper's Solved these Questions
- BOOK - PHYSICS GALAXY - ASHISH ARORACHAPTER - RIGID BODIES AND ROTATIONAL MOTIONEXERCISE - Unsolved Numerical63videos
RIGID BODIES AND ROTATIONAL MOTION
- BOOK - PHYSICS GALAXY - ASHISH ARORACHAPTER - RIGID BODIES AND ROTATIONAL MOTIONEXERCISE - paragraph24videos
RIGID BODIES AND ROTATIONAL MOTION
- BOOK - PHYSICS GALAXY - ASHISH ARORACHAPTER - NEWTONS LAWS OF MOTIONEXERCISE - Unsolved Numerical Problem81videos
NEWTONS LAWS OF MOTION
- BOOK - PHYSICS GALAXY - ASHISH ARORACHAPTER - WORK, ENERGY AND POWEREXERCISE - unsolved1videos
WORK, ENERGY AND POWER
Similar Questions
Thee spheres each of mass M and radius R are placed in contact with each other as shown in the figure here. Then the moment of inertia of the system about the axis CC' is
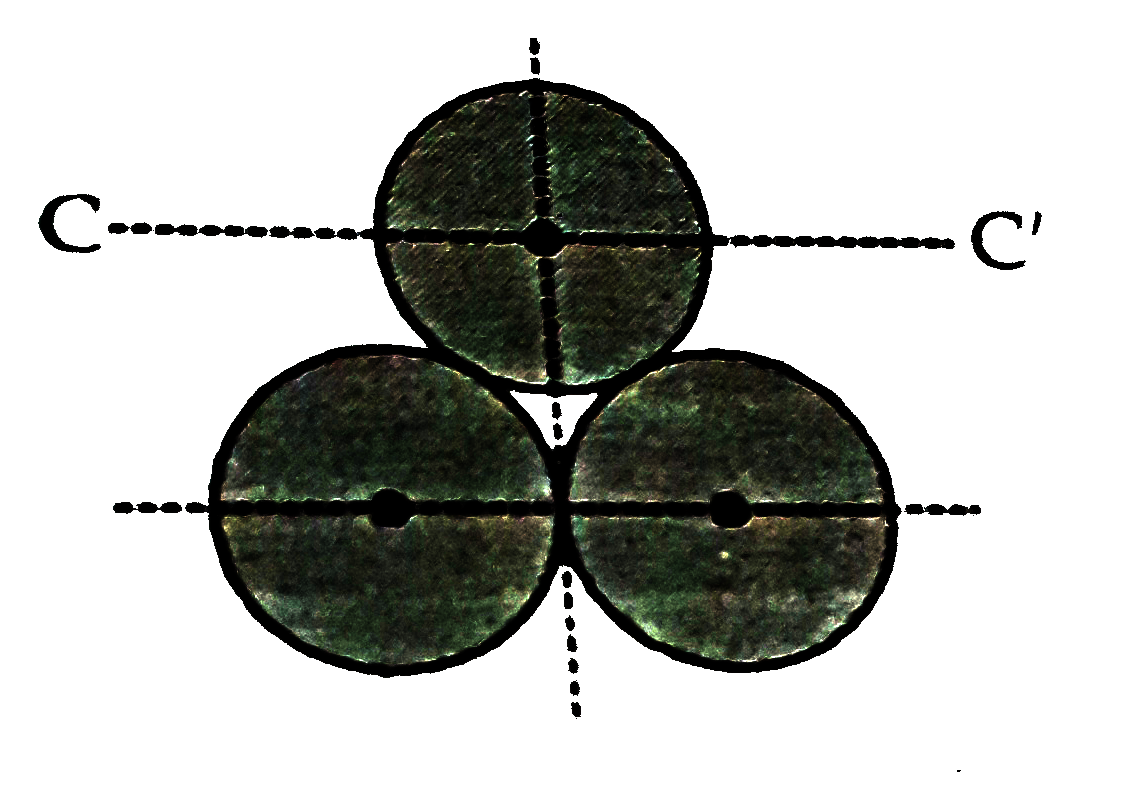 View Solution
View SolutionMoment of inertia of a disc about its own axis is I. Its moment of inertia about a tangential axis in its plane is
View Solution
Knowledge Check
- Question 1 - Select One
Three point masses each of mass m are placed at the corners of an equilateral triangle of side 'a'. Then the moment of inertia of this system about an axis passing along one side of the triangle is
Ama2B3ma2C34ma2D23ma2 - Question 2 - Select One
Particles each of mass 1kg are placed at 1m, 2m and 4m on X-axis with respect to origin. Then moment of inertia of the system about Y-axis is
A7kgm2B14kgm2C21kgm2D28kgm2 - Question 3 - Select One
Two identical rings each of mass m with their planes mutually perpendicular, radius R are welded at their point of contact O. If the system is free to rotate about an axis passing through the point P perpendicular to the plane of the paper, then the moment of in inertia of the system about this axis is equal to
 A6.5mR2B12mR2C6mR2D11.5mR2
A6.5mR2B12mR2C6mR2D11.5mR2
Three identical solid discs, each of mass M and radius R, are arranged as shown in figure. The moment of inertia of the system about an axis AB will be
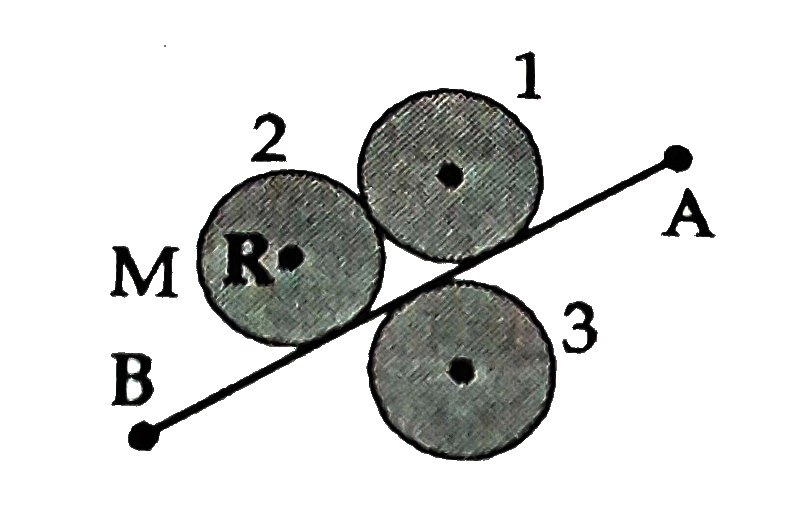 View Solution
View SolutionTwo identical rings each of mass m with their planes mutually perpendicular, radius R are welded at their point of contact O. If the system is free to rotate about an axis passing through the point P perpendicular to the plane to the paper, the moment of inertia of the system about this axis is equal to
 View Solution
View SolutionFour spheres each having mass m and radius r are placed with their centres on the four comers of a square of side a. Then the moment of inertia of the system about an axis along one of the sides of the square, is
View SolutionThe diagram shows a uniform disc of mass M & radius 'a', if the moment of inertia of the disc about the axis XY is I, its moment of inertia about an axis through O and perpendicular to the plane of the disc is
 View Solution
View SolutionTwo identical concentric rings each of mass m and radius R are placed perpendicularly. What is the moment of inertia of the system about the axis of one of the rings ?
View Solution

