In the previous example, calculate the magnitude of the tension in the cord.
Since the magnitude of a has been determined, we can use either of the two original equations to calculate FT. Using equation (2), Mg−FT=Ma (because it's simpler), we find
FT=Mg−Ma=Mg−M.34g=14Mg=14(10)(10)=25N
As you can see, we would have found the same answer if we'd used equation (1) :
FT−Ff=ma⇒FT=Ff+ma=μmg+ma=μmg+m.34g=mg(μ+34)=(2)10(0.5+0.75)=25N
Topper's Solved these Questions
Similar Questions
Knowledge Check
In the previous problem 3 the magnitude of the momentum transferred during the hit is .
AzeroB0.75kgms−1C1.5kgms−1D14kgms−1In the previous problem 3 the magnitude of the momentum transferred during the hit is .
AzeroB0.75kgms−1C1.5kgms−1D14kgms−1A ball is held at rest in position A by two light cords. The horizontal cord is now cut and the ball swings to the position B. What is the ratio of the tension in the cord in position B to that in position A?
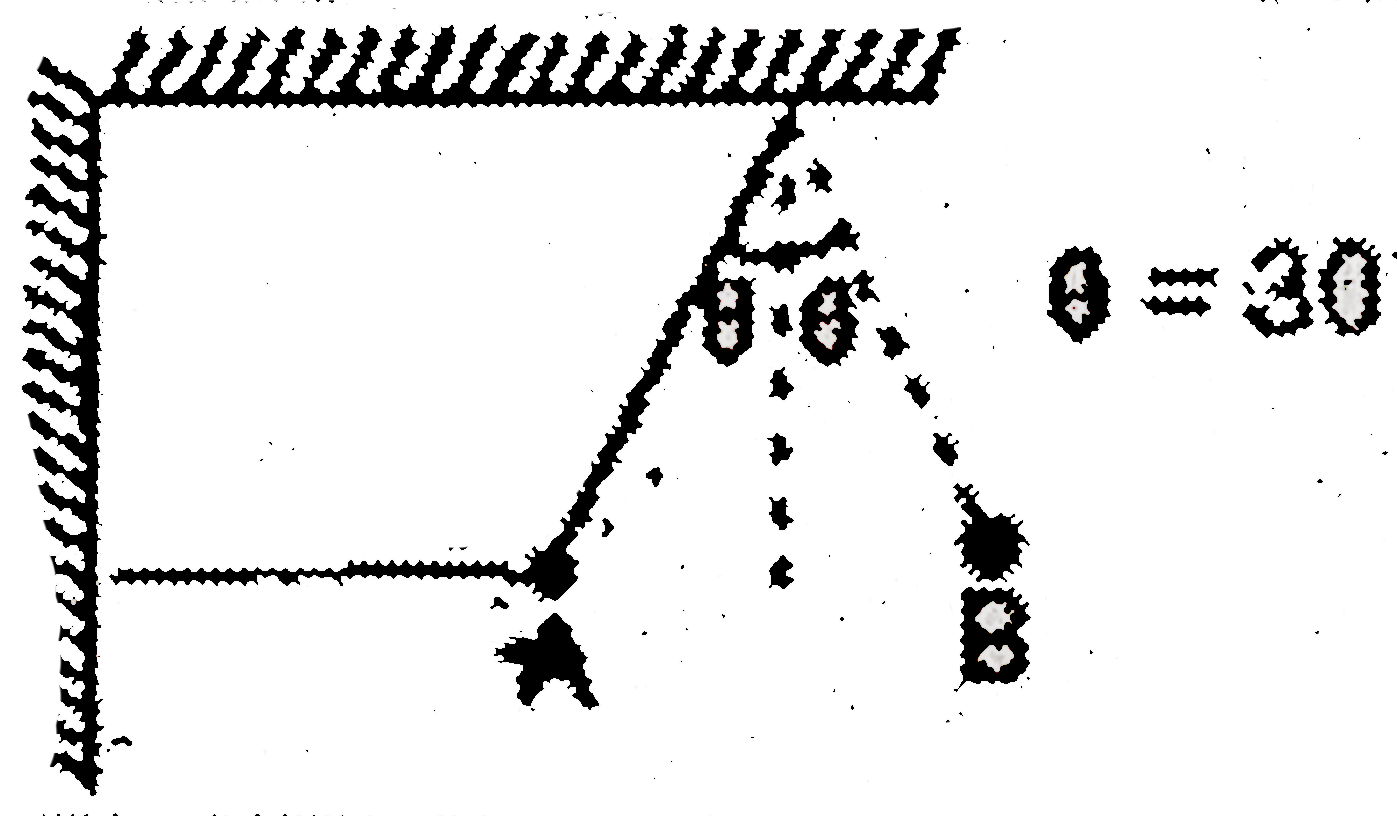 A3/4B1/2C3D1
A3/4B1/2C3D1
Using two light cords a bob is held in equilibrium at A. The horizontal cord is cut and the bob is allowed to swing as a pendulum. Calculate the ratio of the tension in the supporting cord in position B to that in position A.
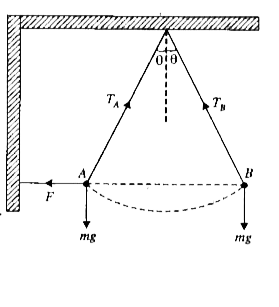
View SolutionIn the previous problem 3 the magnitude of the momentum transferred during the hit is .
View SolutionA small mass m and its supporting wire because a simple pendulum when the horizontal cord is cut . Find the ratio of the tension in the supporting wire immediately after the cord is cut to the tension in the wire before the cord is cut
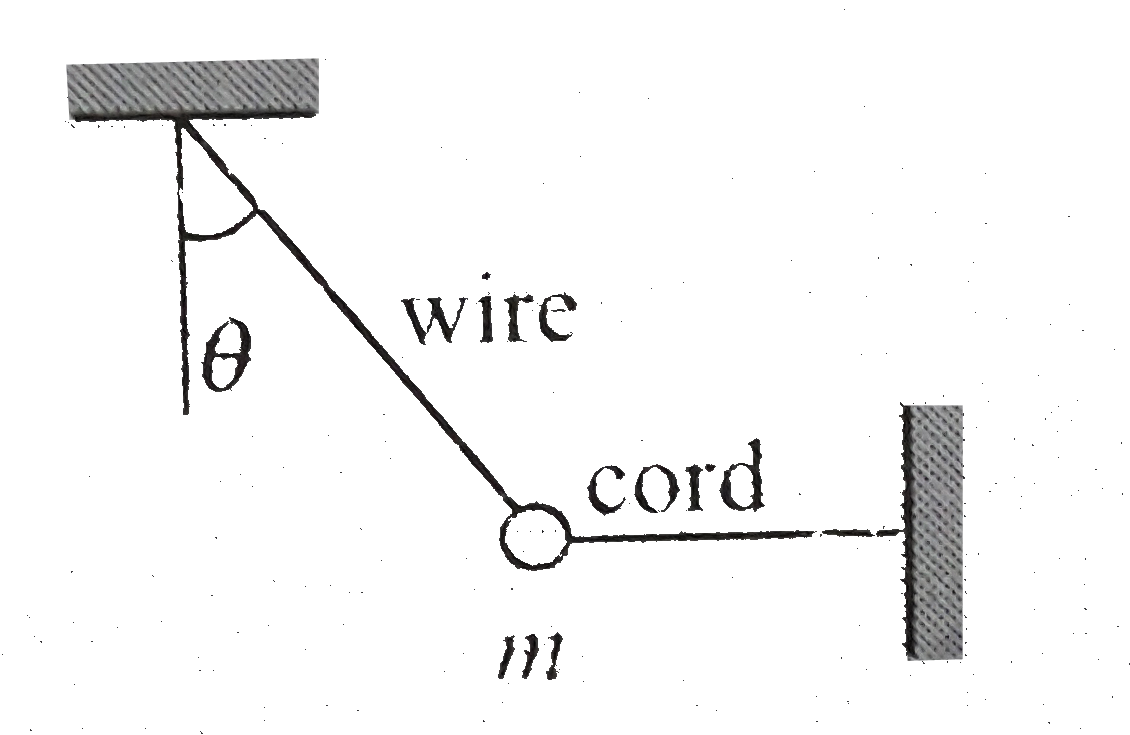
View SolutionA small mass m and its supporting wire because a simple pendulum when the horizontal cord is cut . Find the ratio of the tension in the supporting wire immediately after the cord is cut to the tension in the wire before the cord is cut

View SolutionA ball is held at rest in position A by two light cords. The horizontal cord is now cut and the ball swings to the position B. What is the ratio of the tension in the cord in position B to that in position A?
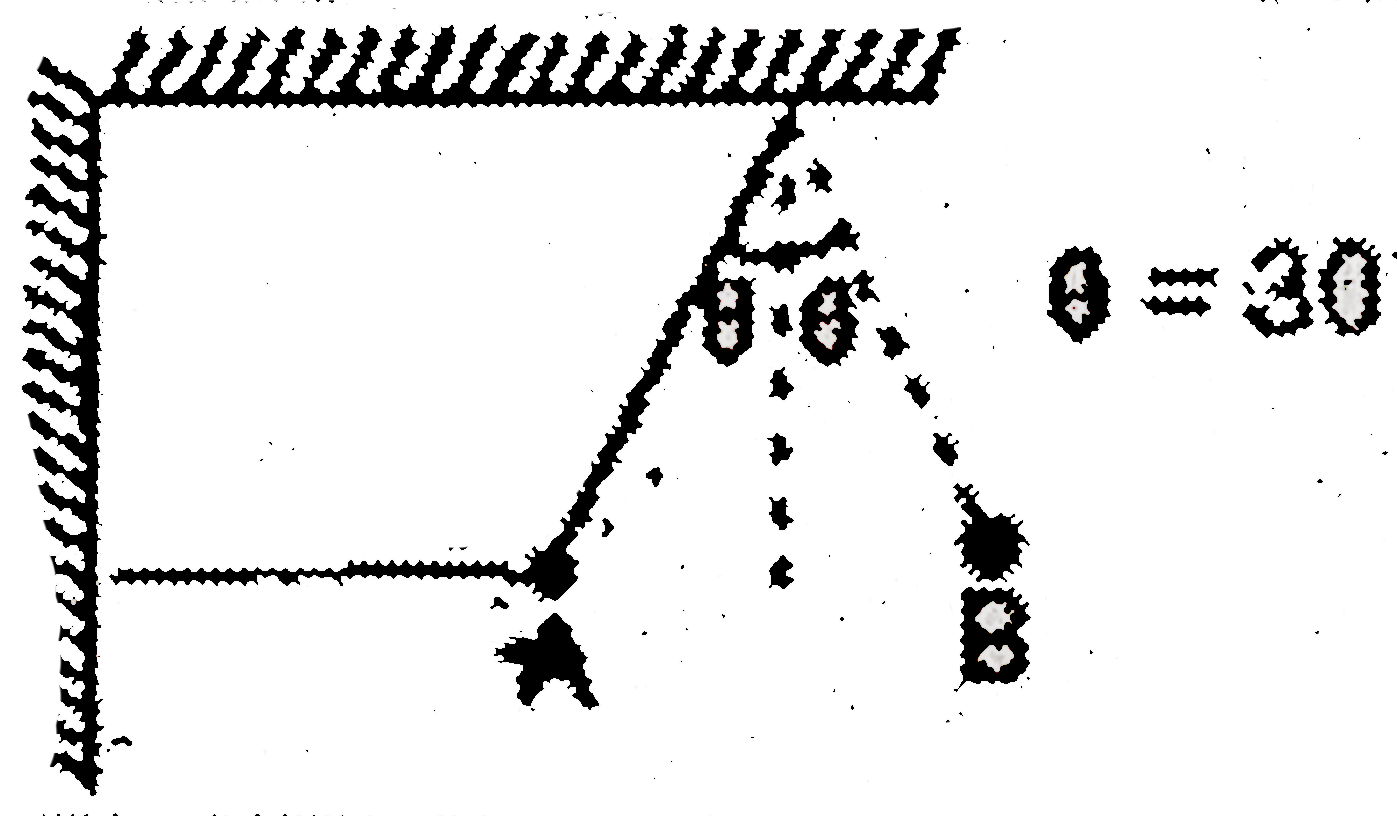
View Solution
