Two masses of mass m each are fixed at a separation distance of 2d. A small mass ms placed midway, when displaced slightly, starts oscillating. Then :
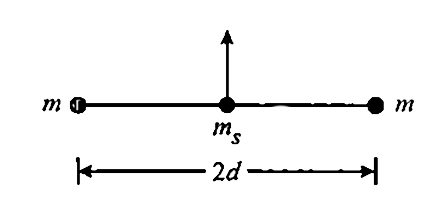
Frequency of simple harmonic motion is given by 12π√4Gmd3
Frequency of simple harmonic motion is given by 12π√2Gmd3
Accleration of the mass ms, is given by Gmd2
Time period of vibration is 2π√GM(2d)3
The correct Answer is:B
Step by step video, text & image solution for Two masses of mass m each are fixed at a separation distance of 2d. A small mass m_(s) placed midway, when displaced slightly, starts oscillating. Then : by Physics experts to help you in doubts & scoring excellent marks in Class 11 exams.
Topper's Solved these Questions
- BOOK - PHYSICS GALAXY - ASHISH ARORACHAPTER - GRAVITATIONEXERCISE - Advance MCQs17videos
GRAVITATION
- BOOK - PHYSICS GALAXY - ASHISH ARORACHAPTER - GRAVITATIONEXERCISE - Unsolved Numerical94videos
GRAVITATION
- BOOK - PHYSICS GALAXY - ASHISH ARORACHAPTER - GRAVITATIONEXERCISE - Concetual MCQ39videos
GRAVITATION
- BOOK - PHYSICS GALAXY - ASHISH ARORACHAPTER - FLUID MECHANICSEXERCISE - Unsolved Numerical Problems86videos
FLUID MECHANICS
- BOOK - PHYSICS GALAXY - ASHISH ARORACHAPTER - KINEMATICSEXERCISE - Unsolved Numerical Pro.83videos
KINEMATICS
Similar Questions
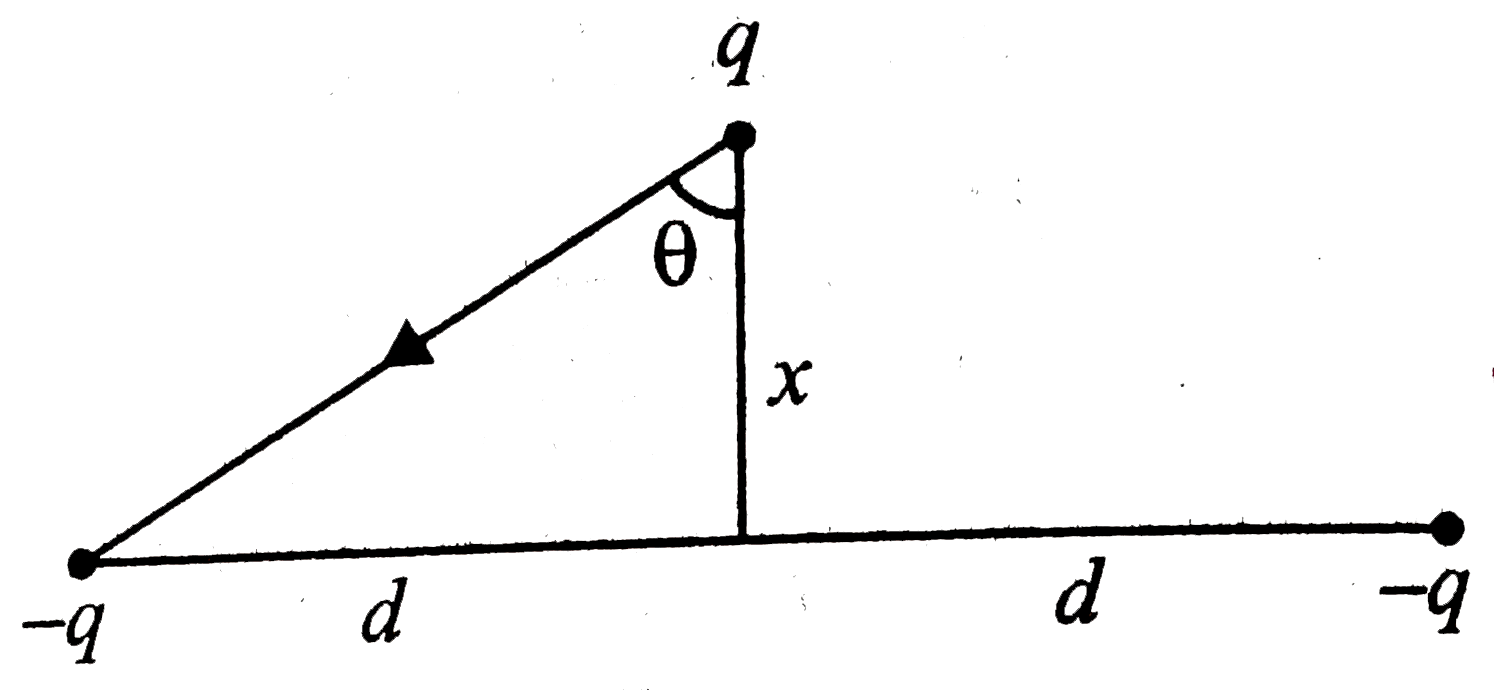
Two charges −q each are fixed separated by distance 2d. A third charge q of mass m placed at the mid-point is displaced at the mid-point is placed slightly by x(x<<d) perpendicular to the line joining the two fixed charges as shown in Fig. Show that q will perform simple harmonic oscillarion of time period.
T=[8π3∈0md3q2]1/2
 View Solution
View SolutionTwo point masses, each equal to M, are placed a distance 2a apart. Show that a small mass m placed midway between them on the line joining them will be in equilibrium and if it is slightly displaced from this position along the line perpendicular to the line joining the masses, it will execute simple harmonic oscillations. Calculate the frequnency of these oscillations.
View Solution
Knowledge Check
- Question 1 - Select One
Two electrons each are fixed at a distance '2d'. A third charge proton placed at the midpoint is displaced slightly by a distance x(x<<d) perpendicular to the line joining the two fixed charges. Proton will execute simple harmonic motion having angular frequency : (m = mass of charged particle)
A(2q2πε0md3)12B(πε0md32q2)12C(q22πε0md3)12D(2πε0md3q2)12 - Question 2 - Select One
The frequency of the mass when it is displaced slightly is
 Aυ=12π√k1k2(k1+k2)mBυ=12π√k1+k2mCυ=12π√k1k2mDυ=12π√k1+k2k1k2m
Aυ=12π√k1k2(k1+k2)mBυ=12π√k1+k2mCυ=12π√k1k2mDυ=12π√k1+k2k1k2m - Question 3 - Select One
Two bodies, each of mass M, are kept fixed with a separation 2L. A particle of mass m is projected from the midpoint of the line joining their centres, perpendicular to the line. The gravitational constant is G. The correct statement (s) is (are)
AThe minimum initial velocity of the mass m to escape the gravitational field of the two bodies in 4√GMLBThe minimum initial velocity of the mass m to escape the gravitational field of the two bodies is 2√GMLCThe minimum initial velocity of the mass m t eacape the gravitational field of the two bodies is √2GMLDThe energy of the mass m remains constant
We know that when two equal masses are placed on a line then, gravitational force on a third mass is zero due to these two masses when the third is placed at the centre of line joining the two masses. But if third mass is placed anywhere else on the x-axis, the gravitational force acts on that mass due to these two masses. Consider a system shown in figure.
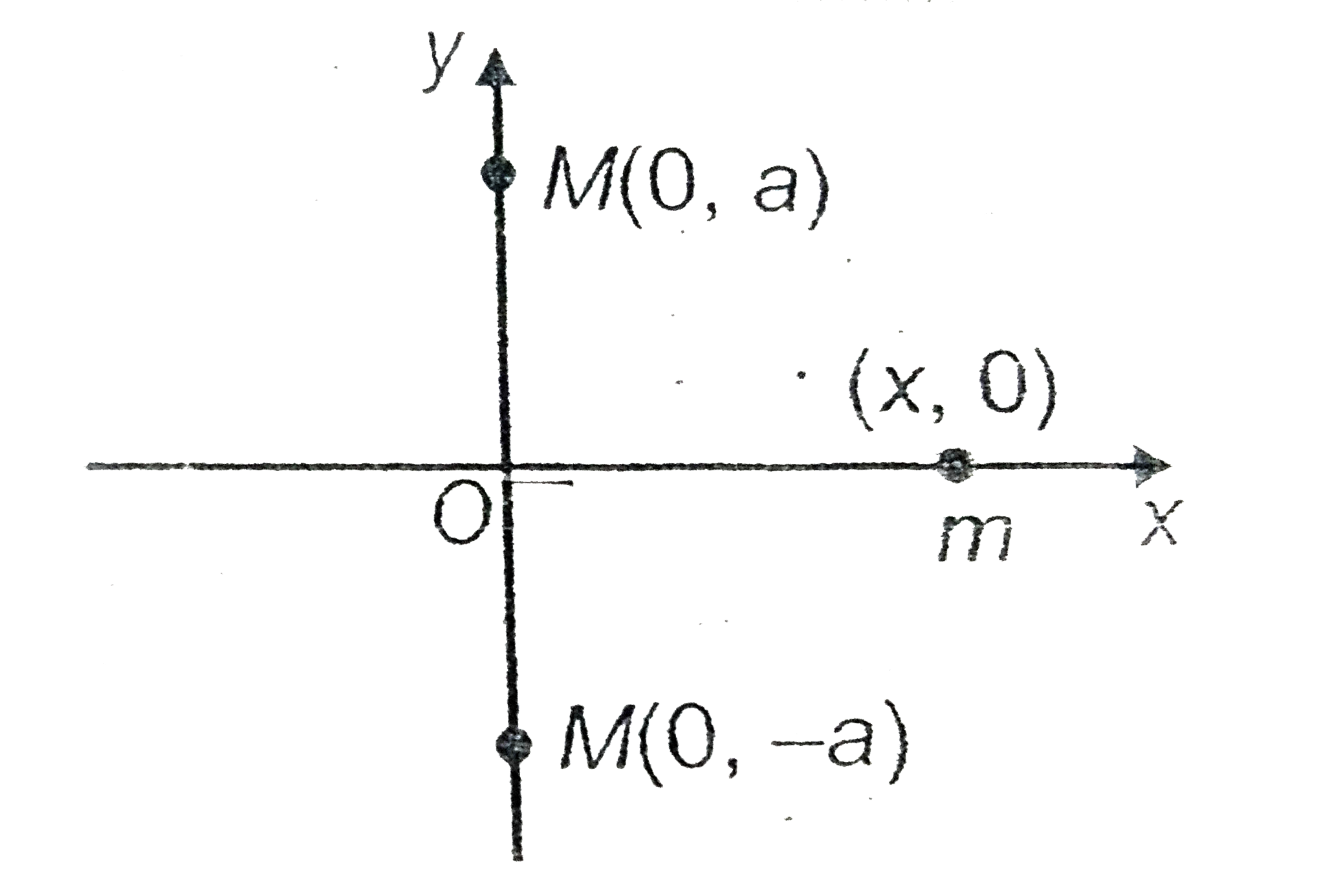
In this system two point masses of mass M each are placed at y-axis at a distance a from origin and these masses are fixed. If a third point mass of mass m is released at a distance x from origin on x-axis, then answer the following questions.
The distance between positions of maximum force on mass m isView SolutionWe know that when two equal masses are placed on a line then, gravitational force on a third mass is zero due to these two masses when the third is placed at the centre of line joining the two masses. But if third mass is placed anywhere else on the x-axis, the gravitational force acts on that mass due to these two masses. Consider a system shown in figure.
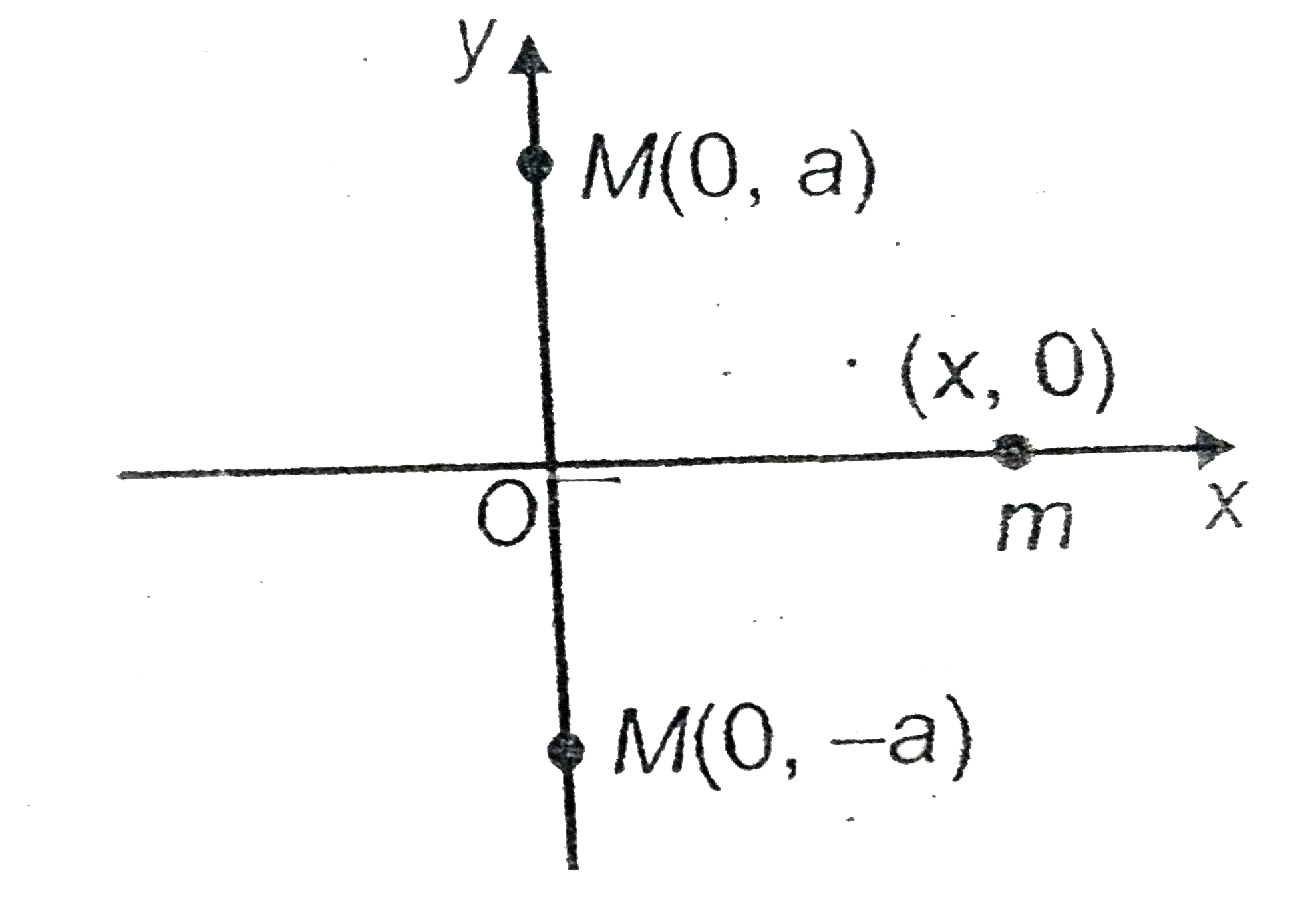
In this system two point masses of mass M each are placed at y-axis at a distance a from origin and these masses are fixed. If a third point mass of mass m is released at a distance x from origin on x-axis, then answer the following questions.
Which of the following graphs best represents the variation of force on mass m with distance x ?View SolutionWe know that when two equal masses are placed on a line then, gravitational force on a third mass is zero due to these two masses when the third is placed at the centre of line joining the two masses. But if third mass is placed anywhere else on the x-axis, the gravitational force acts on that mass due to these two masses. Consider a system shown in figure.
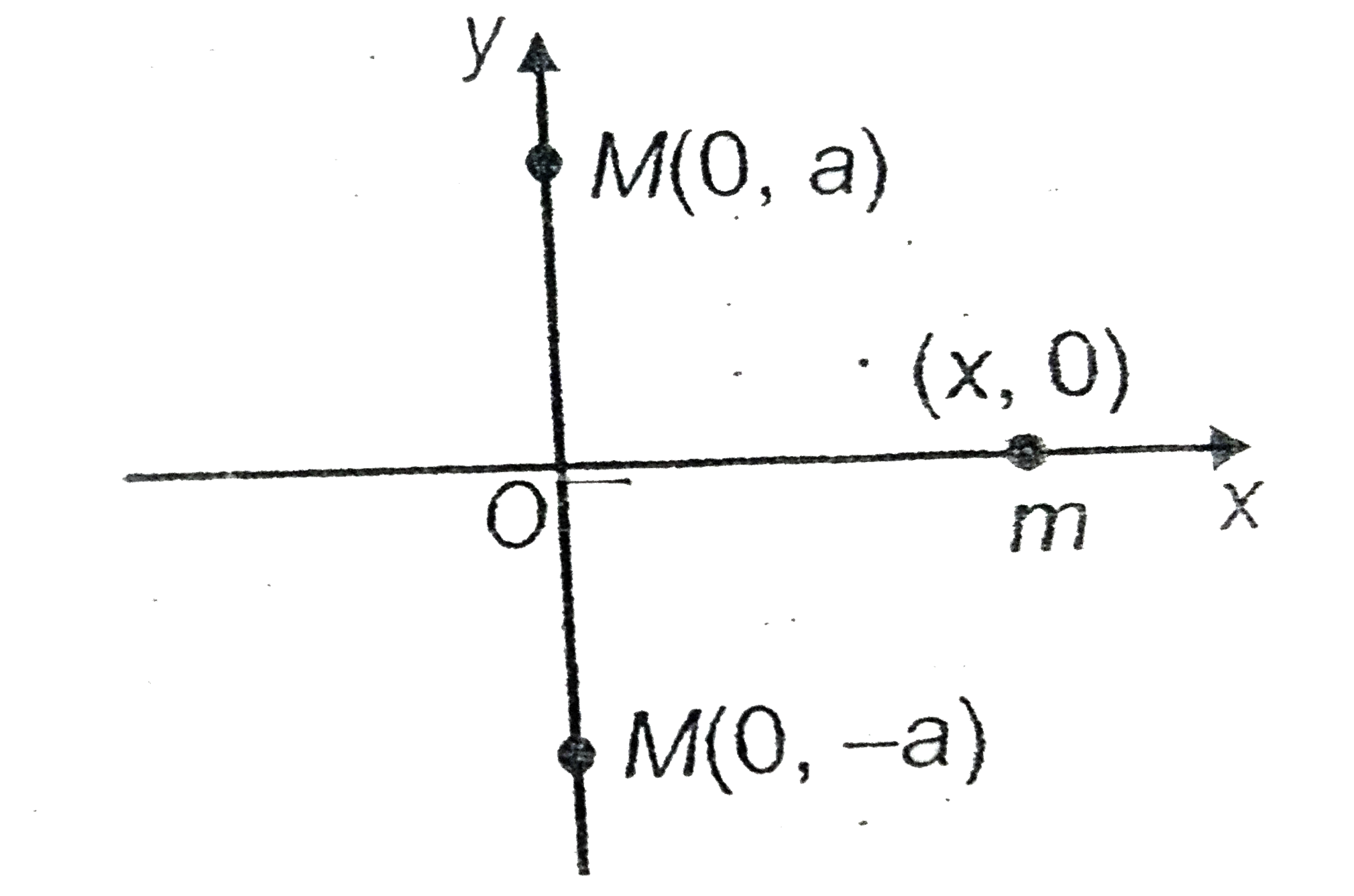
In this system two point masses of mass M each are placed at y-axis at a distance a from origin and these masses are fixed. If a third point mass of mass m is released at a distance x from origin on x-axis, then answer the following questions.
If a gt gt x, then which of the following statements is correct about the motion of mass m ?View SolutionWe know that when two equal masses are placed on a line then, gravitational force on a third mass is zero due to these two masses when the third is placed at the centre of line joining the two masses. But if third mass is placed anywhere else on the x-axis, the gravitational force acts on that mass due to these two masses. Consider a system shown in figure.
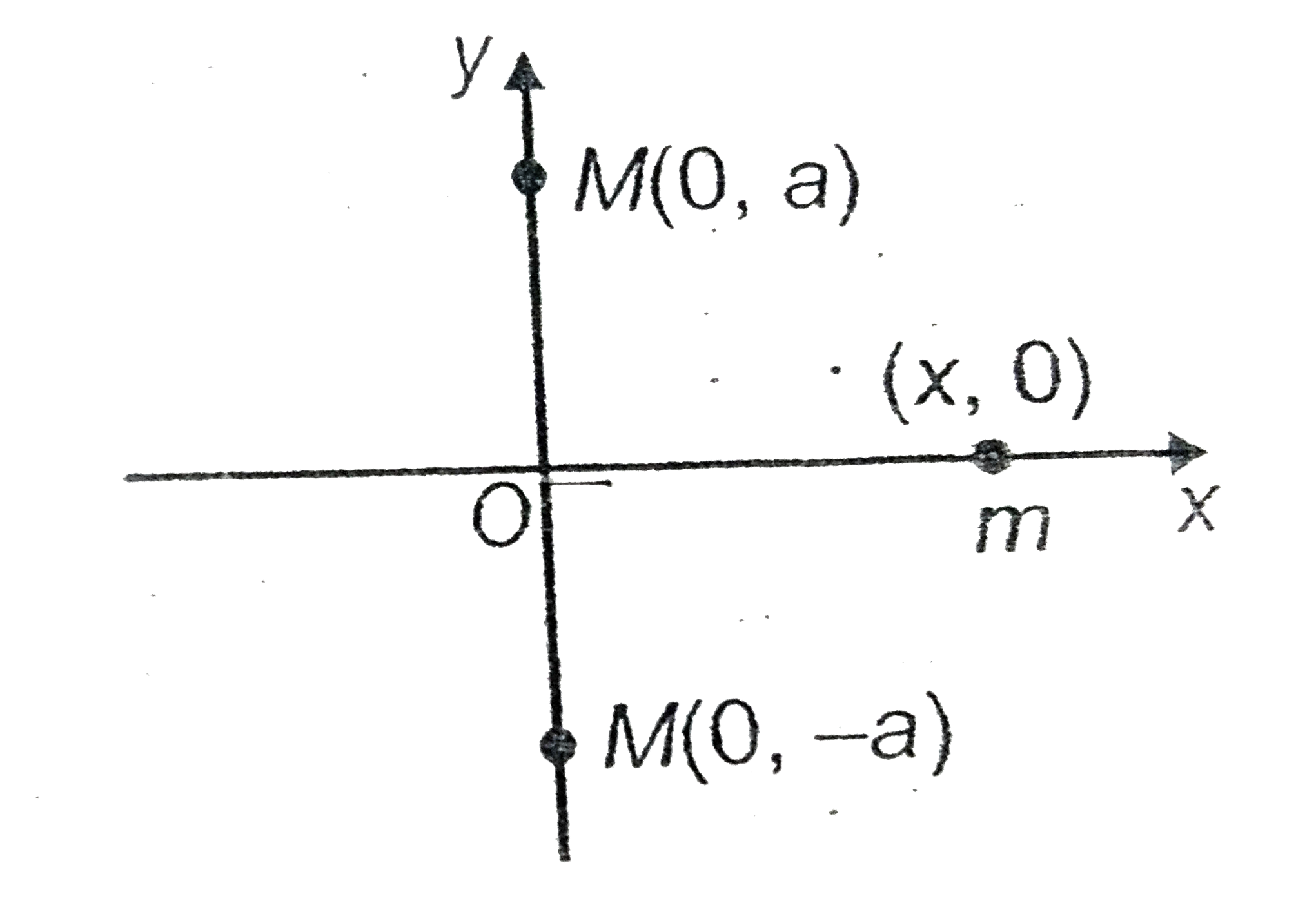
In this system two point masses of mass M each are placed at y-axis at a distance a from origin and these masses are fixed. If a third point mass of mass m is released at a distance x from origin on x-axis, then answer the following questions.
The net force on the mass m is
when →x is position vector of the point mass of mass m.View SolutionTwo spheres of masses mand2m are separated by distance d. A particle of mass m5 is projected straight from 2m towards m with a velocity v0. Which of the following statements is correct ?
View Solution

