Question

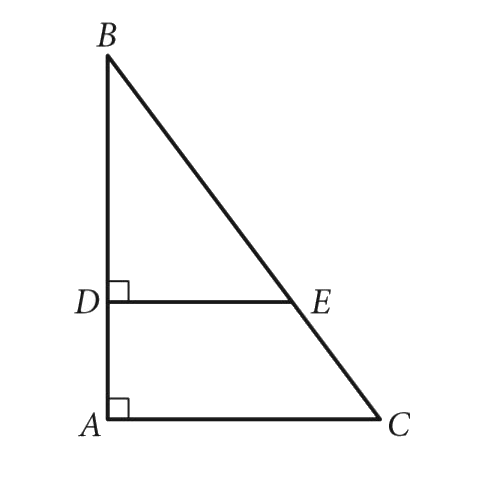
In the figure above , tan $B=\frac{3}{4}$. If BC =15 and DA=4, what is the length of $\overline{DE}$ ?

Explanation

Since tan B = `3/4` , `triangleABC` and `triangleDBE` are both 3-4-5 triangles. This means that they are both similar to the right triangle with sides of lengths 3, 4, and 5. Since BC = 15, which is 3 times as long as the hypotenuse of the 3-4-5 triangle, the similarity ratio of `triangleABC` to the 3-4-5 triangle is 3:1. Therefore, the length of `bar(AC)` (the side opposite to B) is 3 × 3 = 9, and the length of `bar(AB)` (the side adjacent to angle B) is 4 × 3 = 12. It is also given that DA = 4. Since AB = DA + DB and AB = 12, it follows that DB = 8, which means that the similarity ratio of `triangleDBE` to the 3-4-5 triangle is 2:1 ( `bar(DB)` is the side adjacent to angle B). Therefore, the length of `bar(DE)` , which is the side opposite to angle B, is 3 × 2 = 6.