Question

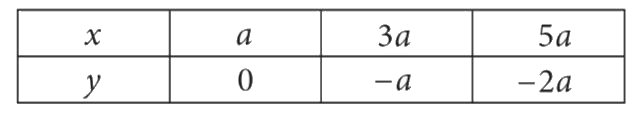
Some values of x and their corresponding values of y are shown in the table above, where a is a constant. If there is a linear relationship between x and y, which of the following equations represents the relationship?

Options

A. x + 2y = a
B. x + 2y = 5a
C. 2x − y = −5a
D. 2x − y = 7a

Answer

A

Explanation

The slope-intercept form of a linear equation in the xy-plane is y = mx + b, where m is the slope of the graph of the equation and b is the y-coordinate of the y-intercept of the graph. Any two ordered pairs `(x_1, y_1)` and `(x_2, y_2)` that satisfy a linear equation can be used to compute the slope of the graph of the equation using the formula m =`(y_2-y_1)/(x_2-x_1)` . Substituting the two pairs (a, 0) and (3a, −a) from the table into the formula gives `m=(-a-0)/(3a-a)`, which can be rewritten as `(-a)/(2a)`, or `-1/2` . Substituting t his value for m in the slope-intercept form of the equation produces `y=-1/2x+b`. Substituting values from the ordered pair (a, 0) in the table into this equation produces `0=-1/2(a)+b`, which simplifies to `b=a/2`. Substituting t his value for b in the slopeintercept form of the equation produces `y=-1/2x+a/2`. Rewriting this equation in standard form by adding `1/2x` to both sides and then multiplying both sides by 2 gives the equation x + 2y = a.
Choice B is incorrect and may result from a calculation error when determining the y-intercept of the graph of the equation. Choices C and D are incorrect and may result from an error in calculation when determining the slope of the graph of the equation.