A circular ring of mass 6kg and radius a is placed such that its center of lies at the origin. Two particles of masses 2 kg each are placed at the intersecting points of the circle with positive X-axis and positive Y-axis. Then, the angle made by the position vector of center of mass of entire system with X-axis is
45∘
60∘
tan−(45)
30∘
The correct Answer is:A
P is the position of center of mass of particle at 2 and 3. Q is position of center of mass of all three particles.

tanθ=yCMxCM=(m1y1+m2y2+m3y3m1x1+m2y2+m3y3
6×0+2×0+2×a6×0+2×a+2×0=1
or θ=45∘
Topper's Solved these Questions
- BOOK - DC PANDEYCHAPTER - CENTRE OF MASSEXERCISE - Assertion and reason21videos
CENTRE OF MASS
- BOOK - DC PANDEYCHAPTER - CENTRE OF MASSEXERCISE - Match the coloumns9videos
CENTRE OF MASS
- BOOK - DC PANDEYCHAPTER - CENTRE OF MASSEXERCISE - Check points50videos
CENTRE OF MASS
- BOOK - DC PANDEYCHAPTER - CALORIMETRY AND HEAT TRANSFEREXERCISE - Medical entrance s gallery38videos
CALORIMETRY AND HEAT TRANSFER
- BOOK - DC PANDEYCHAPTER - CENTRE OF MASS, IMPULSE AND MOMENTUMEXERCISE - Comprehension type questions15videos
CENTRE OF MASS, IMPULSE AND MOMENTUM
Similar Questions
A circular ring of mass 8kg and radius 2m is placed such that its centre lies at the origin Two particles of masses 3kg and 4kg are placed at the intersecting points of the circle with +ve x-axis and +ve y-axis respectively. Then the angle made by the position vector of centre of mass of entire system with positive y-axis is?
View SolutionTwo particle of mass 4 kg & 2kg are located as shown in figure then find out the position of centre of mass.
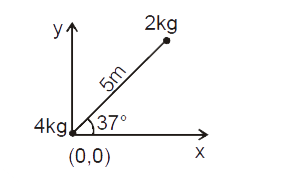 View Solution
View Solution
Knowledge Check
- Question 1 - Select One
Two particles of masses 1kg and 2kg are located at x=0 and x=3m. Find the position of their centre of mass.
A2m from 1 kgB2m from 2 kgC1m from 1 kgDnone of these - Question 2 - Select One
Four particles, each of mass 1kg are placed at the corners of a square OABC of side 1m. O is at the origin of the coordinate system. OA and OC are aligned along positive X-axis and positive Y-axis respectively. The position vector of the centre of mass is (in m)
Aˆi+ˆjB12(ˆi+ˆj)C(ˆi−ˆj)D12(ˆi−ˆj) - Question 3 - Select One
Three particles , each of mass m, are placed at the vertices of a right angled triangle as shown in figure. The position vector of the center of mass of the system is (O is the origin and ˆi,ˆj,ˆk are unit vectors)
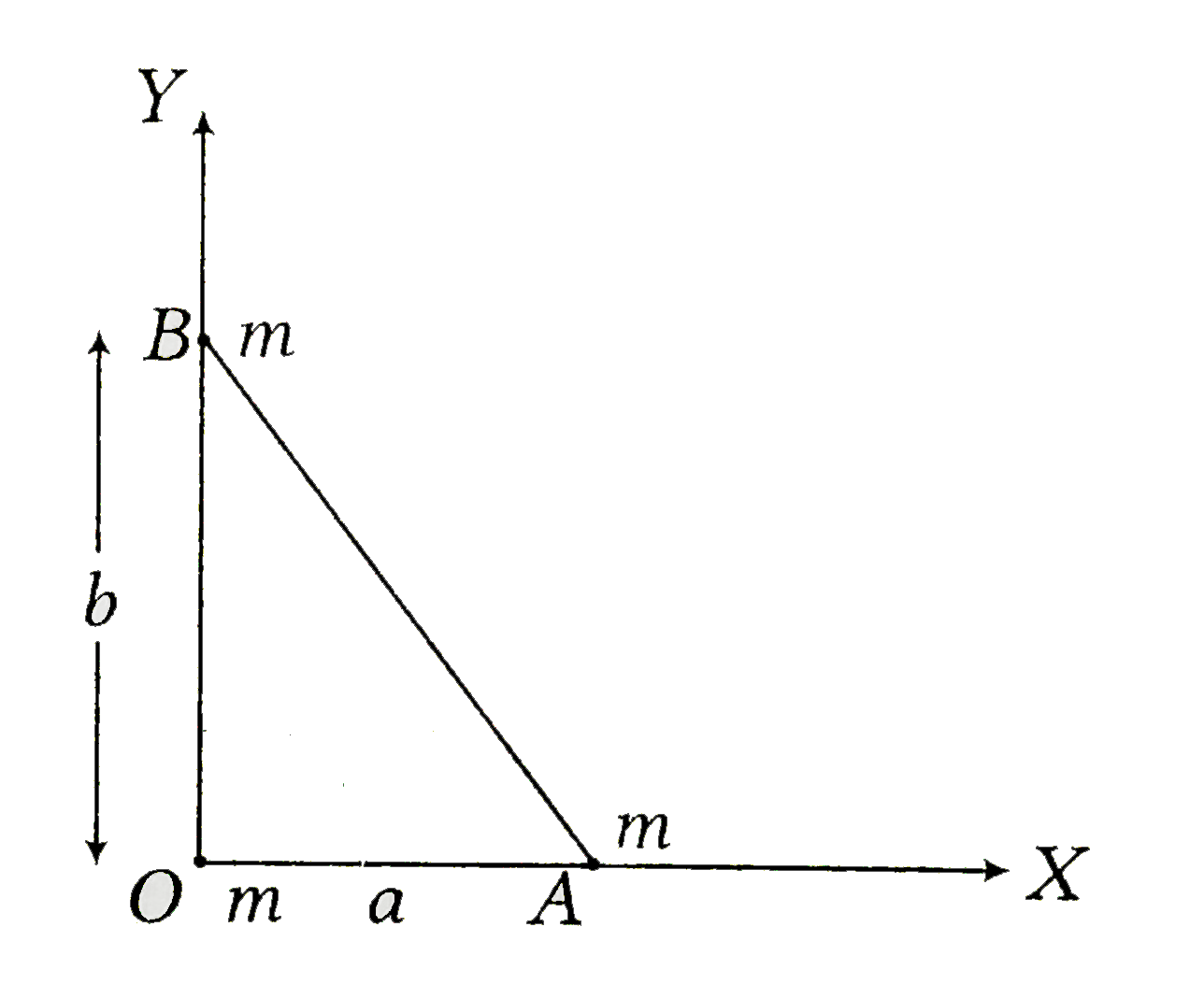 A13(aˆi−bˆj)B23(aˆi−bˆj)C23(aˆi+bˆj)D13(aˆi+bˆj)
A13(aˆi−bˆj)B23(aˆi−bˆj)C23(aˆi+bˆj)D13(aˆi+bˆj)
Two particle of mass 1 kg and 2 kg are located at x = 0 and x = 3 m. Find the position of their centre of mass.
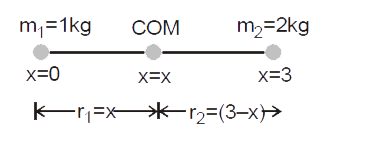 View Solution
View SolutionIf a line makes an angle π4 with the positive directions of each of X-axis and Y-axis, then the angle that the line makes with the positive direction of the Z-axis is
View SolutionIf a line makes an angle π4 with the positive directions of each of X-axis and Y-axis, then the angle that the line makes with the positive direction of the Z-axis is
View SolutionA circular ring of mass M and radius 'a' is placed in a gravity free space. A small particle of mass m placed on the axis of the ring at a distance √3a from the center of the ring, is released from rest. The velocity with which the particle passes through the center of the ring is
View SolutionThree rings each of mass m and radius r are so placed that they touch each other. The radius of gyration of the system about the axis as shown in the figure is
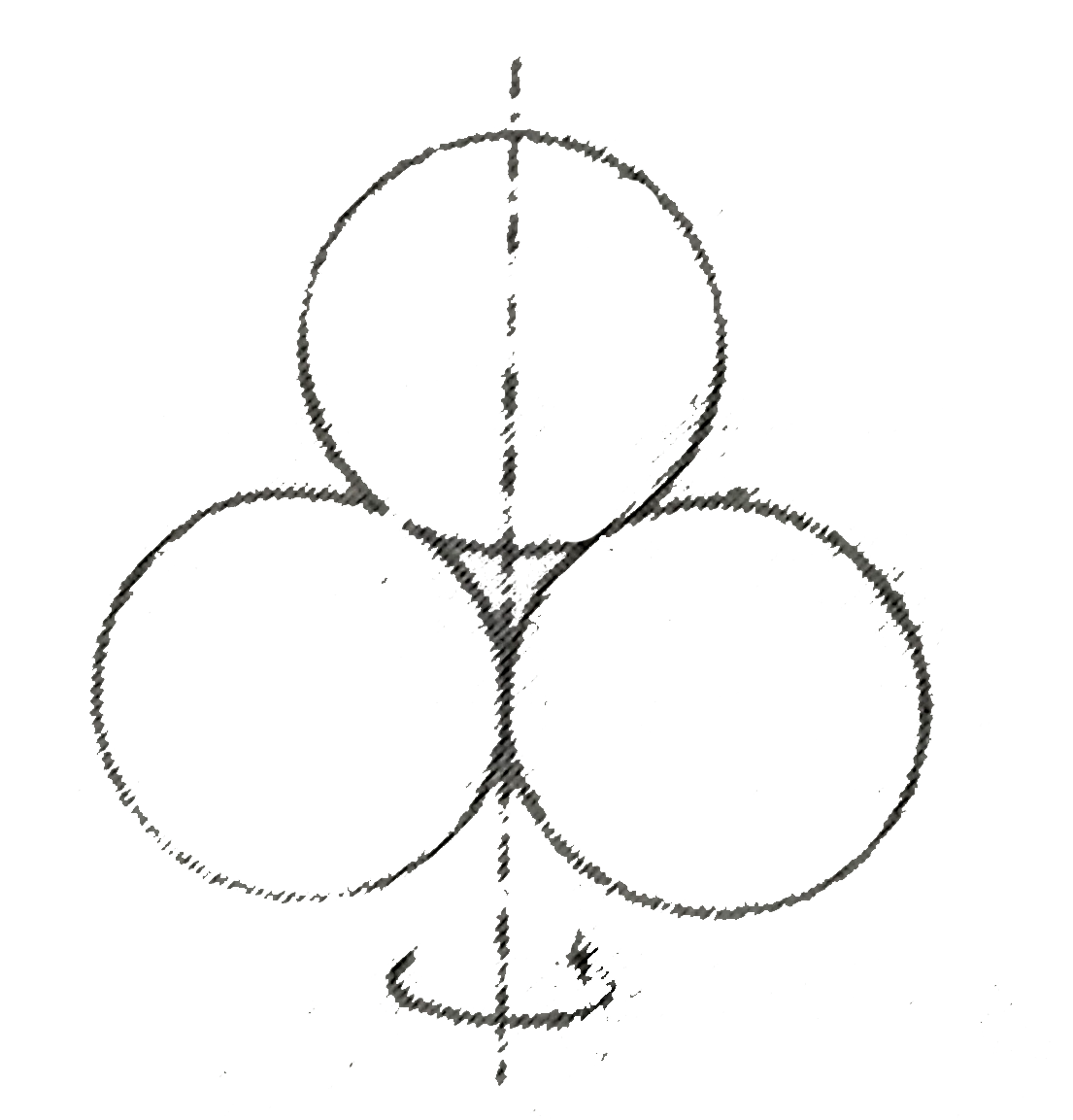 View Solution
View Solution

