A ball is released from the top of a tower. The ratio of work done by force of gravity in 1st second, 2nd second and 3rd second of the motion of ball is
1:2:3
1:4:16
1:3:5
1:9:25
The correct Answer is:C
To solve the problem of finding the ratio of work done by the force of gravity in the 1st, 2nd, and 3rd seconds of the motion of a ball released from the top of a tower, we can follow these steps:
Step 1: Understand the motion of the ball
When the ball is released from rest, it falls freely under the influence of gravity. The distance it travels in each second can be calculated using the equations of motion.
Step 2: Calculate the displacement in each second
The displacement Sn during the n-th second can be calculated using the formula:
Sn=g⋅n−12g
where g is the acceleration due to gravity.
For the 1st second (n=1):
S1=g⋅1−12g=g−12g=g2
For the 2nd second (n=2):
S2=g⋅2−12g=2g−12g=3g2
For the 3rd second (n=3):
S3=g⋅3−12g=3g−12g=5g2
Step 3: Write the displacements
Now we have the displacements for each second:
- S1=g2
- S2=3g2
- S3=5g2
Step 4: Find the ratio of displacements
The ratio of displacements S1:S2:S3 is:
S1:S2:S3=g2:3g2:5g2
Cancelling g2 from each term gives:
1:3:5
Step 5: Calculate the work done by gravity
The work done by gravity in each second can be calculated using the formula:
Work=Force×Displacement
Since the force of gravity (weight of the ball) remains constant, the work done in each second will be directly proportional to the displacement in that second.
Step 6: Write the ratio of work done
Thus, the ratio of work done by gravity in the 1st, 2nd, and 3rd seconds will also be:
Work1:Work2:Work3=1:3:5
Final Answer
The ratio of work done by the force of gravity in the 1st, 2nd, and 3rd seconds of the motion of the ball is:
1:3:5
When the ball is released from rest, it falls freely under the influence of gravity. The distance it travels in each second can be calculated using the equations of motion.
The displacement Sn during the n-th second can be calculated using the formula:
Sn=g⋅n−12g
where g is the acceleration due to gravity.
S1=g⋅1−12g=g−12g=g2
S2=g⋅2−12g=2g−12g=3g2
S3=g⋅3−12g=3g−12g=5g2
Now we have the displacements for each second:
- S1=g2
- S2=3g2
- S3=5g2
The ratio of displacements S1:S2:S3 is:
S1:S2:S3=g2:3g2:5g2
Cancelling g2 from each term gives:
1:3:5
The work done by gravity in each second can be calculated using the formula:
Work=Force×Displacement
Since the force of gravity (weight of the ball) remains constant, the work done in each second will be directly proportional to the displacement in that second.
Thus, the ratio of work done by gravity in the 1st, 2nd, and 3rd seconds will also be:
Work1:Work2:Work3=1:3:5
The ratio of work done by the force of gravity in the 1st, 2nd, and 3rd seconds of the motion of the ball is:
1:3:5
Topper's Solved these Questions
- BOOK - DC PANDEYCHAPTER - WORK, POWER AND ENERGYEXERCISE - A Only One Option is Correct42videos
WORK, POWER AND ENERGY
- BOOK - DC PANDEYCHAPTER - WORK, POWER AND ENERGYEXERCISE - B More than One Option is Correct26videos
WORK, POWER AND ENERGY
- BOOK - DC PANDEYCHAPTER - WORK, ENERGY AND POWEREXERCISE - MEDICAL ENTRACES GALLERY33videos
WORK, ENERGY AND POWER
Similar Questions
A ball is dropped from the top of a tower of herght (h). It covers a destance of h//2 in the last second of its motion. How long does the ball remain in air?
View SolutionA ball is released from the top of a tower to height h. It takes T seconds to reach the ground. What is the position of ball in T/3 seconds?
View Solution
Knowledge Check
- Question 1 - Select One
A ball is dropped from the top of a tower. The ratio of work done by force of gravity in 1st,2nd, and 3rd second of the motion of ball is
A1:2:3B1:4:16C1:3:5D1:9:5 - Question 2 - Select One
A ball is released from the top of a tower of height h metre. It takes T second to reach the ground. What is the position of the ball in T3 second?
Ah9metre from the groundB(7h/9) metre from the groundC(8h/9) metre from the groundD(17h/18) metre from the ground - Question 3 - Select One
A ball is released from the top of a tower of height h metre. It takes T second to reach the ground. What is the position of the ball in T/3 sccond?
Ah/9 metre from the groundB7h/9 metre from the groundC8h/9 metre from the groundD17h/18 metre from the ground.
A ball falls freely from rest. The ratio of the distance travelled in first, second, third and fourth second is
View SolutionA ball is released from the top of a tower of height h metre. It takes T second to reach the ground. What is the position of the ball in T3 second?
View SolutionA ball is dropped from the roof of a tower height h. The total distance covered by it in the last second of its motion is equal to the distance covered by it in first three seconds. The value of h in metre is (g=10m/s2)
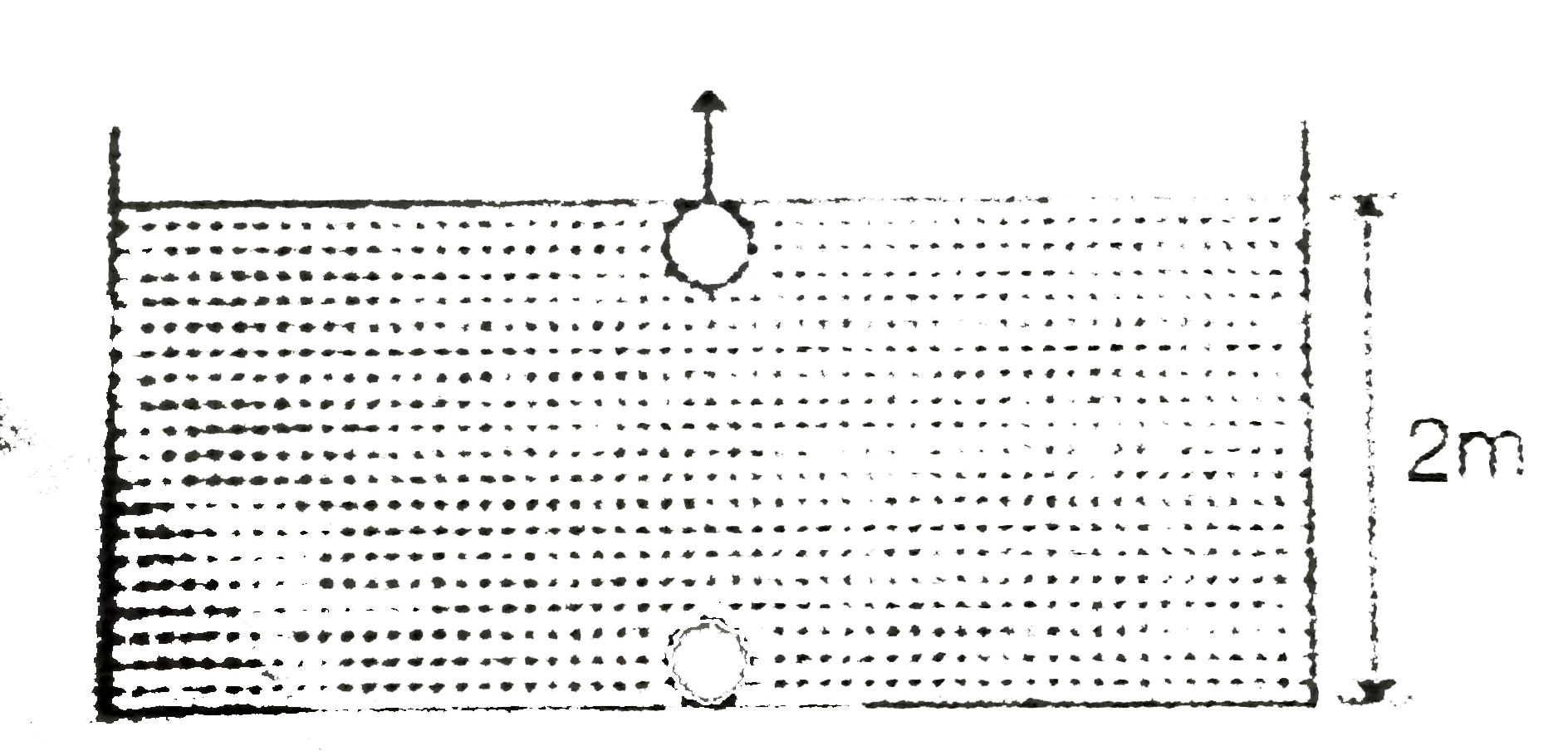
View SolutionA ball is released from the bottom of a tank filled with water up to 2m. On reaching the top its kinetic energy is found to be 16 J. Mass of the ball is 2 kg.
Ignoring the viscosity, let W1 be the work done by upthrust and W2 the work done by gravity, then (g=10m/s2)
 View Solution
View SolutionA ball rleased from the tope of a tower travels 1136 of the height of the tower in the last second of its journey. The height of the tower is (g= 10 ms^(2)).
View Solution

