A tank cotaining water has an orifice 10 m below the surface of water in the tank . If there is no wastage of energy ,find the speed of discharge.
The correct Answer is:14ms−1
To find the speed of discharge of water from an orifice located 10 meters below the surface of water in a tank, we can use the principle of conservation of energy, specifically Bernoulli's equation. Here’s a step-by-step solution:
Step 1: Understand the setup
We have a tank filled with water, and there is an orifice (a small opening) located 10 meters below the surface of the water. We need to find the speed at which water discharges from this orifice.
Step 2: Apply Bernoulli's equation
According to Bernoulli's principle, the total mechanical energy along a streamline is constant if there is no energy loss. The equation can be expressed as:
P+12ρv2+ρgh=constant
Where:
- P = pressure at the point (at the surface of the water)
- ρ = density of the fluid (water)
- v = velocity of the fluid at that point
- g = acceleration due to gravity (approximately 9.81m/s2)
- h = height above a reference point
Step 3: Set up the equation for the two points
1. At the surface of the water (Point 1):
- Pressure P0 (atmospheric pressure)
- Velocity v1=0 (the water is at rest)
- Height h1=10m
2. At the orifice (Point 2):
- Pressure P2=P0 (atmospheric pressure)
- Velocity v2=v (the velocity we want to find)
- Height h2=0m
Step 4: Write Bernoulli's equation for both points
At Point 1:
P0+12ρ(0)2+ρg(10)=P0+ρg(10)
At Point 2:
P0+12ρv2+ρg(0)=P0+12ρv2
Step 5: Equate the two points
Since there is no energy loss, we can equate the total energy at both points:
P0+ρg(10)=P0+12ρv2
Step 6: Simplify the equation
Cancel P0 from both sides:
ρg(10)=12ρv2
Step 7: Cancel ρ from both sides
Assuming ρ is not zero, we can divide both sides by ρ:
g(10)=12v2
Step 8: Solve for v2
Multiply both sides by 2:
20g=v2
Step 9: Substitute the value of g
Using g=9.81m/s2:
v2=20×9.81
Step 10: Calculate v2
v2=196.2
Step 11: Take the square root to find v
v=√196.2≈14.0m/s
Final Answer
The speed of discharge of water from the orifice is approximately 14.0 m/s.
---
We have a tank filled with water, and there is an orifice (a small opening) located 10 meters below the surface of the water. We need to find the speed at which water discharges from this orifice.
According to Bernoulli's principle, the total mechanical energy along a streamline is constant if there is no energy loss. The equation can be expressed as:
- P = pressure at the point (at the surface of the water)
- ρ = density of the fluid (water)
- v = velocity of the fluid at that point
- g = acceleration due to gravity (approximately 9.81m/s2)
- h = height above a reference point
1. At the surface of the water (Point 1):
- Pressure P0 (atmospheric pressure)
- Velocity v1=0 (the water is at rest)
- Height h1=10m
- Pressure P2=P0 (atmospheric pressure)
- Velocity v2=v (the velocity we want to find)
- Height h2=0m
At Point 1:
P0+12ρ(0)2+ρg(10)=P0+ρg(10)
P0+12ρv2+ρg(0)=P0+12ρv2
Since there is no energy loss, we can equate the total energy at both points:
Cancel P0 from both sides:
Assuming ρ is not zero, we can divide both sides by ρ:
Multiply both sides by 2:
Using g=9.81m/s2:
v2=196.2
v=√196.2≈14.0m/s
The speed of discharge of water from the orifice is approximately 14.0 m/s.
Topper's Solved these Questions
Similar Questions
A tank containing water has an orifice 2 m below the surface of water. If there is no wastage of energy. Find the speed of discharge.
View SolutionA water tank is placed on a platform of height 5 m high and there is an orifice near the bottom in the wall of tank at 5 m below the level of water. Find the speed with which water will hit the ground [Take g = 10 m/s2 ]
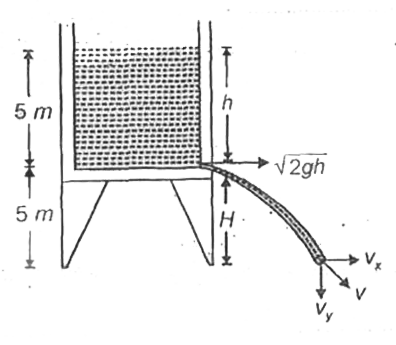
View Solution
Knowledge Check
An open water tank has an orifice 10 cm below the surface of water. If there is no loss of energy, then what is the speed of efflux ? (Take g = 10 m s−2)
A1.414 m s−1B2.5 m s−1C6.32 m s−1D3.7 m s−1A tank containing water has an orifice in one vertical side. If the centre of orifice is 4.9 m below the surface level in the tank, the velocity of discharge is:
A4.9 metre/secondB9.8 metre/secondC2.45 metre/secondDzeroA water tank has a hole in its wall at a distance of 40 m below the free surface of water. Compute the velocity of flow of water from the hole. If the radius of the hole is 1 mm. find the rate of flow of water
A26m/s,8.8×10−5m3/sB28m/s,8.8×10−5m3/sC28m/s,6.8×10−5m3/5D26m/s,9.8×10−5m3/s
A rectangular water tank of base 11m×6m contains water up to a height of 5 m. If the water in the tank is transferred to a cylindrical tank of radius 1.75 m, find the height of the water level in the tank.
View SolutionA rectangular water tank of base 11m×6m contains water upto a height of 5 m. If the water in the tank is transferred to a cylindrical tank of radius 3.5 m, find the height of the water level in the tank.
View SolutionA boat carrying steel balls is floating on the surface of water in a tank. If the balls are thrown into the tank one by one, how will it affect the level of water ?
View SolutionA tank with vertical walls is monted so that its base is at height of 1.2 m above the horizotnal ground. The tank is filled with water to depth 2.8 m. A holw is punched in the side wall of the tank at a depth x m below the surface of water to have maximum range of the emerging stream. then the value of x in metre is
View SolutionA primitive diving bell consists of a cylindrical tank with one end open and one end closed. The tank is lowered into a freshwater lake, open end downward. Water rises into the tank, compressing the trapped air, whose temperature remains constant during the descent. The tank is brought to a halt when the distance between the surface of the water in the tank and the surface of the lake is 40.0 m. Atmospheric pressure at the surface of the lake is 1.01×105 Pa. Find the fraction of the tank's volume that is filled with air.
View Solution
