In non inertial frames,Newton's second law of motion is written as
where a= acceleration of the body, relative to the non-inertial fram and Fp is the pseudo force.
→F=m→a
→F=m→a+→Fp
→F=m→a−→Fp
→F=2ma
The correct Answer is:C
To express Newton's second law of motion in a non-inertial frame, we need to consider the effects of pseudo forces that arise due to the acceleration of the frame itself. Here's a step-by-step breakdown of how this is done:
1. Understanding Non-Inertial Frames:
- A non-inertial frame is one that is accelerating. For example, if you are in a lift (elevator) that is accelerating upwards, you are in a non-inertial frame.
2. Identifying the Forces:
- In a non-inertial frame, the forces acting on an object include not only the real forces (like gravity) but also a pseudo force that appears due to the acceleration of the frame itself.
3. Defining the Pseudo Force:
- The pseudo force Fp acts in the opposite direction to the acceleration of the non-inertial frame. If the frame is accelerating upwards with an acceleration A, then the pseudo force acting on a mass m is given by:
Fp=−mA
4. Applying Newton's Second Law:
- In an inertial frame, Newton's second law states:
F=ma
- In a non-inertial frame, we modify this to account for the pseudo force:
Fnet=ma+Fp
- Substituting the expression for the pseudo force:
Fnet=ma−mA
5. Rearranging the Equation:
- We can rearrange this equation to express the net force in terms of the acceleration of the object relative to the non-inertial frame:
Fnet=m(a−A)
- This shows that the effective acceleration of the object in the non-inertial frame is a−A.
6. Final Expression:
- Thus, in a non-inertial frame, Newton's second law can be expressed as:
Fnet=ma+mA
- Where a is the acceleration of the body relative to the non-inertial frame and A is the acceleration of the frame itself.
Summary:
In non-inertial frames, Newton's second law is modified to include a pseudo force that accounts for the acceleration of the frame. The final expression is:
Fnet=ma+mA
- A non-inertial frame is one that is accelerating. For example, if you are in a lift (elevator) that is accelerating upwards, you are in a non-inertial frame.
- In a non-inertial frame, the forces acting on an object include not only the real forces (like gravity) but also a pseudo force that appears due to the acceleration of the frame itself.
- The pseudo force Fp acts in the opposite direction to the acceleration of the non-inertial frame. If the frame is accelerating upwards with an acceleration A, then the pseudo force acting on a mass m is given by:
Fp=−mA
- In an inertial frame, Newton's second law states:
F=ma
- In a non-inertial frame, we modify this to account for the pseudo force:
Fnet=ma+Fp
- Substituting the expression for the pseudo force:
Fnet=ma−mA
- We can rearrange this equation to express the net force in terms of the acceleration of the object relative to the non-inertial frame:
Fnet=m(a−A)
- This shows that the effective acceleration of the object in the non-inertial frame is a−A.
- Thus, in a non-inertial frame, Newton's second law can be expressed as:
Fnet=ma+mA
- Where a is the acceleration of the body relative to the non-inertial frame and A is the acceleration of the frame itself.
In non-inertial frames, Newton's second law is modified to include a pseudo force that accounts for the acceleration of the frame. The final expression is:
Fnet=ma+mA
Topper's Solved these Questions
Similar Questions
Knowledge Check
Non-inertial frame of reference have
Azero acceleration.Bsome acceleration with respect to inertial frame of reference.Czero velocity.Dconstant velocity with respect to inertial frame of reference.If the Newton's laws of motion are to hold true in an accelerated frame of reference then the observer in this frame of reference will assume the existence of pseudo force known as
Agravitation forceBcentripetal forceCcentrifugal forceDrestoring forceWhen a body is moving uniformly along a straight line and there is no force of friction, acceleration/retardation of the body. According to Newton's second law of motion, the magnitude of force acting on a body is the product of mass and acceleration of the body
The distance - time table of an object in motion is given below
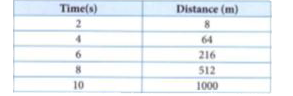
In the given table, acceleration of the object isAincreasingBdecreasingCconstantDnone of these
Explain how does Newton's second law of motion give a quantitative definition of force.
View SolutionInertial and Non Inertial Frame||Pseudo Force
View SolutionAccording to newtons third law of motion a force acting on a body results in its acceleration .
View SolutionState Newton’s second law of motion. How does it help to measure force? Also state the units of force.
View SolutionState and Explain Newton s second law of motion Hence , Deduce the relation F = ma Where the symbols have their usual meaning .
View Solution
