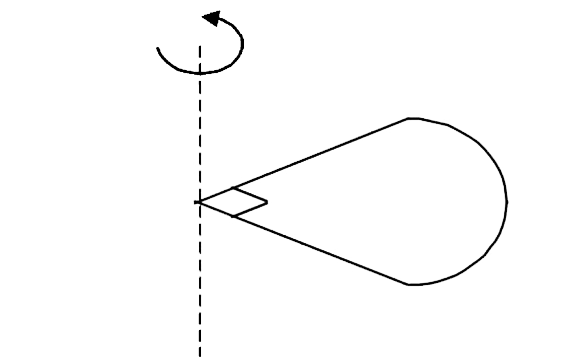
One quarter sector is cut from a uniform disc of radius R. This sector has mass M. It is made to rotate about a line perpendicular to its plane and passing through the centre of the original disc. What is its moment of inertia about the axis of rotation ?
MR28
√2MR2
MR24
MR22
The correct Answer is:D
To find the moment of inertia of a quarter sector cut from a uniform disc of radius R and mass M about an axis perpendicular to its plane and passing through the center of the original disc, we can follow these steps:
1. Identify the mass of the full disc:
Since the mass of the quarter sector is M, the mass of the full disc can be calculated as:
Mfull=4M
This is because a quarter of the disc has 14 of the total mass.
2. Calculate the moment of inertia of the full disc:
The moment of inertia I of a full disc about an axis passing through its center and perpendicular to its plane is given by the formula:
Ifull=12MfullR2
Substituting Mfull=4M:
Ifull=12(4M)R2=2MR2
3. Determine the moment of inertia of the quarter sector:
The moment of inertia of the quarter sector about the same axis can be found by taking 14 of the moment of inertia of the full disc since the mass of the quarter sector is M (which is 14 of the full mass):
Iquarter=14Ifull=14(2MR2)=12MR2
4. Final Result:
Therefore, the moment of inertia of the quarter sector about the specified axis is:
Iquarter=12MR2
Since the mass of the quarter sector is M, the mass of the full disc can be calculated as:
Mfull=4M
This is because a quarter of the disc has 14 of the total mass.
The moment of inertia I of a full disc about an axis passing through its center and perpendicular to its plane is given by the formula:
Ifull=12MfullR2
Substituting Mfull=4M:
Ifull=12(4M)R2=2MR2
The moment of inertia of the quarter sector about the same axis can be found by taking 14 of the moment of inertia of the full disc since the mass of the quarter sector is M (which is 14 of the full mass):
Iquarter=14Ifull=14(2MR2)=12MR2
Therefore, the moment of inertia of the quarter sector about the specified axis is:
Iquarter=12MR2
Topper's Solved these Questions
Similar Questions
One quarter sector is cut from a uniform circular disc of radius R. This sector has mass M. it is made to rotate about a line perpendicular to its plane and passing through the center of the original disc. Its moment of inertia about the axis of rotation is
 View Solution
View SolutionThe M.I. of a uniform semicircular disc of mass M and radius R about a line perpendicular to the plane of the disc and passing through the centre is
View Solution
Knowledge Check
- Question 1 - Select One
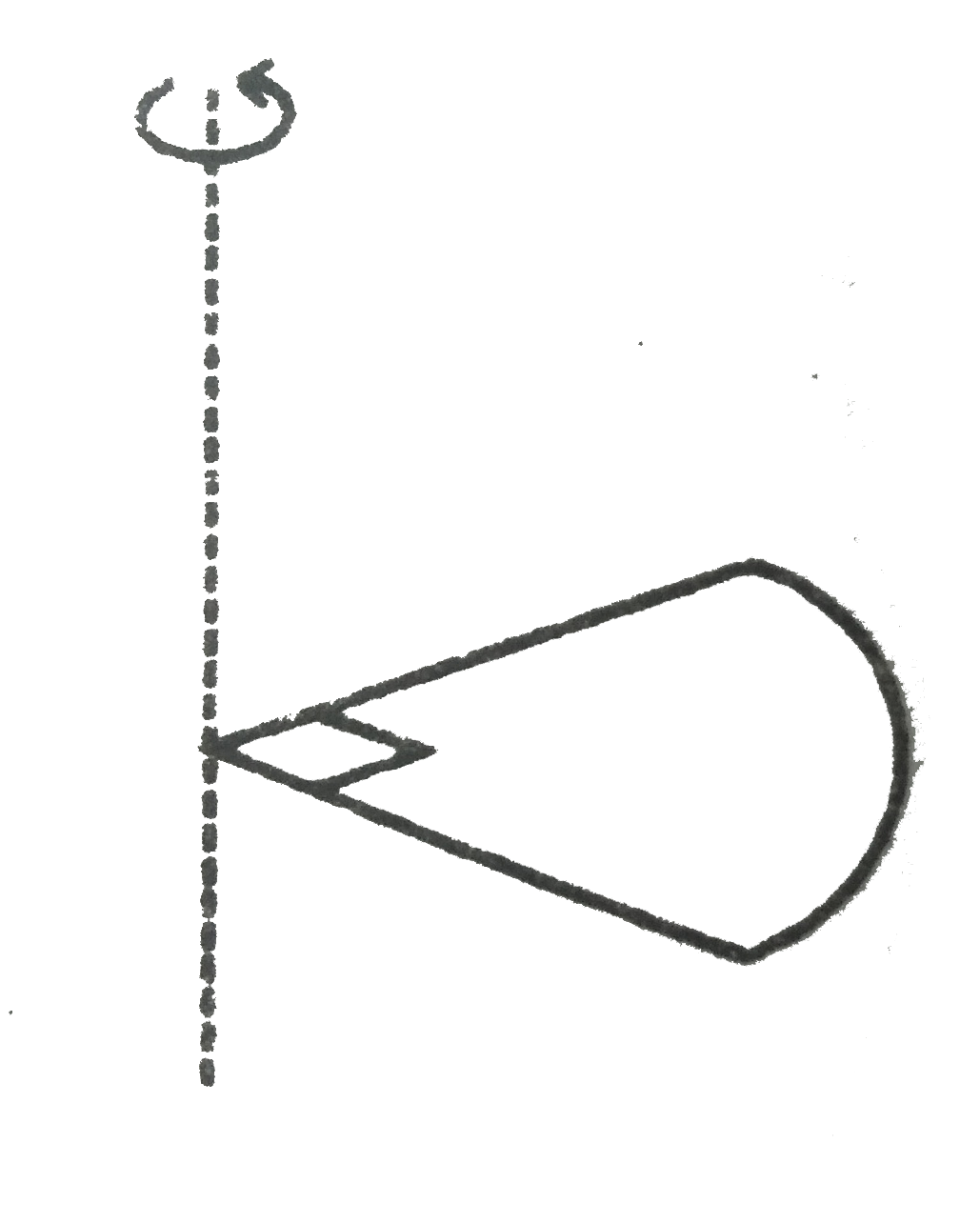
One quarter sector is cut from a uniform circular disc of radius R. This sector has mass M. It is made to rotate about a line perpendicular to its plane and passing through the centre of the original disc. It moment of inertia about the axis of rotation is.
 .A12MR2B14MR2C18MR2D√2MR2
.A12MR2B14MR2C18MR2D√2MR2 - Question 2 - Select One
One quarter sector is cut from a uniform circular disc ofradius R. This sector has mass M. It is made to rotate about a line perpendicular to its plane and passing through the centre of the original disc. Its moment of inertia about the axis of rotation is
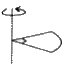 A12MR2B14MR2C18MR2D√2MR2
A12MR2B14MR2C18MR2D√2MR2 - Question 3 - Select One
One quarter sector is cut from a uniform circular disc of radius R. This sector has mass M. It is made to rotate about a line perpendicular to its plane and passing through the centre of the original disc. It moment of inertia about the axis of rotation is.
 .A12MR2B14MR2C18MR2D√2MR2
.A12MR2B14MR2C18MR2D√2MR2
Radius of gyration of disc rotating about an axis perpendicular to its plane passing through through its centre is (If R is the radius of disc)
View SolutionMoment of inertia of a uniform quarter disc of radius R and mass M about an axis through its centre of mass and perpendicular to its plane is :
View SolutionThe moment of inertia of a uniform semicircular disc of mass M and radius r about a line perpendicular to the plane of the disc through the center is
View SolutionCircular disc of mass 2 kg and radius 1 m is rotating about an axis perpendicular to its plane and passing through its centre of mass with a rotational kinetic energy of 8 J. The angular momentum is (Js) is
View SolutionA quarter disc of radius R and mass m is rotating about the axis OO' (perpendicular to the plane of the disc) as shown. Rotational kinetic energy of the quarter disc is
 View Solution
View Solution

