Using dimensional analysis the resistivity in terms of fundamental constants h,me,c,e,ε0 can be expressed as
hε0mece2
ε0mece2h
h2mece2
meε0ce2
The correct Answer is:C
Step by step video, text & image solution for Using dimensional analysis the resistivity in terms of fundamental constants h, m_(e), c, e, epsilon_(0) can be expressed as by Physics experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Topper's Solved these Questions
Similar Questions
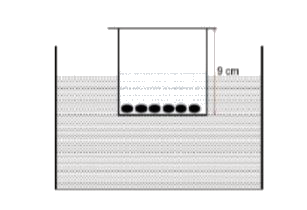
A wide bottom cylindrical massless plastic container of height 9 cm has 40 identical coins inside it and is floating on water with 3 cm inside the water. If we start putting more of such coins on its lid. It is observed that after N coins are put, its equilibrium changes from stable to unstable. Equilibrium in floating is stable if the geometric center of the submerged portion is above the center of mass of the object) The value of N is closed to
View SolutionIf m,e,ε0h and c denote mass ,electron , change of electron, plank 's constant and speed of light , respectively , then the dimensions of me4ε20h2c are
View SolutionThe dimensions of e2/4πε0hc, where e,ε0,h and c are electronic charge, electric permittivity, Planck’s constant and velocity of light in vacuum respectively
View SolutionThe Quantum Hall Resistance RH is a fundamental constant with dimensions of resistance. If h is Planck's constant and e the electron charge, then the dimension of RH is the same as
View SolutionCheck the correctness of the equation E=mc2 using dimensional analysis method.
View SolutionCheck the correctness of the equation E=mc2 using dimensional analysis method.
View SolutionDimensional formula of e4ε20mpm2ec3G is (Where e= charge , mp and mc are masses, ε0= permittivity of free space, c= velocity of light and G= gravitational constant)
View SolutionIf epsilon_(0) is permittivity of free space, e is charge of proton, G is universal gravitational constant and m_(p) is mass of a proton then the dimensional formula for (e^(2))/(4pi epsilon_(0)Gm_(p)^(2)) is
View SolutionThe dimensional formula of 12ε0E2 is (E=electric field)
View SolutionStatement-I: Dimensional analysis can give us the numerical value of proportionality constants that may appear in an algebraic expression.
Statement-II: Dimensional analysis make use of the fact that dimensions can be treated as algebraic quantities.
View SolutionStatement - 1: Dimensional analysis can give statistical value of proportional constant appearing in algebraic expression. Statement-2: Dimensional analysis can use the fact that dimensions can be algebraic quantities.
View Solutione2/4πε0hc की विमा क्या होगी जहां e,ε0,h एवं c क्रमशः इलेक्ट्रॉनिक आवेश विद्युतशीलता, प्लान स्थिरांक तथा निर्वात में प्रकाश का वेग है
View Solution(1)/(epsilon_(0))(e^(2))/(hc) Is the dimensional formula of
View SolutionThe dimension of the quantity 1ε0e2hc is (e = charge of electron, h = Planck's constant and c = velocity of light)
View SolutionAmount 1/epsilon_(0) e^(2)/h_(c) The dimension is - (e = charge of electron, h = constant of Planck and c = velocity of light)
View Solution
