A cylinder of length 42cm is divided into chambers of equal volumes and each half contains a gas of equal mass at temperature 27∘C. The separator is a frictionless piston of insulating material. Calculate the distance by which the piston will be displacement if the temperature of one half is increaded to 57∘C.
The correct Answer is:1cm
Step by step video, text & image solution for A cylinder of length 42cm is divided into chambers of equal volumes and each half contains a gas of equal mass at temperature 27^(@)C. The separator is a frictionless piston of insulating material. Calculate the distance by which the piston will be displacement if the temperature of one half is increaded to 57^(@)C. by Physics experts to help you in doubts & scoring excellent marks in Class 11 exams.
Topper's Solved these Questions
Similar Questions
A horizontally kept cylindrical vessel closed at both ends is separated into two equal (42 cm each) parts by an adiabatic piston. Both parts contain gas having equal mass at a temperature of 27∘C and a pressure of 1 atm. How much should the gas be heated in one part of the cylinder to shift the piston by 2 cm? Find the pressure p of the gas after shifting of the piston.
View SolutionA vertical cylinder closed at both ends is divided into two parts by a frictionless piston, each part containing one mole of air. At 300K the volume of the upper part is 4 times of the lower part. At what temperature will the volume of the upper part be three times that of the lower part?
View Solution
Knowledge Check
- Question 1 - Select One
A cylinder closed at both ends is separated into two equal parts (45 cm each) by a piston impermeable to heat. Both the parts contain the same masses of gas at a temperature of 300 K and a pressure of 1 atm. If now the gas in one of parts is heated such that the piston shifts by 5 cm, then the temperature and the pressure of the gas in this part after heating is
AT = 365 K and P = 1.125 atmBT = 350 K and P = 1.125 atmCT= 375 K and P = 2.125 atmDT = 375 K and P = 1.125 atm - Question 2 - Select One
An insulated container of gas has two chambers separated by an insulating partition. One of the chambers has volume V1 and contains ideal gas at pressure P1 and temperature T1. The other chambers has volume V2 and contains ideal gas at pressure P2 and temperature T2. If the partion is removed without doing any work on the gas, the final equilibrium temperature of the gas in the container will be
AT1T2(P1V1+P2V2)P1V1T2+P2V2T1BP1V1T1+P2V2T2P1V1+P2V2CP1V1T2+P2V2T1P1V1+P2V2DT1T2(P1V1+P2V2)P1V1T1+P2V2T2
An horizontal insulated cylindrical vessel of length 2l is separated by a thin insulating piston into two equal parts as shown. Each part contains n moles of an ideal monoatomic gas at temperature T. The piston is connected to end faces of cylinder with help of two springs each of spring constank k. Initially both springs are in relaxed state. The temperature of left part of cylinder is kept constant by some external means while heat is supplied to right part with help of filament. Answer following questions.
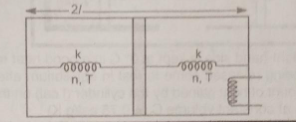
If energy Q is supplied to right part so that the piston moves to left by l/4 then change in internal energy of right part of cylinder isView SolutionAn horizontal insulated cylindrical vessel of length 2l is separated by a thin insulating piston into two equal parts as shown. Each part contains n moles of an ideal monoatomic gas at temperature T. The piston is connected to end faces of cylinder with help of two springs each of spring constank k. Initially both springs are in relaxed state. The temperature of left part of cylinder is kept constant by some external means while heat is supplied to right part with help of filament. Answer following questions.
temperature of gas in right part in final equilibrium isView SolutionAn horizontal insulated cylindrical vessel of length 2l is separated by a thin insulating piston into two equal parts as shown. Each part contains n moles of an ideal monoatomic gas at temperature T. The piston is connected to end faces of cylinder with help of two springs each of spring constank k. Initially both springs are in relaxed state. The temperature of left part of cylinder is kept constant by some external means while heat is supplied to right part with help of filament. Answer following questions.
heat given by left part of cylinder to external arrangement isView SolutionA vertical cylinder of height 100cm contains air at a constant temperature. The top is closed by a friction less light piston. The atmospheric pressure is equal to 75 cm of mercury. Mercury is slowly poured over the piston. Find the maximum height of the mercury column that can be put on the piston.
View Solutionshows a cylindrical tube of cross-sectional area A fitted with two frictionless pistons. The pistons are connected to each other by a metallic wire. Initially, the temperature of the gas is T0and its pressure is p0which equals the atmospheric pressure, (a)What is the tention in the wire?(b)What will be the tention if the temperature is increased to 2T_(0)?
 View Solution
View SolutionA vertical cylinder closed at both ends is divided into two parts by a frictionless piston, each part containing one mole of air. At 300K the volume of the upper part is 4 times of the lower part. At what temperature will the volume of the upper part be three times that of the lower part?
View Solution

