Two coils of self inductance L(1) and L(2) are connected by in parll

Shown in the figure is an $R - L$ circuit. Just after the key $(K)$ is closed

Athe current in the circuit is zero
Bpotential drop across the resistor is zero
Cemf developed across the inductor equals the emf of the battery
Dno heat is dissipated in the circuit
Two coils of self-inductance $L_{1}$ and $L_{2}$ are placed closed to each other so that total flux in one coil is completely linked with other. If $M$ is mutual inductance between them, then
A $M = L_{1} L_{2}$
B $M = L_{1} / L_{2}$
C $M = \sqrt{L_{1} L_{2}}$
D $M = {(L_{1} L_{2})}_{1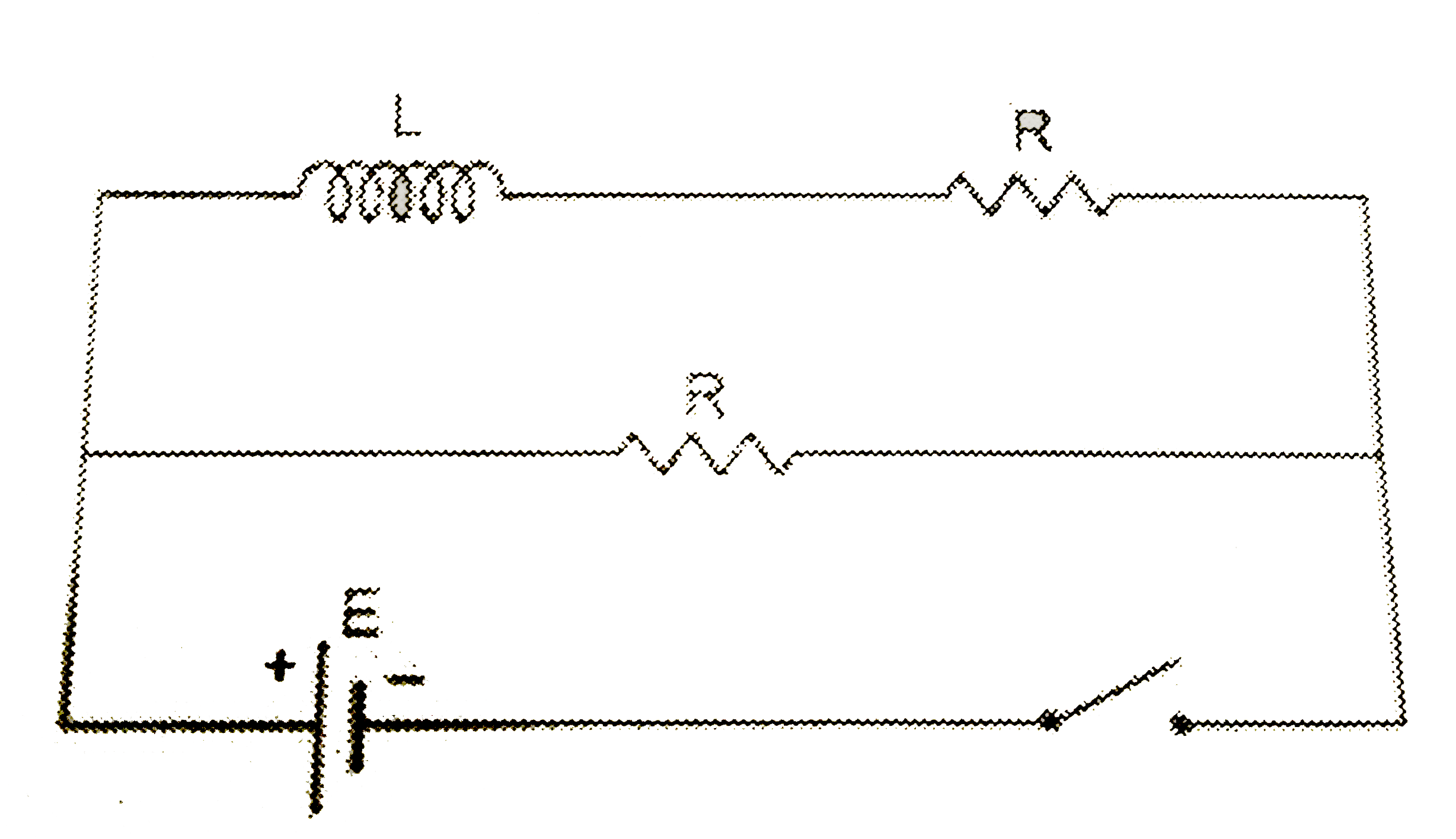}^{2}$
A coil of self -inductance $L$ and resistance $R$ is connected to a resistance $R$ and a cell of emf $E$ as shown. The switch is kept closed for a long time and then opened. The heat produced in the coil, after opening the switch is
A $L E^{2} / 2 R^{2}$
B $L E^{2} / 4 R^{2}$
C $L E^{2} / 8 R^{2}$
D $2 L E^{2} / 3 R^{2}$

Two coils of self inductance $L_{1}$ and $L_{2}$ are connected by in parllel and the then to cell of emf $ε$ and of resistance R throught a Key . Find the instantaneous current throught L after the key is closed .