Question

While travelling through the Syrian Desert, you are in desperate need of water. The owl, still bursting with energy(God knows how) comes flying back to you to tell you that she has located a water faucet nearby. Hearing this, you rush to it, to find a real water cooler. But the problem is, it has seven pieces lying on the ground which have to be fitted to form a rectangle in order to have the faucet working.

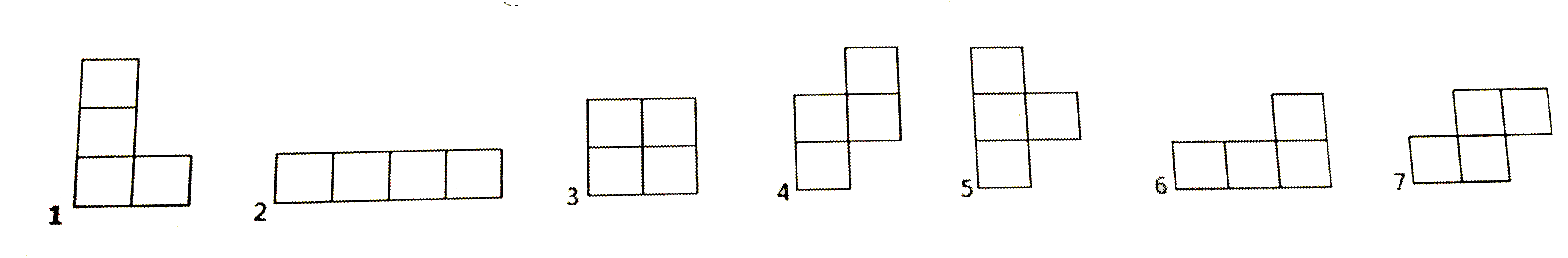
Which of the following statements are correct about the rectangle formed?

Options

A. The corner four pieces are 1,3,5,7
B. The corner four pieces are 1,2,5,6
C. The corner four pieces are 2,4,5,7
D. Rectangle cannot be formed

Explanation

D
The 7 individual pieces, add up to a total of 28 squares. Therefore, assuming we can indeed form it into a rectangle, it would have to be 7x4 or 14x2 squares in size. I’m using the former case here simply because it’s a more natural shape, however this proof applies equally as well to the latter. Now imagine that we label each of these squares with a colour - either black or white - such that they form a checkerboard pattern as shown beside. Notice that the number of black squares must be equal to the number of white, a property we’ll exploit. So that’s 14 black squares, and 14 white. Looking at each of the pieces individually, the issue with our assumption quickly appears.
As shown beside, for pieces 1-6, the number of black squares within the piece is equal to the number of white. Clearly which squares are black and which are white depends on the actual placement of the piece within the rectangle, but the shapes themselves dictate the count of each colour (since adjacent squares must be of different colours).
However, piece 7 disrupts the trend. Irrelevant of how it’s located, it must be comprised of 3 squares of one colour, and 1 of the other, a property that is purely down to its shape. So, taking that into account along with the other 6 pieces, in total they’re comprised of 13 squares of one colour, and 15 of the other, with no assumptions about how they’re located within the rectangle. We needed 14 of each, and since we’ve just shown that we can’t get that, our original assumption is overturned and our proof is complete.