Question

Seeing that Max has managed to solve the puzzle, the trainer gives Max another challenge. The trainer has a dozen Pokéballs, arranged in a $3\times 4$ grid, which for convenience we have labeled A through L.

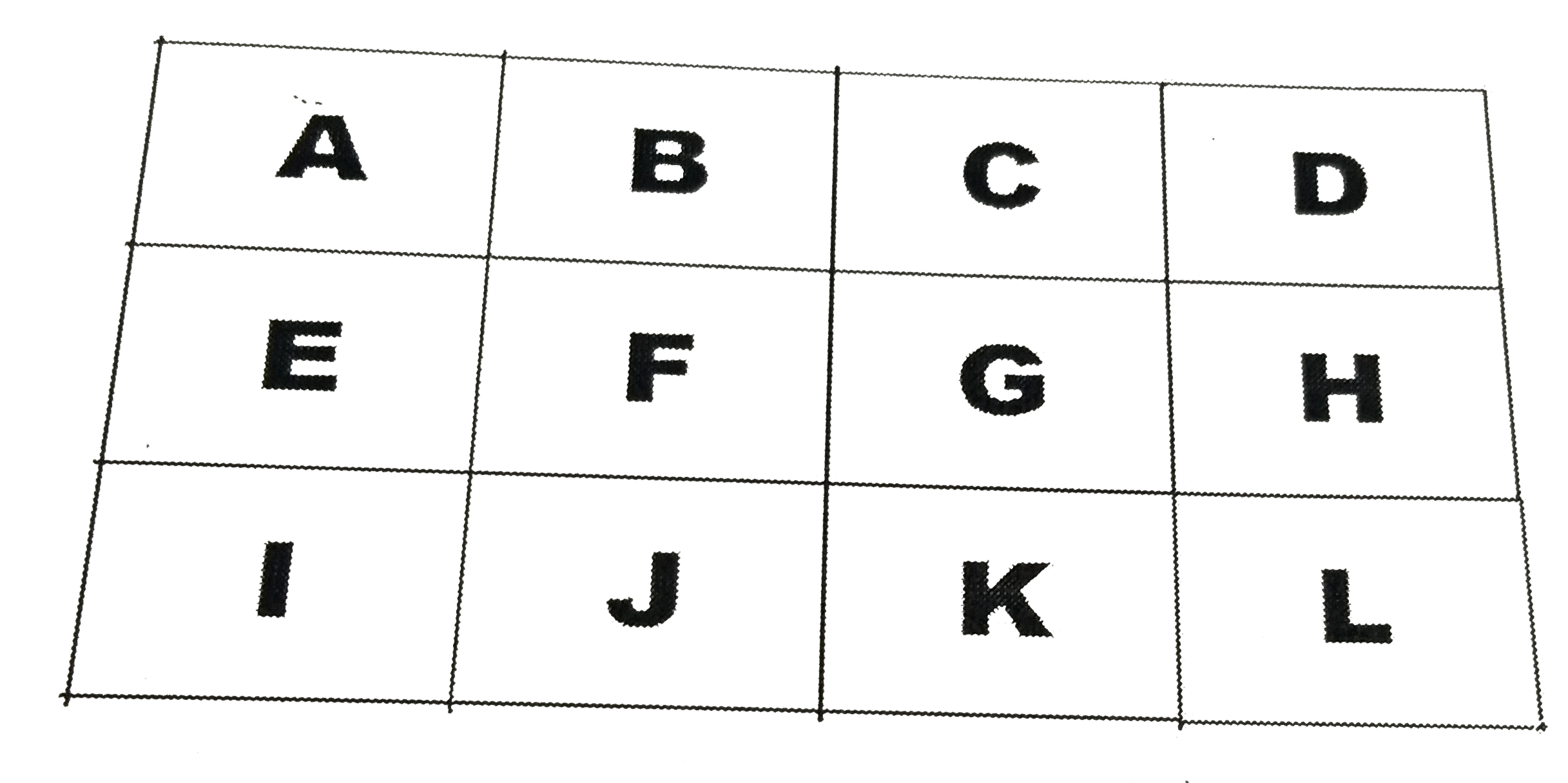
Two of the pokéballs are chosen randomly, and they have a pokémon inside them. The other ten are empty. Max is given two options. He can either open them in the order A,B,C,D,E,F,G,H,I,J,K,L (Option A) or he can open in the order A, E, I, B, F, J, C, G, K, D, H, L (Option B). Whatever option Max chooses, the trainer will choose the other. You stop when you have found a pokéball with a pokémon inside. Your score is the number of pokéballs you have opened. Once you have found a pokéball with a pokémon, you once again put the pokémon back in the same pokéball and leave it intact. The one with the lowest score wins. For example, let the pokémon be in H and K. Let’s say Max chooses Option A. Then he will find the first pokémon in H, and will score 8 points. Whereas the trainer, following option B, will find his first Pokémon in K, and score 9 points. Which option should Max choose so that he has a higher chance of winning the game ?

Options

A. A, B, C, D, E, F, G, H, I, J, K, L
B. A, E, I, B, F, J, C, G, K, D, H, L
C. Choice doesn't matter
D. Can’t decide without knowing the Pokéballs that have the pokémon

Explanation

Option A is more likely to win. Let max choose option A and Brock choose option B. And let’s assume a new character, Alice selects the pokeball that will contain the pokemon. Label a Pokeball with a "b" (respectively, a "c") if Max (respectively, Brock) reaches that Pokeball more quickly, and also record Max's (respectively, Brock's) score upon reaching that Pokeball. Label Pokeballs A and L with "xx" since both players reach those Pokeballs simultaneously. We obtain:
`{:(x x,b2,b3,b4),(c2,c5,b7,b8),(c3,c6,c9,x x):}`
Note that there are five b Pokeballs and five c Pokeballs. So the cases in which the pokemon is in pokeball A or Pokeball L are equally split between Max and Brock. Similarly if Alice selects two b Pokeballs then Max necessarily wins, but these are balanced out by an equal number of cases in which Alice selects two c Pokeballs and C necessarily wins. The crucial cases occur when Alice selects one b Pokeball and one c Pokeball. Max wins if the b Pokeball has a lower score than the c Pokeball:
b2 and (c3 or c5 or c6 or c9)
b3 adn (c5 or c6 or c9) ltbr. b4 and (c5 or c6 or c9)
b7 and c9
b8 and c9
Brock wins if the c Pokeball has a lower score than the b Pokeball: c2 and (b3 or b4 or b7 or b8)
c3 and (b4 or b7 or b8)