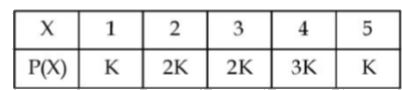
The probability distribution of a random variable X is given as
X−5−4−3−2−1012345P(X)p2p3p4p5p7p8p9p10p11p12p
Then, the value of p is
172
373
572
174
The correct Answer is:A
To find the value of p in the given probability distribution of the random variable X, we follow these steps:
Step 1: Write down the probability distribution
The random variable X takes the values:
X={−5,−4,−3,−2,−1,0,1,2,3,4,5}
with corresponding probabilities:
P(X)={p,2p,3p,4p,5p,7p,8p,9p,10p,11p,12p}
Step 2: Set up the equation for total probability
The sum of all probabilities must equal 1:
p+2p+3p+4p+5p+7p+8p+9p+10p+11p+12p=1
Step 3: Combine like terms
Add all the coefficients of p:
(1+2+3+4+5+7+8+9+10+11+12)p=1
Calculating the sum of the coefficients:
1+2+3+4+5+7+8+9+10+11+12=72
Thus, we have:
72p=1
Step 4: Solve for p
To find p, divide both sides by 72:
p=172
Final Answer
The value of p is:
172
The random variable X takes the values:
X={−5,−4,−3,−2,−1,0,1,2,3,4,5}
with corresponding probabilities:
P(X)={p,2p,3p,4p,5p,7p,8p,9p,10p,11p,12p}
The sum of all probabilities must equal 1:
p+2p+3p+4p+5p+7p+8p+9p+10p+11p+12p=1
Add all the coefficients of p:
(1+2+3+4+5+7+8+9+10+11+12)p=1
Calculating the sum of the coefficients:
1+2+3+4+5+7+8+9+10+11+12=72
Thus, we have:
72p=1
To find p, divide both sides by 72:
p=172
The value of p is:
172
Topper's Solved these Questions
Similar Questions
Knowledge Check
A random variable has the following probability dustribution.
x:01234567p(x):02p2p3pp22p27p22p
The value of p, isA1/10B−1C−1/10Dnone of theseThe probability distribution of random variable X with two missing probabilities p1 and p2 is given below
XP(X)1k2p134k4p252k
It is further given that P(X≤2)=0.25andP(X≥4)=0.35. Consider the following statements
1. p1=p2
2. p1+p2=P(X=3)
which of the statements given above is/are correct ?A1 onlyB2 onlyCBoth 1 and 2DNeither 1 nor 2For a binomial variable X if n=5 and P(X=1)=8P(X=3), then p=
A45B15C13D23
If a variable takes the discrete values p+4,p−72,p−52,p−3,p−2,p+12,p−12,p+5,p>0, then the median is.
View SolutionA random variable has the following probability distribution: X=xi: 0 1 2 3 4 5 6 7 P(X=xi):0 2p 2p 3p p2 2p2 7p2+p The value of p is a. 110 b. −1 c. −110 d. 15
View SolutionThe random variable X can the values 0, 1, 2, 3, Give P(X = 0) = P(X = 1)= p and P(X = 2) = P(X = 3) such that ∑pix2i=2∑pixi then find the value of p
View SolutionIf a variable takes the discrete values p+4,p−72,p−52,p−3,p−2,p+12,p−12,p+5(p>0), and the median p−K then find k
View SolutionThe probability function of a binomial distribution is P(x)=(6x)pxq6−x,x=0,1,2..........,6. If 2P(2)=3P(3), then p=
View Solution