A 100 Hz a.c. is flowing in a 14 mH coil. Find its reactance.
To find the reactance of a coil in an AC circuit, we can use the formula for inductive reactance (X_L):
XL=ωL
Where:
- ω (angular frequency) is given by ω=2πf
- L is the inductance in henries.
1. Identify the given values:
- Frequency f=100Hz
- Inductance L=14mH=14×10−3H
2. Calculate the angular frequency ω:
ω=2πf=2π×100
ω=200πrad/s
3. Substitute ω and L into the reactance formula:
XL=ωL=(200π)×(14×10−3)
4. Calculate XL:
XL=200×3.14×14×10−3
XL=200×3.14×0.014
XL=200×0.04396
XL≈8.792ohms
5. Round the result:
XL≈8.8ohms
Final Answer:
The reactance XL of the coil is approximately 8.8ohms.
---
- ω (angular frequency) is given by ω=2πf
- L is the inductance in henries.
- Frequency f=100Hz
- Inductance L=14mH=14×10−3H
ω=2πf=2π×100
ω=200πrad/s
XL=ωL=(200π)×(14×10−3)
XL=200×3.14×14×10−3
XL=200×3.14×0.014
XL=200×0.04396
XL≈8.792ohms
XL≈8.8ohms
The reactance XL of the coil is approximately 8.8ohms.
Topper's Solved these Questions
Similar Questions
Knowledge Check
- Question 1 - Select One
The resistance of a coil for direct current is 10 ohm. When a.c. is sent through the same coil, its resistance would be
A10ΩB>10ohmC<10ohmDcannot say - Question 2 - Select One
In the circuit shown, a 30V d.c. source gives a current 2.0A as recorded in the ammeter A and 30V a.c. source of frequency 100Hz gives a currentd 1.2A. The inductive reactance is
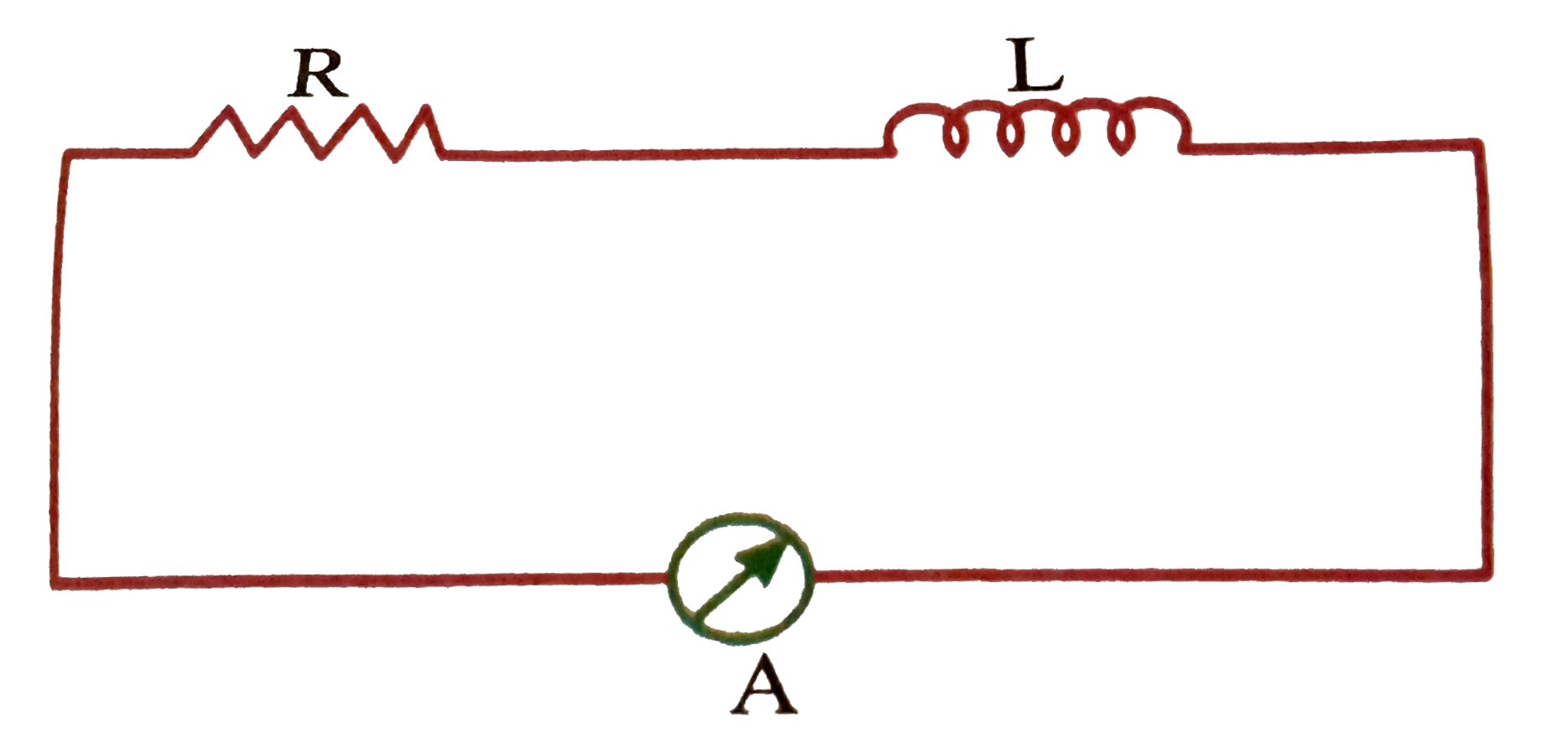 A10 ohmB20 ohmC5√34 ohmD40 ohm
A10 ohmB20 ohmC5√34 ohmD40 ohm - Question 3 - Select One
A circuit has three elements, a resistance fo 11Ω, a coil of inductaive reactance 120Ω and a capacitve reactance of 120Ω in series and connected to an A.C source of 110V,60Hz. Which of the three elements have minimum potential difference?
AResistanceBCapacitanceCInductorDAll will have equal potential difference
Derive the expression of the inductive reactance and for the phase shift in an a.c. circuit containing a coil of zero resistance.
View SolutionThe virtual current in the a.c. circuit shown in fig, 14.15 is 1.0 A. Find (i) virtual coltage across the coil L (ii) impendance of the circuit and (ii) reactance of the coil.
 View Solution
View SolutionIf the frequency of a.c. is doubled then the value of inductive reactance (X) as well as capacitive reactance (X) gets doubled.
View SolutionThe capacitive reactance is 20Ω, when the frequency is 100 Hz. Find the reactance, when frequency is 150 Hz.
View SolutionThe reactance offered by a capcitor to an a.c. of frequency 50 Hz is 15Ω. What would be its reactance if the frequency is increased to 75 Hz?
View Solution

