Calculate the threshold frequency of photon for photoelectric emission from a metal of work function 0.1 eV.
To calculate the threshold frequency of a photon for photoelectric emission from a metal with a work function of 0.1 eV, we can follow these steps:
Step 1: Understand the relationship between work function and threshold frequency
The work function (W) is related to the threshold frequency (ν₀) by the equation:
W=hν0
where:
- W is the work function in joules,
- h is Planck's constant, and
- ν0 is the threshold frequency.
Step 2: Convert the work function from electron volts to joules
Given that the work function is 0.1 eV, we need to convert this to joules. The conversion factor is:
1 eV=1.6×10−19 joules
Thus, we can calculate:
W=0.1 eV×1.6×10−19 J/eV=1.6×10−20 J
Step 3: Use Planck's constant
Planck's constant h is given as:
h=6.63×10−34 J s
Step 4: Rearrange the equation to find the threshold frequency
From the equation W=hν0, we can rearrange it to solve for ν0:
ν0=Wh
Step 5: Substitute the values into the equation
Now, substituting the values we have:
ν0=1.6×10−20 J6.63×10−34 J s
Step 6: Calculate the threshold frequency
Perform the calculation:
ν0=1.6×10−206.63×10−34≈2.41×1013 Hz
Final Answer
The threshold frequency ν0 for photoelectric emission from the metal is approximately:
ν0≈2.41×1013 Hz
---
The work function (W) is related to the threshold frequency (ν₀) by the equation:
W=hν0
where:
- W is the work function in joules,
- h is Planck's constant, and
- ν0 is the threshold frequency.
Given that the work function is 0.1 eV, we need to convert this to joules. The conversion factor is:
1 eV=1.6×10−19 joules
Thus, we can calculate:
W=0.1 eV×1.6×10−19 J/eV=1.6×10−20 J
Planck's constant h is given as:
h=6.63×10−34 J s
From the equation W=hν0, we can rearrange it to solve for ν0:
ν0=Wh
Now, substituting the values we have:
ν0=1.6×10−20 J6.63×10−34 J s
Perform the calculation:
ν0=1.6×10−206.63×10−34≈2.41×1013 Hz
The threshold frequency ν0 for photoelectric emission from the metal is approximately:
ν0≈2.41×1013 Hz
Topper's Solved these Questions
- BOOK - SL ARORACHAPTER - ELECTRONS AND PHOTONSEXERCISE - Problem Solution19videos
ELECTRONS AND PHOTONS
- BOOK - SL ARORACHAPTER - ELECTRONS AND PHOTONSEXERCISE - TYPE A : Problem For Self Practice21videos
ELECTRONS AND PHOTONS
- BOOK - SL ARORACHAPTER - ELECTROMAGNETIC WAVESEXERCISE - Problem for self-practice21videos
ELECTROMAGNETIC WAVES
- BOOK - SL ARORACHAPTER - ELECTROSTATIC FORCES, CHARGES AND FIELDSEXERCISE - Problems For Self Practice1videos
ELECTROSTATIC FORCES, CHARGES AND FIELDS
Similar Questions
Knowledge Check
- Question 1 - Select One
Speed of photons in photoelectric emission is
Asame as light.Bgreater than light speed.Cone third of light speed.Done-fourth of light speed. - Question 2 - Select One
Threshold frequency for photoelectric effect from a metal surface of work function 4.5 eV is
A1.1×109HzB540 HzC1.1×1015 HzDNone of these - Question 3 - Select One
Kmax (in eV) = 3, and frequency of light (in Hz) =1×1015 for a metal used as a cathode in a photoelectric experiment. The threshold frequency of light for the photoelectric emission from the metal is -
A1×1014 HzB1.5×1014 HzC2.1×1014 HzD2.7×1014 Hz
Define the term "threshold frequency", in the context of photoelectric emission.
View SolutionThe work function of a metal is 2,5×(10−19)J. (a) Find the threshold frequency for photoelectric emission. (b) If the metal is exposed to a light beam of frequency (6.0×1014) Hz, what will be the stopping potential ?
View SolutionA metal has a threshold wavelength fo 6000Å. Calculate (i) threshold frequency (ii) work function. Will there be photoelectric emission or not ?
View Solutionrepresents a graph of most energetic photoelectrons Kmax(in eV) and frequency v for a metal used as cathod in photoelectrons experiment. The threshold frequency of light for the photoelectric emission from the metal is
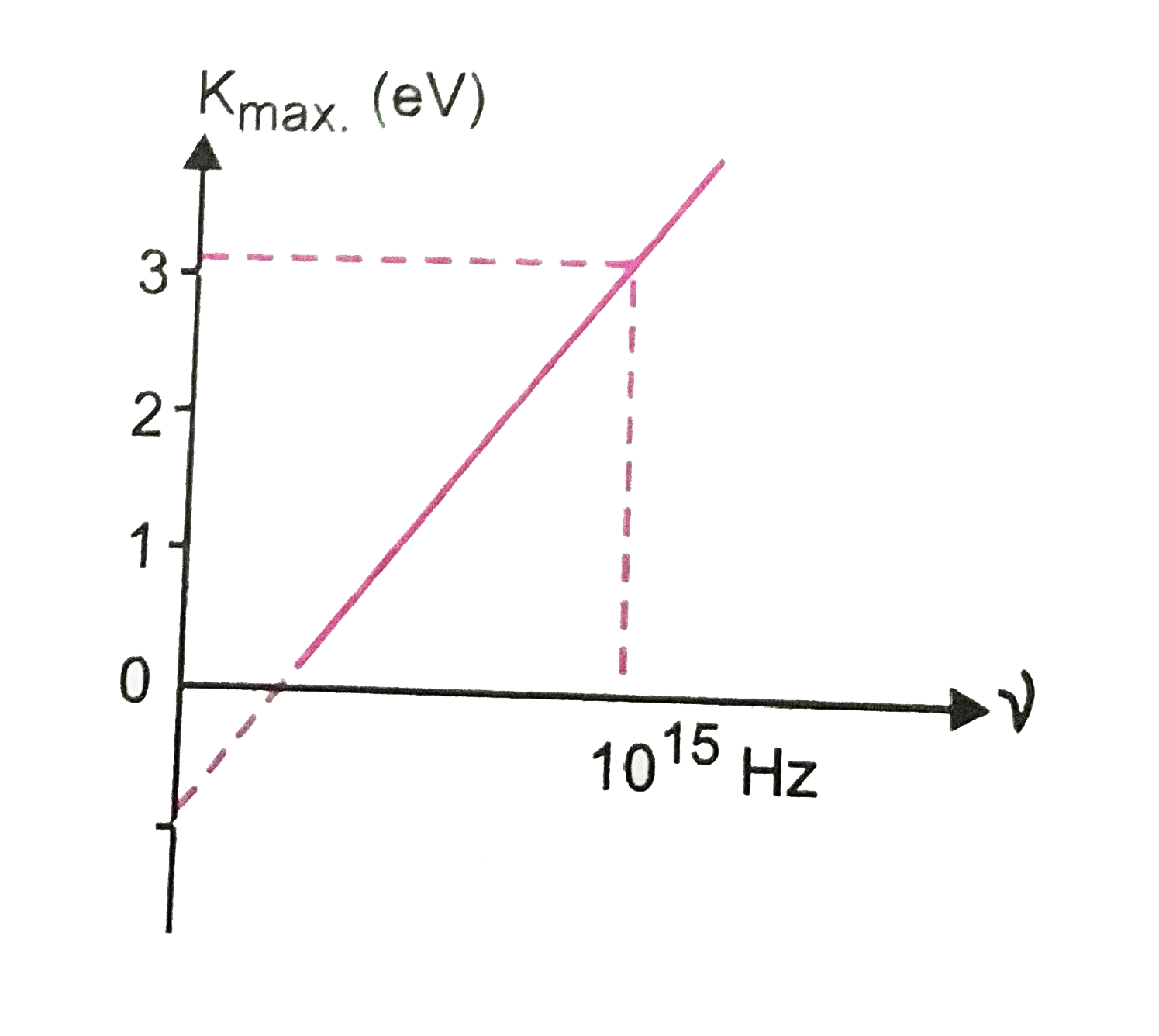 View Solution
View SolutionKmax (in eV) = 3, and frequency of light (in Hz) =1×1015 for a metal used as a cathode in a photoelectric experiment. The threshold frequency of light for the photoelectric emission from the metal is -
View Solution

