A gear ratio r:s is the ratio of the number of teeth of two connected gears. The ratio of the number of revolutions per minute (rpm) of two gear wheels is s:r. In the diagram below, Gear A is turned by a motor. The turning of Gear A causes Gears B and C to turn as well.
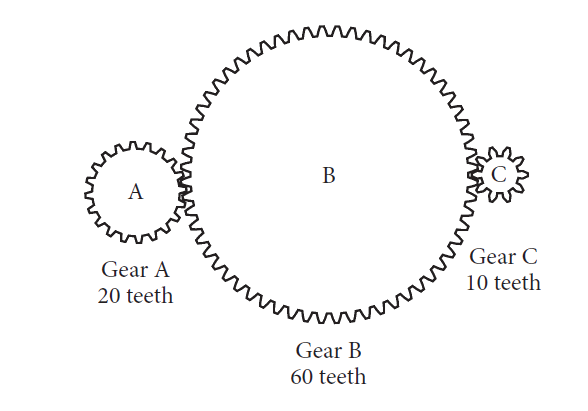
If Gear A is rotated by the motor at a rate of 100 rpm, what is the number of revolutions per minute for Gear C?
50
110
200
1,000
The correct Answer is:C
Since Gear A has 20 teeth and Gear B has 60 teeth, the gear ratio for Gears A and B is 20:60. Thus the ratio of the number of revolutions per minute (rpm) for the two gears is 60:20, or 3:1. That is, when Gear A turns at 3 rpm, Gear B turns at 1 rpm. Similarly, since Gear B has 60 teeth and Gear C has 10 teeth, the gear ratio for Gears B and C is 60:10, and the ratio of the rpms for the two gears is 10:60. That is, when Gear B turns at 1 rpm, Gear C turns at 6 rpm. Therefore, if Gear A turns at 100 rpm, then Gear B turns at 1003 rpm , and Gear C turns at 1003×6=20 rpm
Alternate approach: Gear A and Gear C can be considered as directly connected since their “contact” speeds are the same. Gear A has twice as many teeth as Gear C, and since the ratios of the number of teeth are equal to the reverse of the ratios of rotation speeds, in rpm, Gear C would be rotated at a rate that is twice the rate of Gear A. Therefore, Gear C will be rotated at a rate of 200 rpm since Gear A is rotated at 100 rpm.
Choice A is incorrect and may result from using the gear ratio instead of the ratio of the rpm when calculating the rotational speed of Gear C. Choice B is incorrect and may result from comparing the rpm of the gears using addition instead of multiplication. Choice D is incorrect and may be the result of multiplying the 100 rpm for Gear A by the number of teeth in Gear C.
Similar Questions
A company manufactures the bicycle with gears and without gears in two different factories. The factory A produces 16 bicycles without gears and 20 bicycles with gears in a day. The factory B produces 12 bicycles without gears and 20 bicycles with gears in a day. The expenditure of factory A is Rs. 50,000 daily and the expenditure of factory B is Rs. 40,000 daily. A company has ordered for 96 bicycles without gears and 140 bicycles with gears. To complete these order, how many days the factories will work so that there is minimum expenditure ?
09:09View SolutionThe gear-case was:
View SolutionThe gear-case was:
View SolutionThe following figure shows the three gears, which work in tandem. That means when anyone of the gears rotates, all other gears will also rotate. One gear has 36 teeth, another one has 25 teeth and the third one has 30 teeth. Though the number of teeth is not shown correctly in the given figure. The gears are now in starting position. If you start turning them and continue to turn them, eventually all three will end up at their starting positions. Each gear makesx,y,z complete turns in order to have all three in their starting position. What is the value of x+y+z?
02:34View SolutionWhen the number of teeth of f gear in- creases the speed of rotation…..
View Solutionशुष्क आपंक काम आता है
02:18View SolutionWhat is the antonym of word Geared to?
View SolutionThe teeth of two gears A and B are 100 and 40 respectively. If A is rotating at 500 RPM, then what will be the rotation per minute of B?
03:25View Solution

