f(x)=2x3+6x2+4x
g(x)x2+3x+2
The polynomials f (x ) and g (x ) are defined above. Which of the following polynomials is divisible by 2x+3 ?
h(x)=f(x)+g(x)
p(x)=f(x)+3g(x)
r(x)=2f(x)+3g(x)
s(x)=3f(x)+2g(x)
The correct Answer is:B
To determine which polynomial is divisible by 2x+3, we will analyze the given polynomials f(x) and g(x) and their combinations.
1. Define the Polynomials:
f(x)=2x3+6x2+4x
g(x)=x2+3x+2
2. Option A: h(x)=f(x)+g(x):
h(x)=(2x3+6x2+4x)+(x2+3x+2)
Combine like terms:
h(x)=2x3+(6x2+x2)+(4x+3x)+2=2x3+7x2+7x+2
3. Check Divisibility of h(x) by 2x+3:
We will perform polynomial long division of h(x) by 2x+3.
- Divide the leading term: 2x3÷2x=x2
- Multiply x2 by 2x+3:
2x3+3x2
- Subtract:
(2x3+7x2+7x+2)−(2x3+3x2)=4x2+7x+2
- Next, divide 4x2 by 2x:
4x2÷2x=2x
- Multiply 2x by 2x+3:
4x2+6x
- Subtract:
(4x2+7x+2)−(4x2+6x)=x+2
- Finally, divide x by 2x:
x÷2x=12
- Multiply 12 by 2x+3:
x+32
- Subtract:
(x+2)−(x+32)=2−32=12
Since the remainder is 12, h(x) is not divisible by 2x+3.
4. Option B: p(x)=f(x)+3g(x):
p(x)=(2x3+6x2+4x)+3(x2+3x+2)
Simplifying:
p(x)=2x3+6x2+4x+3x2+9x+6=2x3+9x2+13x+6
5. Check Divisibility of p(x) by 2x+3:
Perform polynomial long division of p(x) by 2x+3.
- Divide the leading term: 2x3÷2x=x2
- Multiply x2 by 2x+3:
2x3+3x2
- Subtract:
(2x3+9x2+13x+6)−(2x3+3x2)=6x2+13x+6
- Next, divide 6x2 by 2x:
6x2÷2x=3x
- Multiply 3x by 2x+3:
6x2+9x
- Subtract:
(6x2+13x+6)−(6x2+9x)=4x+6
- Finally, divide 4x by 2x:
4x÷2x=2
- Multiply 2 by 2x+3:
4x+6
- Subtract:
(4x+6)−(4x+6)=0
Since the remainder is 0, p(x) is divisible by 2x+3.
Conclusion:
The polynomial p(x)=f(x)+3g(x) is divisible by 2x+3.
f(x)=2x3+6x2+4x
g(x)=x2+3x+2
h(x)=(2x3+6x2+4x)+(x2+3x+2)
Combine like terms:
h(x)=2x3+(6x2+x2)+(4x+3x)+2=2x3+7x2+7x+2
We will perform polynomial long division of h(x) by 2x+3.
- Multiply x2 by 2x+3:
2x3+3x2
- Subtract:
(2x3+7x2+7x+2)−(2x3+3x2)=4x2+7x+2
- Next, divide 4x2 by 2x:
4x2÷2x=2x
- Multiply 2x by 2x+3:
4x2+6x
- Subtract:
(4x2+7x+2)−(4x2+6x)=x+2
- Finally, divide x by 2x:
x÷2x=12
- Multiply 12 by 2x+3:
x+32
- Subtract:
(x+2)−(x+32)=2−32=12
p(x)=(2x3+6x2+4x)+3(x2+3x+2)
Simplifying:
p(x)=2x3+6x2+4x+3x2+9x+6=2x3+9x2+13x+6
Perform polynomial long division of p(x) by 2x+3.
- Multiply x2 by 2x+3:
2x3+3x2
- Subtract:
(2x3+9x2+13x+6)−(2x3+3x2)=6x2+13x+6
- Next, divide 6x2 by 2x:
6x2÷2x=3x
- Multiply 3x by 2x+3:
6x2+9x
- Subtract:
(6x2+13x+6)−(6x2+9x)=4x+6
- Finally, divide 4x by 2x:
4x÷2x=2
- Multiply 2 by 2x+3:
4x+6
- Subtract:
(4x+6)−(4x+6)=0
The polynomial p(x)=f(x)+3g(x) is divisible by 2x+3.
Similar Questions
Knowledge Check
- Question 1 - Select One
p(x)=3x3+15x2+18x
q(x)=x2+5x+6
The polynomials p(x) and q(x) are defined above. Which of the following polynomials is divisible by 3x-2?Af(x)=p(x)−2q(x)Bg(x)=2p(x)−3q(x)Ch(x)=3p(x)−2q(x)Dj(x)=4p(x)−3q(x) - Question 2 - Select One
If f(x)=3−xandg(x)=x22, which of the following is NOT in the range of f(g(x) ?
A−3B0C2D4 - Question 3 - Select One
Let f(x)=√x2−4x and g(x) = 3x . The sum of all values for which f(x) = g(x) is
A−8.5B0C8D9.4
If g(x)=2x2+3x−4 and g(f(x))=8x2+14x+1 then f (2) =
View SolutionWhat must be subtracted from the polynomial f(x)=x4+2x3−13x2−12x+21 so that the resulting polynomial is exactly divisible by x4−4x+3 ?
View Solutionf(x)=x3−6x2−19x+84, g(x)=x−7 find the value of f(x)-g(x).
View SolutionWhat must be added to f(x)=4x4+2x3−2x2+x−1 so that the resulting polynomial is divisible by g(x)=x2+2x−3.
View Solution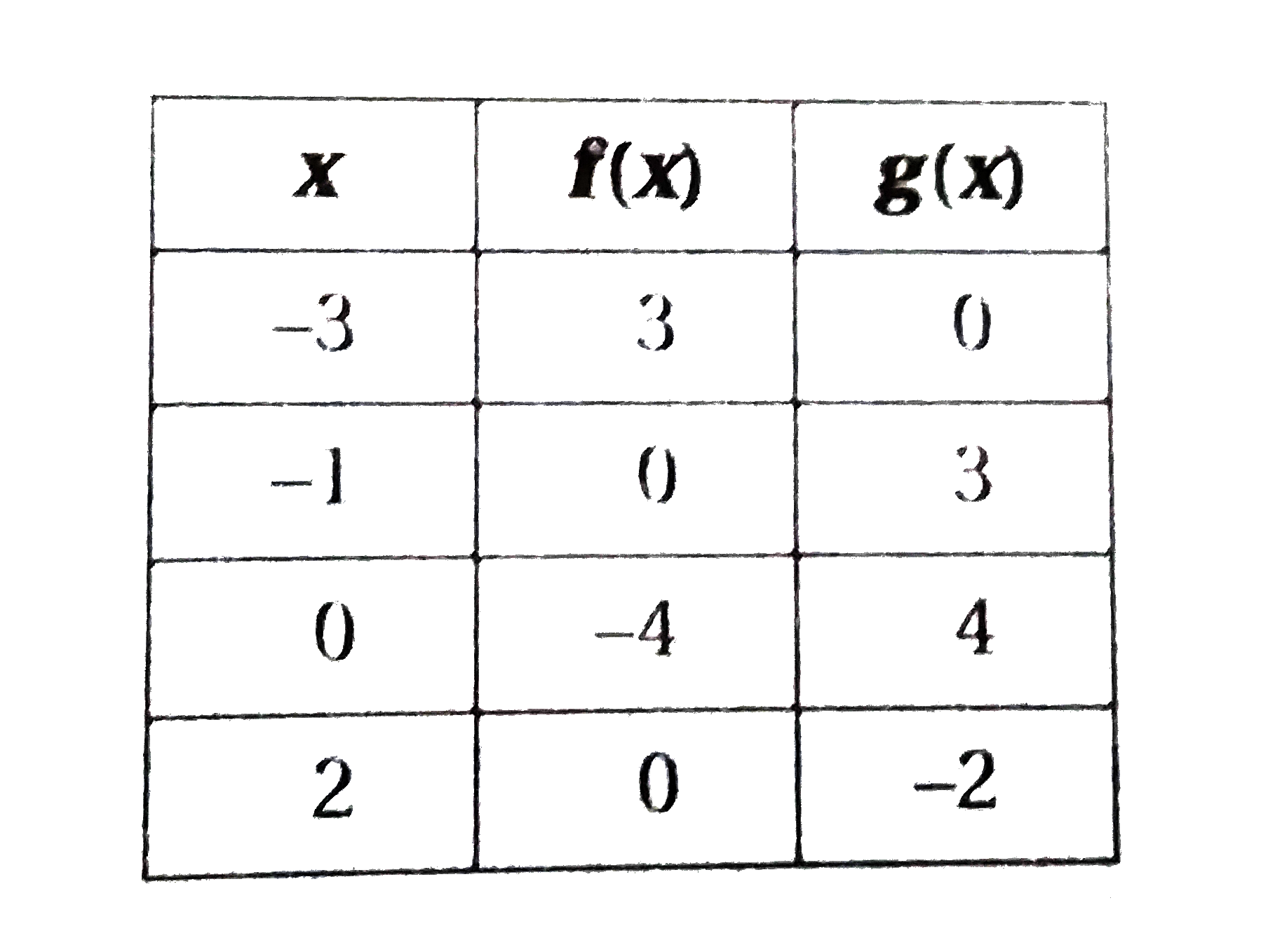
Several values of x, and the corresponding values for polynomial functions f and g are shown in the table above. Which of the following statements is true?
I. f(0)+g(0)=0
II. f(x) is divisible by x+2
III. g(x) is divisible by x+3.View Solution

