The line y=kx+4 , where k is a constant, is graphed in the xy-plane. If the line contains the point (c, d) where c≠0 and d≠0, what is the slope of the line in terms of c and d ?
d−4c
c−4d
4−dc
4−cd
The correct Answer is:A
Step by step video, text & image solution for The line y= kx+4 , where k is a constant, is graphed in the xy-plane. If the line contains the point (c, d) where c ≠ 0 and d ≠ 0, what is the slope of the line in terms of c and d ? by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Similar Questions
Knowledge Check
- Question 1 - Select One
Line k contains the point (4, 0) and has slope 5. Which of the following points is on line k?
A(1, 5)B(3, 5)C(5, 5)D(7, 5) - Question 2 - Select One
Line l is shown in the xy-plane below.
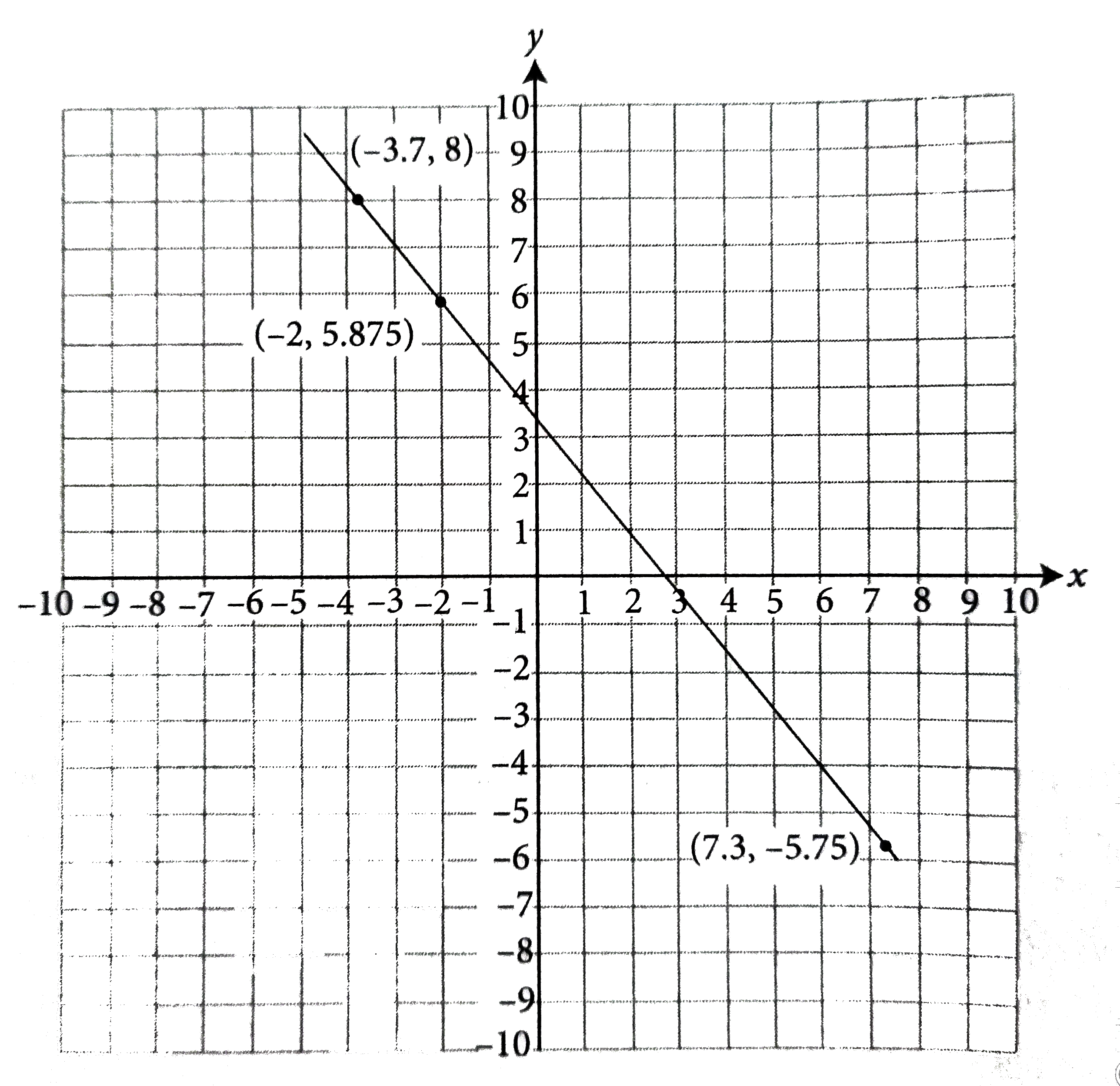
What is the slope of line l?A−1.25B0.80C−0.80D1.25 - Question 3 - Select One
Line m in the xy-plane contains the points (2, 4) and (0, 1).Which of the following is an equation of line m ?
Ay = 2x + 3By = 2x + 4Cy=32x+3Dy=32x+1
Determine the value of k so that the line joining points A(k,1,−1)andB(2,0,2k) is perpendicular to the line joining the points C(4,2k,1)andD(2,3,2).
View SolutionIf →a,→b,→c&→d are position vector of point A,B,C and D respectively in 3-D space no three of A,B,C,D are colinear and satisfy the relation 3→a−2→b+→c−2→d=0 then (a) A, B, C and D are coplanar (b) The line joining the points B and D divides the line joining the point A and C in the ratio of 2:1 (c) The line joining the points A and C divides the line joining the points B and D in the ratio of 1:1 (d)The four vectors →a,→b,→cand→d are linearly dependent .
View SolutionThe slope of a line in the standard (x,y) coordinate plane is 4. What is the slope of a line perpendicular to that line ?
View Solutionf(x)=3x3+kx2−32x+28
The function f is defined by the equation above where k is a nonzero constant. In the xy-plane the graph of f intersects the x-axis at three points: (−2,0),(32,0),and(c,0). What is the value of k?View SolutionThe graph of a line in the xy-plane has slope 12 and contains the point (0,7). The graph of a seconds line passes through the points (0,0)and(−1,3). If the two lines intersect at the point (r,s), what is the value of r+s?
View Solution