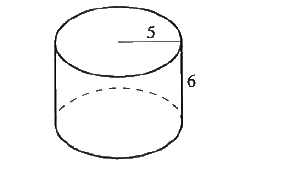
The volume of right circular cylinder A is 22 cubic centimeters. What is the volume , in cubic centimetres, of a right circular cylinder with twice the radius and half the height of cylinder A?
11
22
44
66
To solve the problem, we will follow these steps:
1. Understand the Volume Formula: The volume V of a right circular cylinder is given by the formula:
V=πr2h
where r is the radius and h is the height.
2. Set Up the Volume of Cylinder A: We know that the volume of cylinder A is 22 cubic centimeters. Therefore, we can write:
πr2h=22
3. Determine the Dimensions of the New Cylinder: The new cylinder has:
- Radius r1=2r (twice the radius of cylinder A)
- Height h1=h2 (half the height of cylinder A)
4. Calculate the Volume of the New Cylinder: The volume V1 of the new cylinder can be calculated using the formula:
V1=πr21h1
Substituting the values of r1 and h1:
V1=π(2r)2(h2)
5. Simplify the Expression: Now, simplify the expression:
V1=π(4r2)(h2)
V1=π⋅4r2⋅h2=2πr2h
6. Relate to the Volume of Cylinder A: We know from cylinder A that πr2h=22. Therefore:
V1=2⋅22=44 cubic centimeters
Thus, the volume of the new cylinder is 44 cubic centimeters.
V=πr2h
where r is the radius and h is the height.
πr2h=22
- Radius r1=2r (twice the radius of cylinder A)
- Height h1=h2 (half the height of cylinder A)
V1=πr21h1
Substituting the values of r1 and h1:
V1=π(2r)2(h2)
V1=π(4r2)(h2)
V1=π⋅4r2⋅h2=2πr2h
V1=2⋅22=44 cubic centimeters
Similar Questions
Knowledge Check
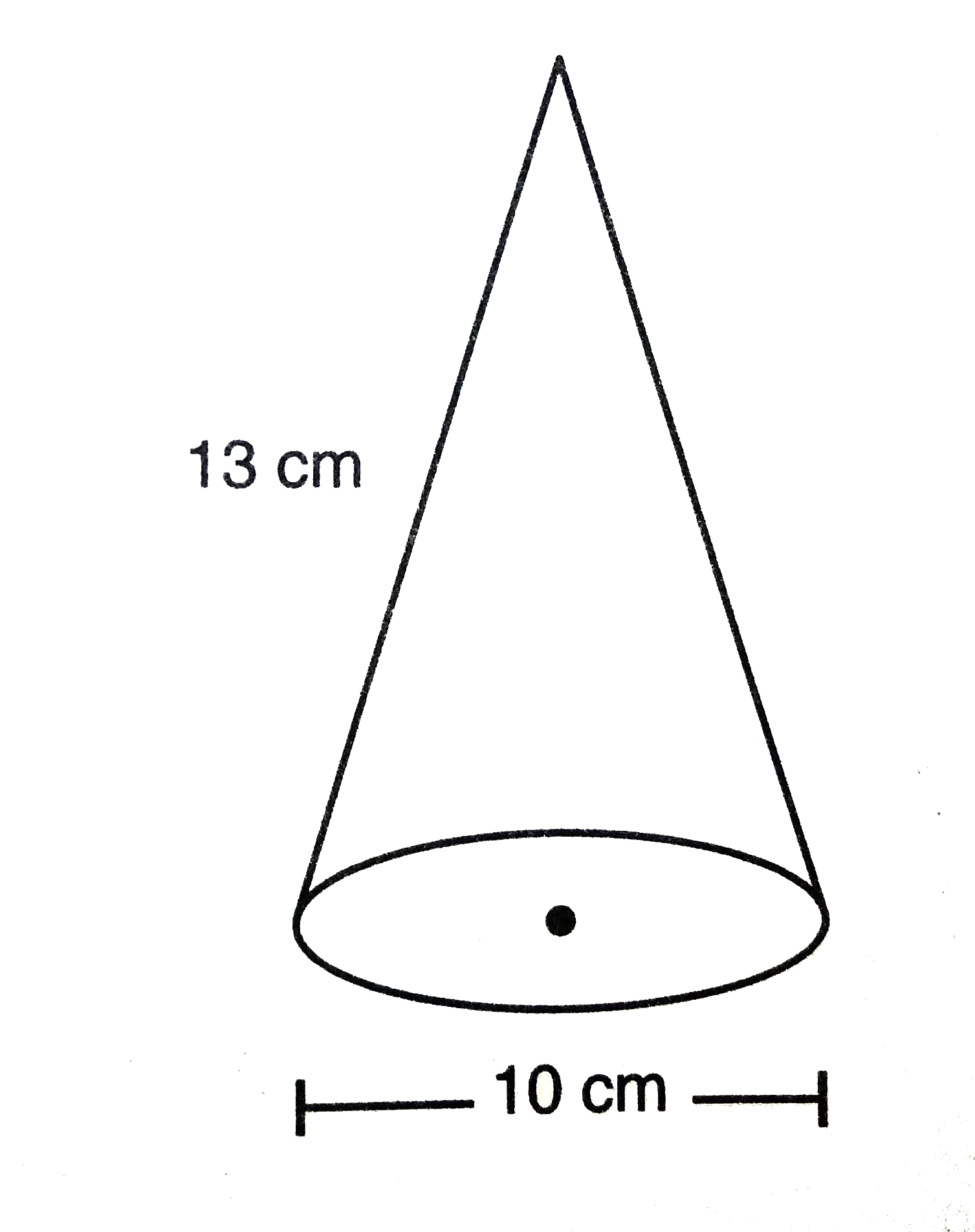
The height and radius of the right circular cylinder below are given in meters. What is the volume, in cubic meters, of the cylinder?
 A30πB31πC150πD180π
A30πB31πC150πD180πThe greatest possible distance between any two points on the surface of a right circular cylinder is √193 and the area of the circular base of the right circular cylinder is 36π. What is the volume of the right circular cylinder ?
A252πB294πC343πD386π
An oil tank has the shape of a right circular cylinder is 12 centimeters in height and has a volume of 108π cubic centimeters. What is the diameter of the base of the cylinder, in centimeters?
View SolutionThe diameter of the base of a right circular cylinder is 42cm and its height is 10cm. Find the volume of the cylinder.
View SolutionFind the volume of a right circular cylinder, if the radius (r) of its base and height (h) are 7cm and 15cm respectively.
View SolutionFind the volume of a right circular cylinder, if the radius (r) of its base and height (h) are 7cm and 15cm respectively.
View SolutionThe height of a right circular cylinder is 10.5. Three times the sum of the areas of its two circular faces is twice the area of the curved surface. Find the volume of the cylinder.
View SolutionThe height of a right circular cylinder is 10.5 m. Three times the sum of the area of its two circular faces is twice the area of the curved surface. Find the volume of the cylinder.
View Solution