The velocity of a projectile at the initial point A is (2ˆi+3ˆj)ms−1. Its velocity (in ms−1) at point B is
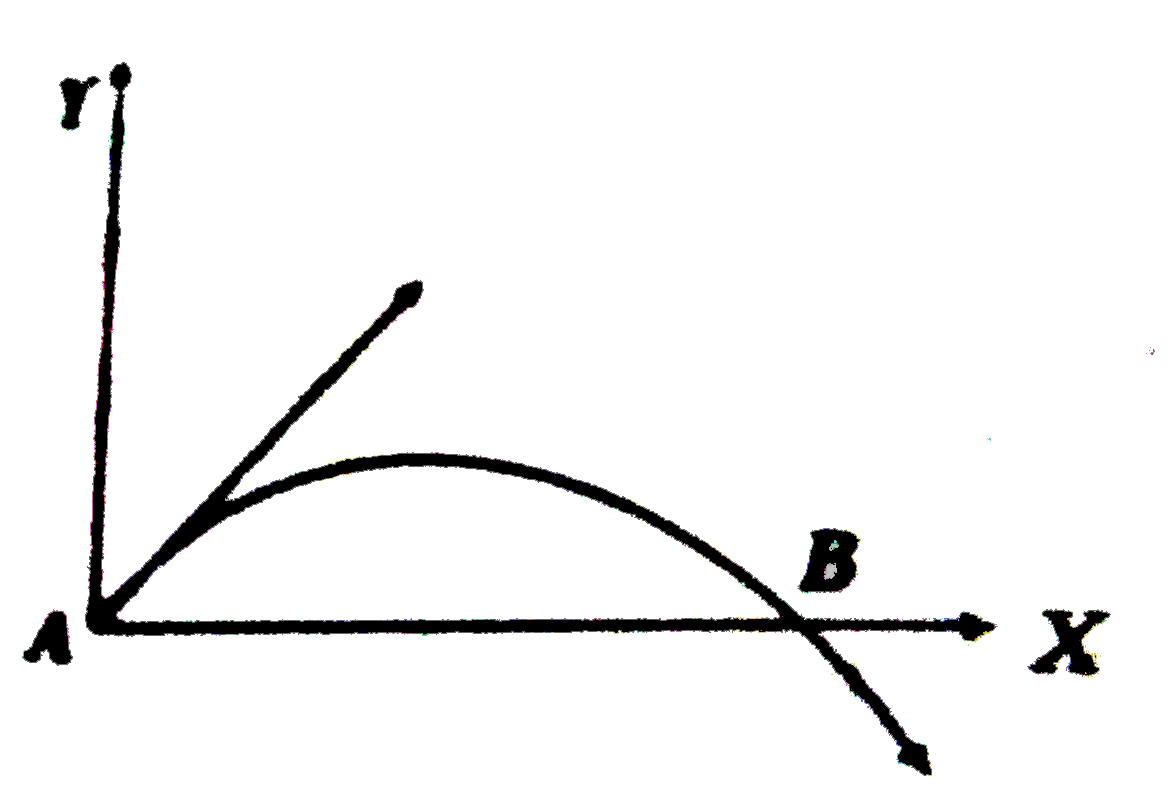
A
2ˆi−3ˆj
B
−2ˆi+3ˆj
C
2ˆi−3ˆj
D
2ˆi+3ˆj
Video Solution
Text Solution
The correct Answer is:C
From the question's figure, the X-component remain unchanged, while the Y-component is reverse. Then, the velocity at point B is (2ˆi−3ˆj)ms−1.
|
Topper's Solved these Questions
Similar Questions
Knowledge Check
The velocity of a projectile at the initial point A is (2ˆi+3ˆj)m/s. Its velocity (in m/s) at point B is
 A−2ˆi−3ˆjB−2ˆi+3ˆjC2ˆi−3ˆjD2ˆi+3ˆj
A−2ˆi−3ˆjB−2ˆi+3ˆjC2ˆi−3ˆjD2ˆi+3ˆjThe velocity of a projectile at the initial point A is (2ˆi+3ˆj)m/s.Its velocity (in m//s) at point B is
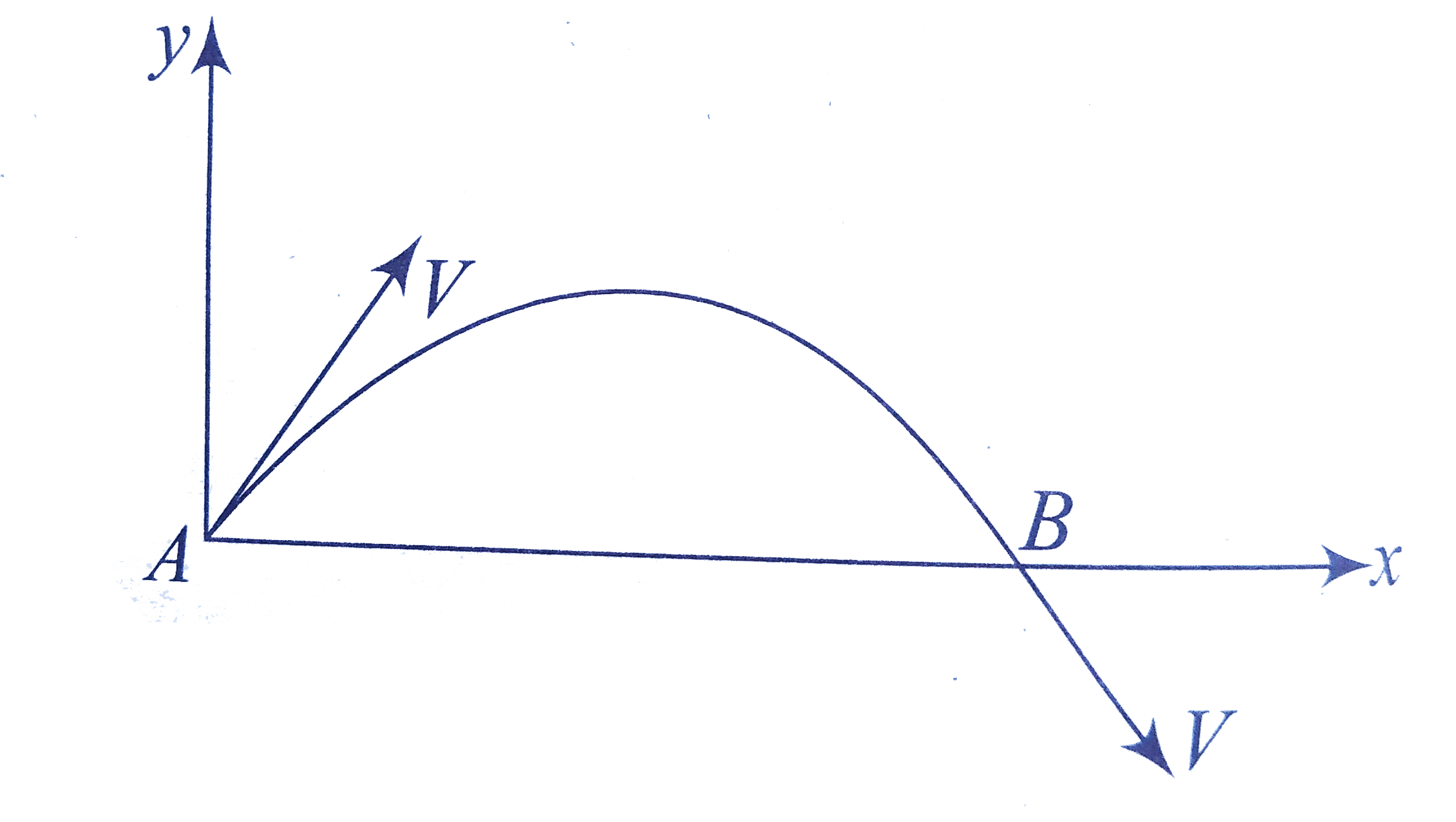 A−2ˆi−3ˆjB−2ˆi+3ˆjC2ˆi−3ˆjD2ˆi+3ˆj
A−2ˆi−3ˆjB−2ˆi+3ˆjC2ˆi−3ˆjD2ˆi+3ˆjThe velocity of a projectile at the initial point A is (2ˆi+3ˆj)m/s. Its velocity (in m/s) at point B is
 A−2ˆi−3ˆjB−2ˆi+3ˆjC2ˆi−3ˆjD2ˆi+3ˆj
A−2ˆi−3ˆjB−2ˆi+3ˆjC2ˆi−3ˆjD2ˆi+3ˆj
The velocity of a projectile at the initial point A is (2ˆi+3ˆj)m/s.Its velocity (in m//s) at point B is

View SolutionThe rms velocity of an ideal gas at 27∘C is 0.3ms−1. Its rms velocity at 927∘C (in ms−1) is:
View SolutionThe rms velocity of an ideal gas at 27∘C is 0.3ms−1. Its rms velocity at 927∘C (in ms−1) is:
View SolutionAssertion At height 20 m from ground , velocity of a projectile is v=(20ˆi+10ˆj)ms−1. Here, ˆi is horizontal and ˆj is vertical. Then, the particle is at the same height after 4s.
Reason Maximum height of particle from ground is 40m (take, g=10ms−2)
View SolutionA projectile is thrown with an initial velocity of (aˆi+ˆj)ms−1. If the range of the projectile is twice the maximum height reached by it, then
View Solution
