A particle moves in a circular path of radius R with an angualr velocity ω=a−bt, where a and b are positive constants and t is time. The magnitude of the acceleration of the particle after time 2ab is
aR
a2R
R(a2+b)
R√a4+b2
The correct Answer is:D
To solve the problem, we need to find the magnitude of the acceleration of a particle moving in a circular path with a given angular velocity after a specific time. Let's break this down step by step.
Step 1: Understand the given parameters
The particle is moving in a circular path of radius R with an angular velocity defined as:
ω=a−bt
where a and b are positive constants, and t is time.
Step 2: Calculate the angular velocity at time t=2ab
Substituting t=2ab into the angular velocity equation:
ω=a−b(2ab)=a−2a=−a
Thus, at t=2ab, the angular velocity ω is −a.
Step 3: Calculate centripetal acceleration AC
Centripetal acceleration is given by the formula:
AC=ω2R
Substituting ω=−a:
AC=(−a)2R=a2R
Step 4: Calculate angular acceleration α
Angular acceleration is defined as the time derivative of angular velocity:
α=dωdt
Differentiating ω=a−bt:
α=0−b=−b
Step 5: Calculate tangential acceleration AT
Tangential acceleration is given by:
AT=αR
Substituting α=−b:
AT=−bR
Step 6: Calculate the total acceleration
The total acceleration A is the vector sum of centripetal and tangential accelerations. Since these two accelerations are perpendicular to each other, we can use the Pythagorean theorem:
A=√A2T+A2C
Substituting the values we calculated:
A=√(−bR)2+(a2R)2
A=√b2R2+a4R2
Factoring out R2:
A=R√b2+a4
Final Answer
The magnitude of the acceleration of the particle after time 2ab is:
A=R√b2+a4
The particle is moving in a circular path of radius R with an angular velocity defined as:
ω=a−bt
where a and b are positive constants, and t is time.
Substituting t=2ab into the angular velocity equation:
ω=a−b(2ab)=a−2a=−a
Thus, at t=2ab, the angular velocity ω is −a.
Centripetal acceleration is given by the formula:
AC=ω2R
Substituting ω=−a:
AC=(−a)2R=a2R
Angular acceleration is defined as the time derivative of angular velocity:
α=dωdt
Differentiating ω=a−bt:
α=0−b=−b
Tangential acceleration is given by:
AT=αR
Substituting α=−b:
AT=−bR
The total acceleration A is the vector sum of centripetal and tangential accelerations. Since these two accelerations are perpendicular to each other, we can use the Pythagorean theorem:
A=√A2T+A2C
Substituting the values we calculated:
A=√(−bR)2+(a2R)2
A=√b2R2+a4R2
Factoring out R2:
A=R√b2+a4
The magnitude of the acceleration of the particle after time 2ab is:
A=R√b2+a4
Topper's Solved these Questions
- BOOK - DC PANDEYCHAPTER - CIRCULAR MOTIONEXERCISE - Taking it together67videos
CIRCULAR MOTION
- BOOK - DC PANDEYCHAPTER - CIRCULAR MOTIONEXERCISE - Match the columns3videos
CIRCULAR MOTION
- BOOK - DC PANDEYCHAPTER - CIRCULAR MOTIONEXERCISE - Integer7videos
CIRCULAR MOTION
- BOOK - DC PANDEYCHAPTER - CENTRE OF MASS, LINEAR MOMENTUM AND COLLISIONEXERCISE - Level 2 Subjective21videos
CENTRE OF MASS, LINEAR MOMENTUM AND COLLISION
- BOOK - DC PANDEYCHAPTER - COMMUNICATION SYSTEMEXERCISE - Only One Option is Correct27videos
COMMUNICATION SYSTEM
Similar Questions
The linear speed of a particle moving in a circle of radius R varies with time as v=v0−kt, where k is a positive constant. At what time the magnitudes of angular velocity and angular acceleration will be equal ?
View SolutionA particle moves from A to B in a circular path of radius R covering an angle θ as shown in figure. Find the ratio of magnitude of distance and magnitude of displacement of the particle.
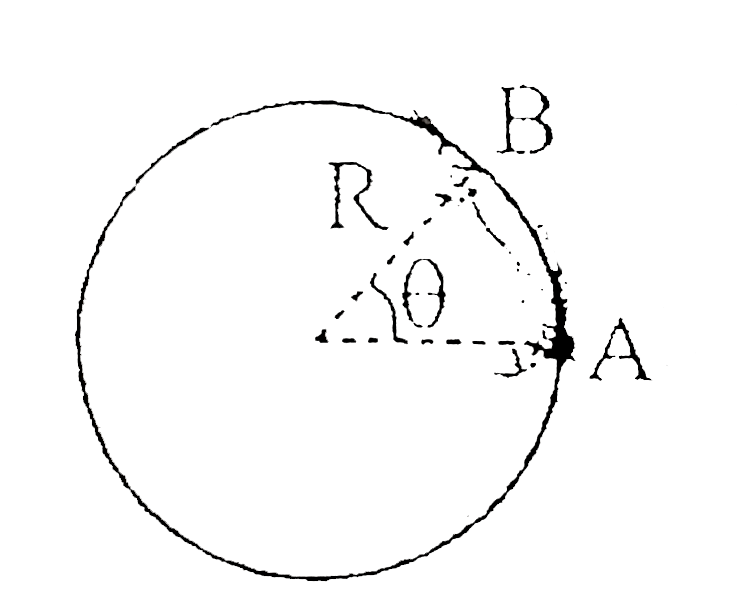 View Solution
View Solution
Knowledge Check
- Question 1 - Select One
A particle moving along a circule path of radius 'r' with uniform angular velocity ω. Its angular acceleration is
Arω2Br/ωCzeroDω/r - Question 2 - Select One
A particle moving in a circular path of radius (r) with velocity (V), Then centripetal acceleration of the particle is
AV2rBrV2CVrDV2r - Question 3 - Select One
A particle moving in a circular path of radius (r) with velocity (V), Then centripetal acceleration of the particle is
AV2rBrV2CVrDV2r
A particle of mass m is moving in a circle of radius r. The centripetal acceleration (ac) of the particle varies with the time according to the relation, ac=Kt2, where K is a positive constant and t is the time. The magnitude of the time rate of change of angular momentum of the particle about the centre of the circle is
View SolutionA particle of mass m moves in a circular path of radius r, under the action of force which delivers it constant power p and increases its speed. The angular acceleration of particle at time (t) is proportional
View SolutionThe velocity varies with time as →v=aˆi+btˆj , where a and b are positive constants. The magnitude of instantaneous velocity and acceleration would be
View SolutionA particle moves along a circle of radius R with a constant angular speed ω. Its displacement (only magnitude) in time t will be
View SolutionA particle moves along a circle of radius R with a constant angular speed ω . Its displacement (only magnitude) in time t will be
View Solution

