Consider a satellite orbiting the earth as shown in the figure below. Let LaandLp represent the angular momentum of the satellite about the earth when at aphelion and perihelion respectively. Consider the following relations
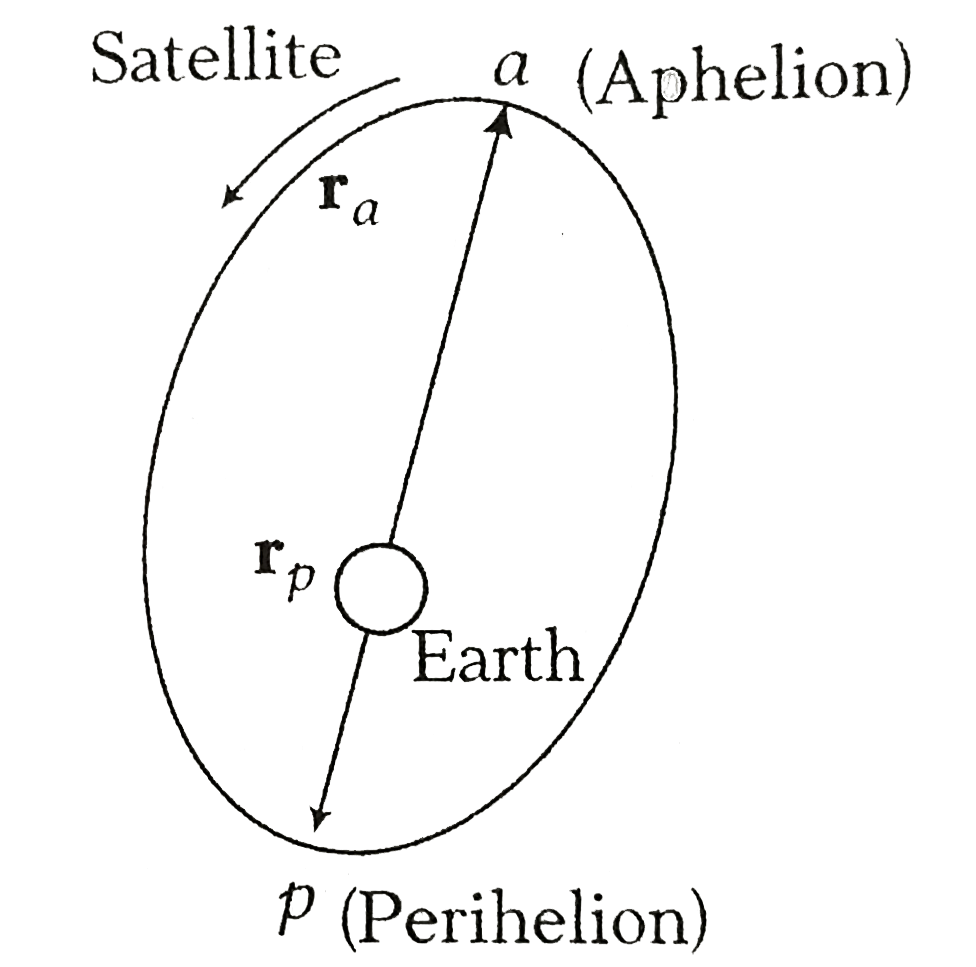
(i) La=Lp
(ii) La=−Lp
(iii) ra×La=rp×Lp
Which of the above relation(s) is/are true ?
(i) only
(ii) only
(iii) only
(i) and (iii)
The correct Answer is:A
Using the law of conservation of angular momentum.
Angular momentum of planet of p = Angular momentum of planet at a
i.e., Lp=La
But ra>rp. So, ra×La>rp'×Lp.
Topper's Solved these Questions
Similar Questions
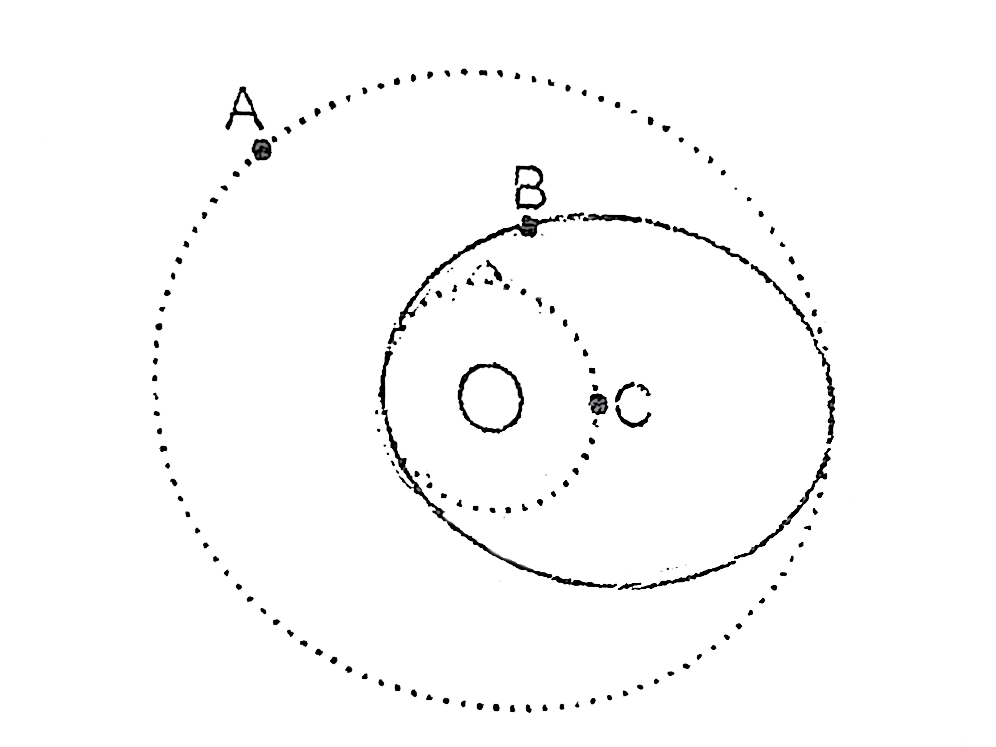
Three equal mass satellites A,B and C are in coplaner orbits around a planet as shown in the figure. The magnitudes of the angular momentum of the satellites as measured about the planet are LA,LB, and LC. Which of the following statements is correct?
 View Solution
View SolutionA satellite of mass m moving around the earth of mass mE in a circular orbit of radius R has angular momentum L. The rate of the area swept by the line joining the centre of the earth and satellite is
View Solution
Knowledge Check
- Question 1 - Select One
If L is the angular momentum of a satellite revolving around earth is a circular orbit of radius r with speed v , then
AL∝vBL∝rCL∝√rDL∝√v - Question 2 - Select One
The curve between loge L and loge P is (L is the angular momentum and P is the linear momentum).
A B
B C
C D
D
- Question 3 - Select One
The angular momentum L, the linear momentum P and position vector 'r' are related as
A→L=→r×→PBL=p/rC→L=→P×→rDL = p.r
A particle with position vector has a linear momentum p. Which of the following statements is true in respect of its angular momentum L about the origin?
View SolutionAngular momentum L is given by L=Pr The variation of log L and logP is correctly shown in Fig.
View SolutionA particle with the position vector r has linear momentum p. Which of the following statements is true is respect of its angular momentum L about the origin ?
View SolutionA spherical body of radius R rolls on a horizontal surface with linear velociltly v. Let L1 and L2 be the magnitudes of angular momenta of the body about centre of mass and point of contact P. Then:
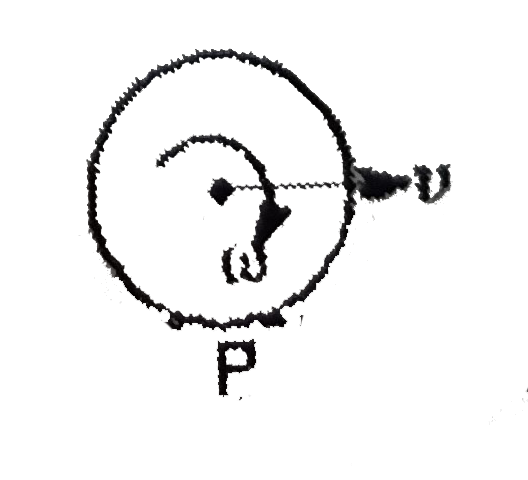 View Solution
View SolutionIf a moving particle have linear momentum →P and position vector →r then choose the correct relation between →→p and angular momentum →L of particle about the origin.
View Solution

