A straight wire of length 30 cm and mass 60 mg lies in a direction 30∘ east of north.The earth's magnetic field at this is horizontal and has a magnitude of 0.8 G.What current must bre passed through the wire,so that it may float in air ?
To solve the problem of determining the current required for a straight wire to float in the air when placed in a magnetic field, we can follow these steps:
Step 1: Understand the Forces Acting on the Wire
The wire will float in the air when the magnetic force acting on it is equal to its weight. The weight (W) of the wire can be calculated using the formula:
W=mg
where:
- m=60mg=60×10−6kg
- g=10m/s2 (acceleration due to gravity)
Step 2: Calculate the Weight of the Wire
Substituting the values into the weight formula:
W=(60×10−6kg)×(10m/s2)=6×10−5N
Step 3: Determine the Magnetic Force
The magnetic force (F) acting on the wire can be expressed as:
F=ILBsinθ
where:
- I is the current through the wire,
- L=30cm=0.3m (length of the wire),
- B=0.8G=0.8×10−4T (magnetic field strength),
- θ=30∘ (angle between the wire and the magnetic field).
Step 4: Set the Forces Equal
For the wire to float, we set the weight equal to the magnetic force:
mg=ILBsinθ
Step 5: Rearrange the Equation to Solve for Current (I)
Rearranging the equation gives:
I=mgLBsinθ
Step 6: Substitute the Known Values
Now we substitute the known values into the equation:
- m=60×10−6kg
- g=10m/s2
- L=0.3m
- B=0.8×10−4T
- sin30∘=12
Substituting these values:
I=(60×10−6kg)×(10m/s2)(0.3m)×(0.8×10−4T)×(12)
Step 7: Calculate the Current
Calculating the numerator:
60×10−6×10=6×10−5N
Calculating the denominator:
0.3×0.8×10−4×12=0.3×0.4×10−4=0.12×10−4=1.2×10−5
Now substituting back into the equation for current:
I=6×10−51.2×10−5=5A
Conclusion
The current that must be passed through the wire for it to float in the air is:
I=50A
The wire will float in the air when the magnetic force acting on it is equal to its weight. The weight (W) of the wire can be calculated using the formula:
W=mg
where:
- m=60mg=60×10−6kg
- g=10m/s2 (acceleration due to gravity)
Substituting the values into the weight formula:
W=(60×10−6kg)×(10m/s2)=6×10−5N
The magnetic force (F) acting on the wire can be expressed as:
F=ILBsinθ
where:
- I is the current through the wire,
- L=30cm=0.3m (length of the wire),
- B=0.8G=0.8×10−4T (magnetic field strength),
- θ=30∘ (angle between the wire and the magnetic field).
For the wire to float, we set the weight equal to the magnetic force:
mg=ILBsinθ
Rearranging the equation gives:
I=mgLBsinθ
Now we substitute the known values into the equation:
- m=60×10−6kg
- g=10m/s2
- L=0.3m
- B=0.8×10−4T
- sin30∘=12
I=(60×10−6kg)×(10m/s2)(0.3m)×(0.8×10−4T)×(12)
Calculating the numerator:
60×10−6×10=6×10−5N
0.3×0.8×10−4×12=0.3×0.4×10−4=0.12×10−4=1.2×10−5
I=6×10−51.2×10−5=5A
The current that must be passed through the wire for it to float in the air is:
I=50A
Topper's Solved these Questions
- BOOK - DC PANDEYCHAPTER - MAGNETIC FIELD AND FORCESEXERCISE - Check point54videos
MAGNETIC FIELD AND FORCES
- BOOK - DC PANDEYCHAPTER - MAGNETIC FIELD AND FORCESEXERCISE - Taking it together75videos
MAGNETIC FIELD AND FORCES
- BOOK - DC PANDEYCHAPTER - INTERFERENCE AND DIFFRACTION OF LIGHTEXERCISE - Level 2 Subjective5videos
INTERFERENCE AND DIFFRACTION OF LIGHT
- BOOK - DC PANDEYCHAPTER - MAGNETICSEXERCISE - MCQ_TYPE1videos
MAGNETICS
Similar Questions
A straight wire of mass 400 g and length 2m carries a current of 2A. It is suspended in mid air by a uniform horizontal field B. What is the magnitude of the magnetic field ?
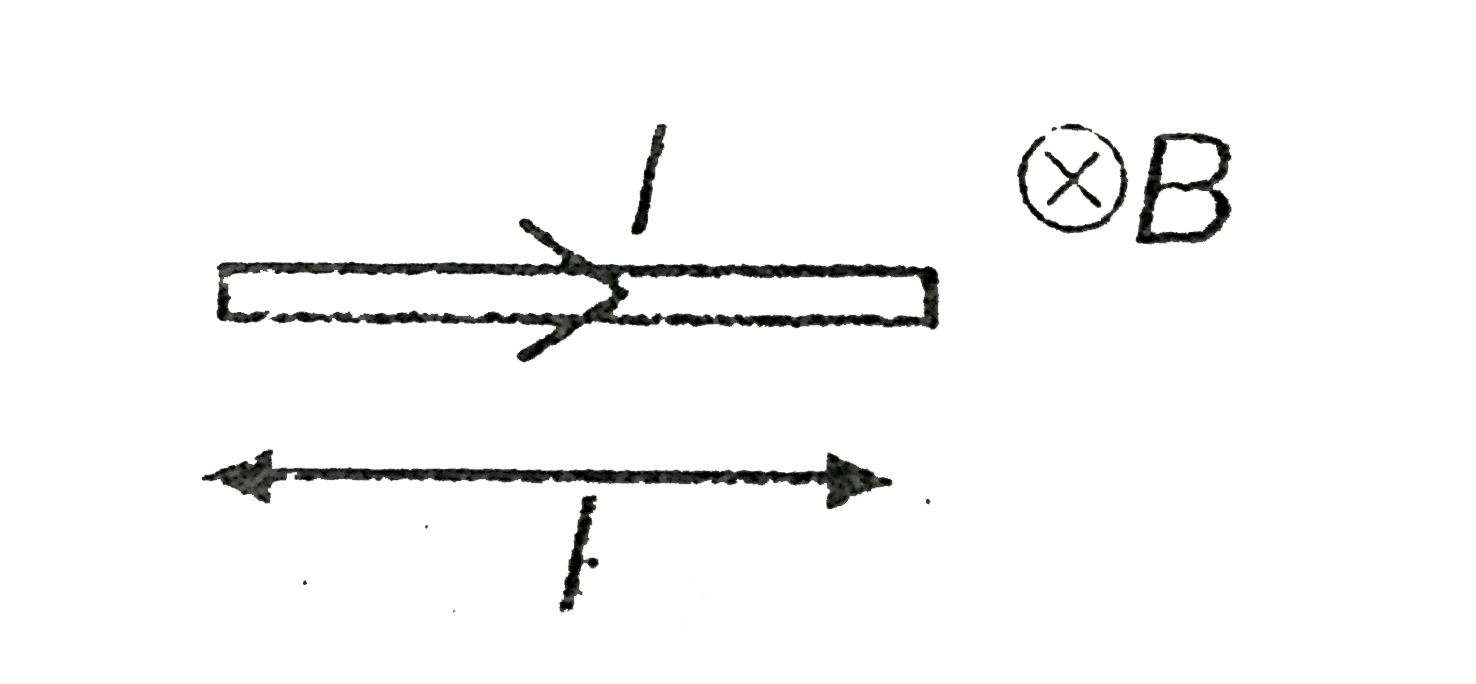 View Solution
View SolutionA straight wire of mass 200 g and length 1.5 m carries a current of 2 A. It is suspended in mid-air by a uniform horizontal magnetic field B. What is the magnitude of the magnetic field?
 View Solution
View Solution
Knowledge Check
- Question 1 - Select One
A fixed horizontal wire carries a current of 200 A. Another wire having a mass per unit length 10−2 kg/m is placed below the first wire at a distance of 2 cm and parallel to it. How much current must be passed through the second wire if it floats in air without any support? What should be the direction of current in it?
A25 A (direction of current is same to first wire)B25 A (direction of current is opposite to first wire)C49 A (direction of current is same to first wire)D49 A (direction of current is opposite to first wire) - Question 2 - Select One
A straight wire having mass of 1.2 kg and length of 1m carries a current of 5 A. If the wire is suspended in mid-air by a uniform horizontal magnetic field, then the magnitude of field is
A0.65TB1.53TC2.4TD3.2T - Question 3 - Select One
A long straight wire carrying current of 30A is placed in an external uniform magnetic field of induction 4×104T. The magnetic field is acting parallel to the direction of current. The magnitude of the resultant magnetic inuduction in tesla at a point 2.0cm away form the wire is
A10−4B3×10−4C5×10−4D6×10−4
A straight wire of mass 200 g and length 1.5 m carries a current of 2 A. It is suspended in mid-air by a uniform horizontal magnetic field B. What is the magnitude of the magnetic field?
 View Solution
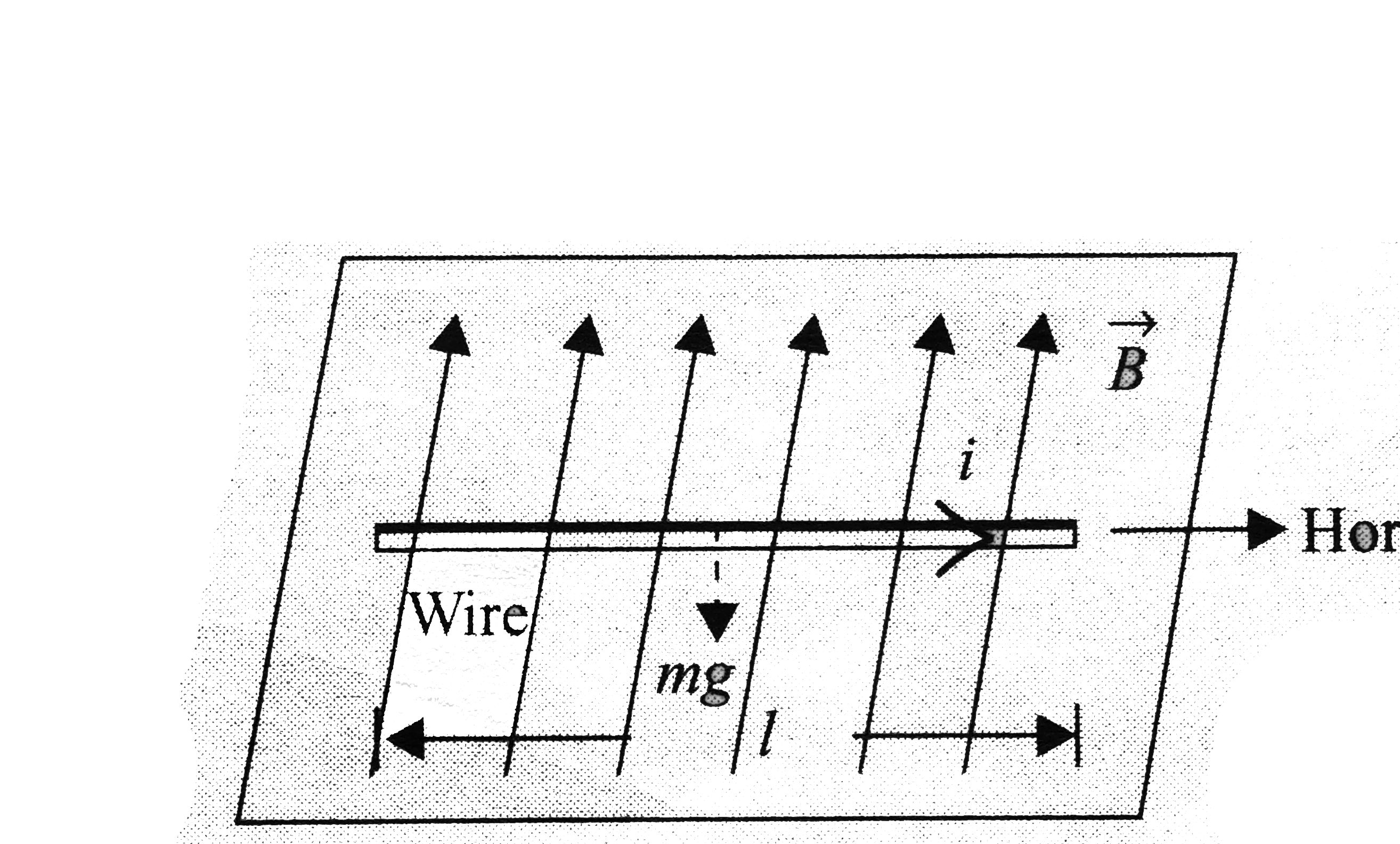
View SolutionA wire of 60 cm length and mass 16 gm is suspended by a pair of flexible leads in a magnetic field of induction 0.40 T. What are the magnitude and direction of the current required to remove the tension in the supporting leads?
 View Solution
View SolutionA current of 5.0 A exists in the circuit shown in The wire PQ has a length of 50 cm the magnetic field in which it is immersed has a magnitude of 0.20 T. Find the magnetic force acting on the wire PQ.
 View Solution
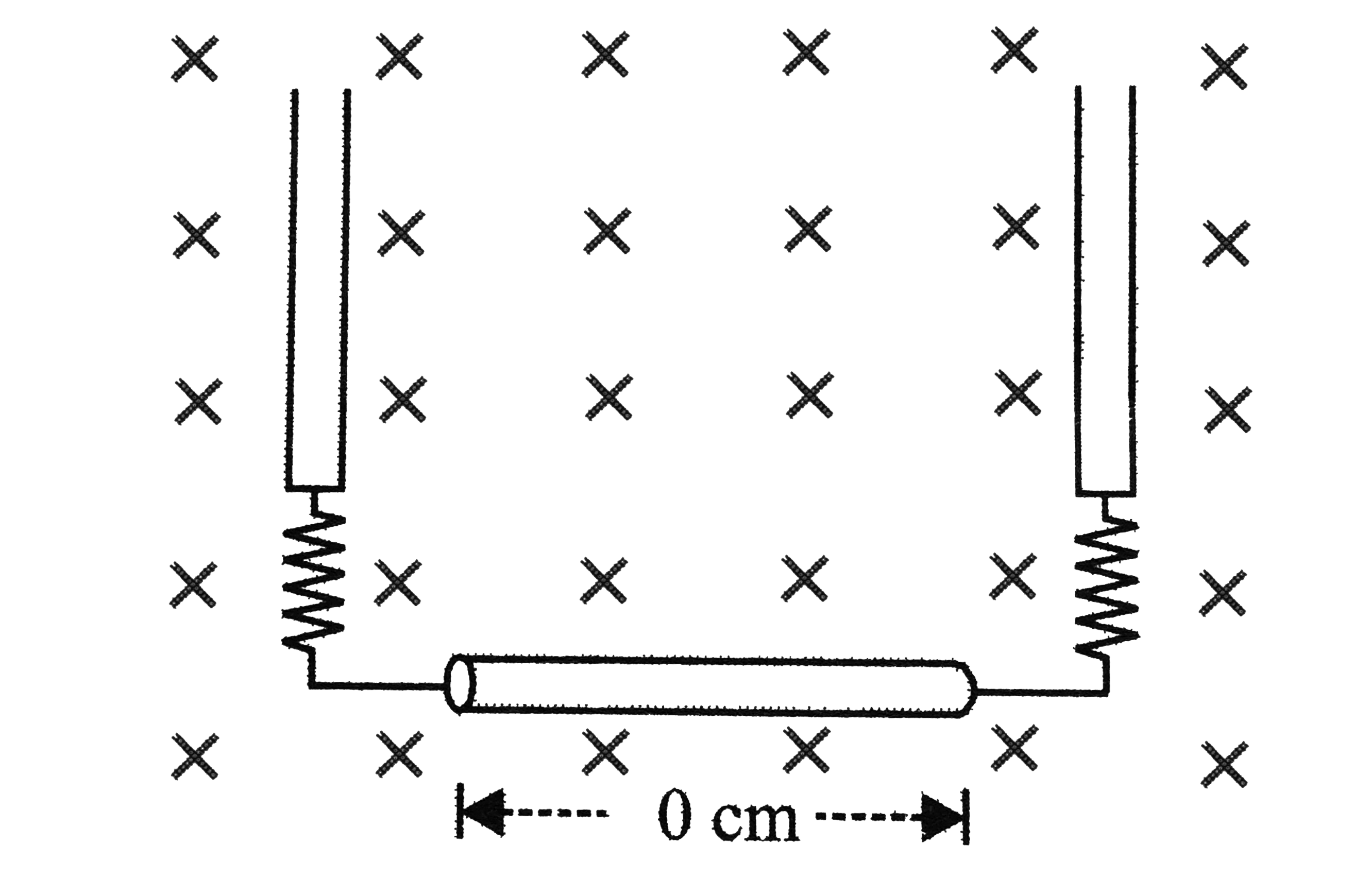
View SolutionA straight wire of length 50 cm carrying a current of 2.5 A is suspended in mid-air by a uniform magnetic field of 0.5 T (as shown in figure). The mass of the wire is (g = 10 ms−2)
 View Solution
View SolutionA straight horizontal conducting rod of length 50 cm and mass 50 g is suspended by two vertical wires at its ends. A current of 5.0 A is set up in the rod through the wires. What magnetic field should be set up normal to the conductor in order that the tension in the wires is zero? (take=10ms−2
View Solution

