Converging light rays are falling on a convex lens. If the focal length of the lens is 30 cm, then find the position of the image.
To find the position of the image formed by a convex lens when converging light rays are incident on it, we can use the lens formula:
1V=1F+1U
Where:
- V is the image distance from the lens,
- F is the focal length of the lens,
- U is the object distance from the lens.
1. Identify the Given Values:
- Focal length F=30cm
- Object distance U=−10cm (the object distance is taken as negative in lens formula convention since the object is on the same side as the incoming light)
2. Substitute the Values into the Lens Formula:
1V=1F+1U
1V=130+1−10
3. Calculate the Right Side:
- Convert the fractions to a common denominator:
1V=130−330=1−330=−230
1V=−115
4. Find the Image Distance V:
- Take the reciprocal:
V=−15cm
5. Interpret the Result:
- The negative sign indicates that the image is formed on the same side as the object, meaning it is a virtual image.
Final Answer:
The position of the image is at 15cm on the same side as the object.
---
- V is the image distance from the lens,
- F is the focal length of the lens,
- U is the object distance from the lens.
- Focal length F=30cm
- Object distance U=−10cm (the object distance is taken as negative in lens formula convention since the object is on the same side as the incoming light)
1V=1F+1U
1V=130+1−10
- Convert the fractions to a common denominator:
1V=130−330=1−330=−230
1V=−115
- Take the reciprocal:
V=−15cm
- The negative sign indicates that the image is formed on the same side as the object, meaning it is a virtual image.
The position of the image is at 15cm on the same side as the object.
Topper's Solved these Questions
- BOOK - SL ARORACHAPTER - RAY OPTICSEXERCISE - TYPE J11videos
RAY OPTICS
- BOOK - SL ARORACHAPTER - RAY OPTICSEXERCISE - Problems26videos
RAY OPTICS
- BOOK - SL ARORACHAPTER - RAY OPTICSEXERCISE - TYPE G19videos
RAY OPTICS
- BOOK - SL ARORACHAPTER - OPTICAL INSTRUMENTSEXERCISE - Problems for self practice35videos
OPTICAL INSTRUMENTS
- BOOK - SL ARORACHAPTER - SOLIDS AND SEMICONDUCTOR DEVICESEXERCISE - PROBLEM_TYPE9videos
SOLIDS AND SEMICONDUCTOR DEVICES
Similar Questions
Rays of light are falling on a convex lens of focal length 40cm. As shown in Fig. Determine the position of the image.
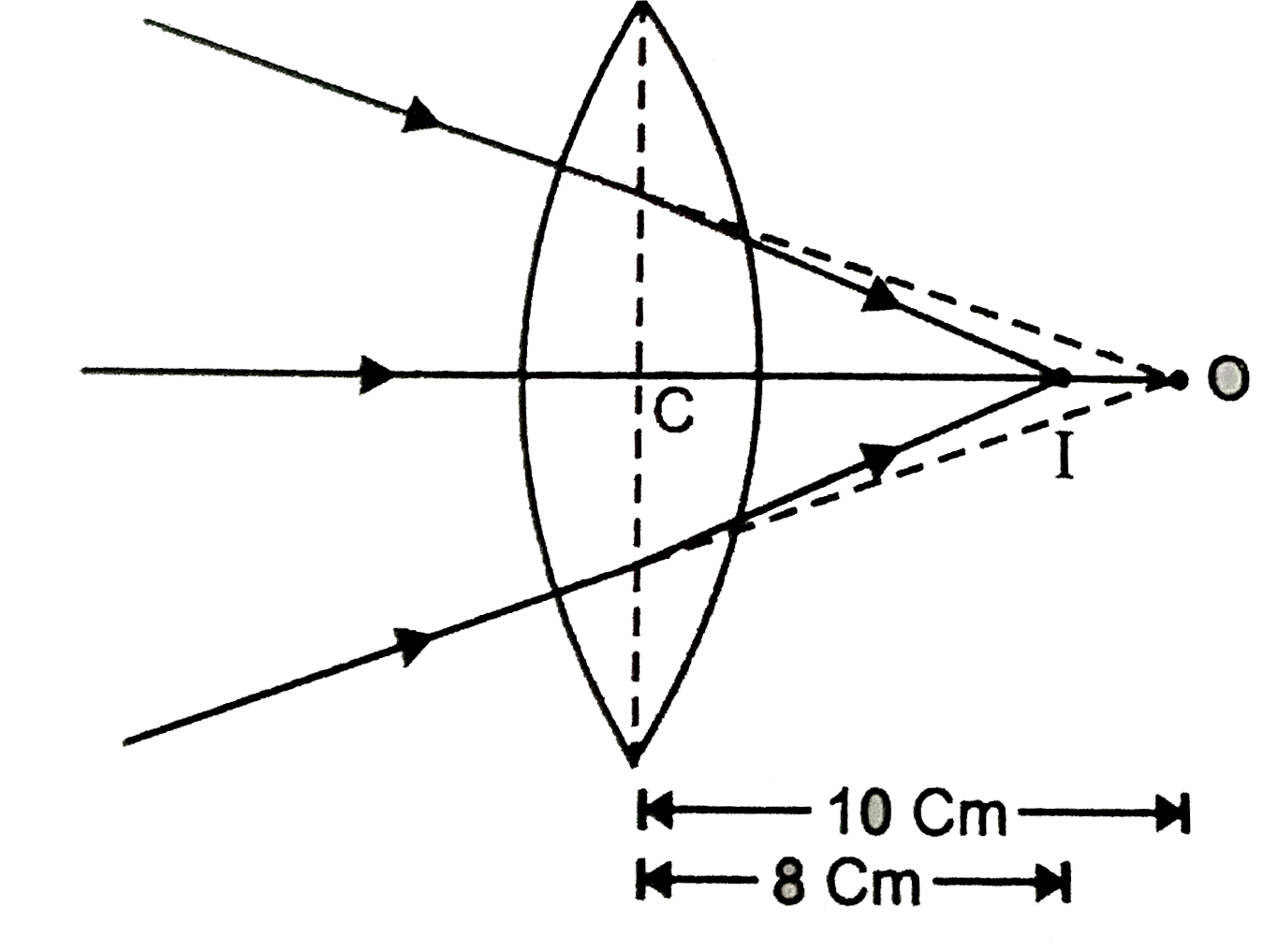 .View Solution
.View SolutionA pin of length 2.0 cm lies along the principal axis of a converging lens, the centre being at a distance of 11 cm from the lens. The focal length of the lens is 6 cm. Find the size of the image.
View Solution
Knowledge Check
- Question 1 - Select One
If in a plano-convex lens, the radius curvature of the convex surface is 10 cm and the focal length of the lens is 30 cm, then the refractive index of the material of lens will be
A1.5B1.66C1.33D3
A pin of length 1cm lies along the principle axis of a converging lens, the centre being at a distance of 5,5cm form the lens. The focal length of the lens is 3cm. Find the size of the image.
View SolutionAn object is placed at a distance of 30 cm from a convex lens of focal length 20 cm.Find the position of the image .
View SolutionFocal length of a convex lens in air is 20cm. and of concave lens 40cm. Find the position of final image.
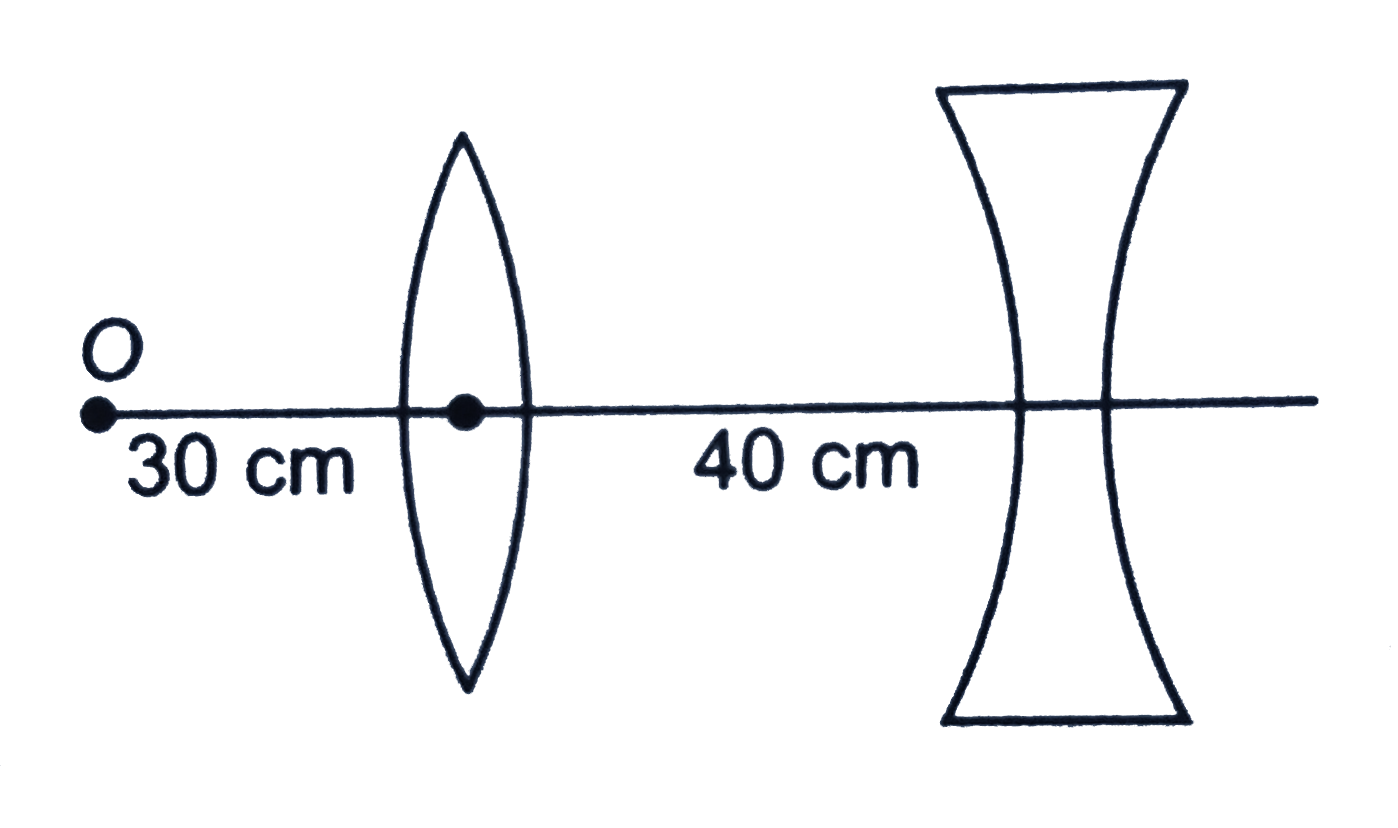 View Solution
View SolutionA lens produces a magnification of -0.5. Is this a converging or diverging lens? If the focal length of the lens is 6 cm, draw a ray diagram showing the image formation in this case.
View SolutionA lens produces a magnification of - 0.5. Is this a converging or diverging lens ? If the focal length of the lens is 6 cm, draw a ray diagram showing the image formation in this case.
View SolutionAn object is placed at 10 cm from a convex lens of focal length 12 cm. Find the position, nature and magnification of the image.
View SolutionAn object is placed at 10 cm from a convex lens of focal length 12 cm. Find the position, nature and magnification of the image.
View Solution

