If two parallel chords of a circle, having diameter 4 units, lie on the opposite sides of the centreand subtend angles ${cos}^{- 1} (\frac{1}{7}) and {sec}^{- 1} (7)$ at the centre respectively, then the distance betweenthese chords, is: (a) $\frac{4}{\sqrt{7}}$ (b) $\frac{8}{\sqrt{7}}$ (c) $\frac{8}{7}$ (d) $\frac{16}{7}$

Video Solution

Answer

Step by step video & image solution for If two parallel chords of a circle, having diameter 4 units, lie on the opposite sides of the centreand subtend angles cos^-1(1/7) and sec^-1(7) at the centre respectively, then the distance betweenthese chords, is: (a) 4/sqrt7 (b) 8/sqrt7 (c) 8/7 (d) 16/7 by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.

Updated on:21/07/2023

Class 12 MATHS DEFAULT

Knowledge Check

In a circle, there are two chords opposite to the centre of the circle. The length of one chord and the radius of the circle is 6 cm and 5 cm respectively, and the distance between both the chords is 7 cm. Find the length of the second chord.
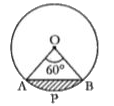
Aa) 8 cm
Bb) 10 cm
Cc) 6 cm
Dd) 14 cm
A chord of length 7 cm subtends an angle of $60^{\circ}$ at the centre of a circle. What is the radius (in cm) of the circle?
A $7 \sqrt{2}$
B $7 \sqrt{3}$
C $7$
D $14$
A chord of length 7 cm subtends an angle of $60^{\circ}$ at the centre of a circle. What is the radius (in cm) of the circle?
A $7 \sqrt{2}$
B $7 \sqrt{3}$
C $7$
D $14$

Similar Questions

Knowledge Check

In a circle, there are two chords opposite to the centre of the circle. The length of one chord and the radius of the circle is 6 cm and 5 cm respectively, and the distance between both the chords is 7 cm. Find the length of the second chord.

A chord of length 7 cm subtends an angle of $60^{\circ}$ at the centre of a circle. What is the radius (in cm) of the circle?

A chord of length 7 cm subtends an angle of $60^{\circ}$ at the centre of a circle. What is the radius (in cm) of the circle?

Recommended Questions

If two parallel chords of a circle, having diameter 4 units, lie on the opposite sides of the centreand subtend angles cos−1(17)andsec−1(7) at the centre respectively, then the distance betweenthese chords, is: (a) 4√7 (b) 8√7 (c) 87 (d) 167

Similar Questions

Knowledge Check

In a circle, there are two chords opposite to the centre of the circle. The length of one chord and the radius of the circle is 6 cm and 5 cm respectively, and the distance between both the chords is 7 cm. Find the length of the second chord.

A chord of length 7 cm subtends an angle of 60∘ at the centre of a circle. What is the radius (in cm) of the circle?

A chord of length 7 cm subtends an angle of 60∘ at the centre of a circle. What is the radius (in cm) of the circle?

Recommended Questions

A chord of length 7 cm subtends an angle of $60^{\circ}$ at the centre of a circle. What is the radius (in cm) of the circle?

A chord of length 7 cm subtends an angle of $60^{\circ}$ at the centre of a circle. What is the radius (in cm) of the circle?