A thin bi-convex lens made out of a material of refractive index $μ_{1}$ is place in a medium of refractive index $μ_{2}$ . A paraxial beam of light, parallel to the principal axis of the lens, is shown in the figure. Based on the ray diagram, we can conclude that 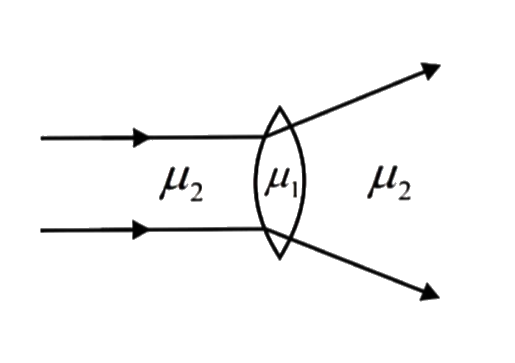$A thin bi-convex lens made out of a material of refractive index mu(1) is place in a medium of refractive index mu(2). A paraxial beam of light, parallel to the principal axis of the lens, is shown in the figure. Based on the ray diagram, we can conclude that$

$μ_{1} > μ_{2}$

$μ_{1} < μ_{2}$

$μ_{1} = μ_{2}$

$μ_{1} < μ_{2}$

Video Solution

Text Solution

Verified by Experts

The correct Answer is:D

Updated on:21/07/2023

Answer

Step by step video & image solution for A thin bi-convex lens made out of a material of refractive index mu_(1) is place in a medium of refractive index mu_(2). A paraxial beam of light, parallel to the principal axis of the lens, is shown in the figure. Based on the ray diagram, we can conclude that by Physics experts to help you in doubts & scoring excellent marks in Class 12 exams.

Updated on:21/07/2023

Class 12 PHYSICS QUESTION BANK

Topper's Solved these Questions

Knowledge Check

A lens made of material of Refractive index $μ_{2}$ is surrounded by a medium of Refractive Index $μ_{1}$ . The focal length f is related as
A $f \propto (μ_{2} - μ_{1})$
B $f \propto \frac{μ_{1}}{(μ_{2} - μ_{1})}$
C $f \propto (μ_{2} + μ_{1})$
D $f \propto \frac{1}{(μ_{2} + μ_{1})}$
A convex lens made up of material of refractive index $μ_{1}$ , is immersed in a medium of refractive index $μ_{2}$ as shown in the figure. The relation between $μ_{1}$ and $μ_{2}$ is

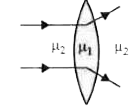A $μ_{1} < μ_{2}$
B $μ_{1} > μ_{2}$
C $μ_{1} = μ_{2}$
D $μ_{1} = \sqrt{μ_{2}}$
If a convex lens of refractive index $μ_{l}$ is placed in a medium of refractive index $μ_{m}$ Such that $μ_{l} > μ_{m} > μ_{a i r}$ then
AThe nature of the lens is diverging
BThe nature of the lens is converging
CThe nalure of the lens may be converging or diverging
DThe nature of the lens can't be determined

NTA MOCK TESTS-JEE MOCK TEST 15-MCQS (PHYSICS)

Home
Ask Doubt
Profile

A thin bi-convex lens made out of a material of refractive index μ1 is place in a medium of refractive index μ2. A paraxial beam of light, parallel to the principal axis of the lens, is shown in the figure. Based on the ray diagram, we can conclude that

The correct Answer is:D

Topper's Solved these Questions

Similar Questions

Knowledge Check

A lens made of material of Refractive index μ2 is surrounded by a medium of Refractive Index μ1. The focal length f is related as

A convex lens made up of material of refractive index μ1, is immersed in a medium of refractive index μ2 as shown in the figure. The relation between μ1 and μ2 is

If a convex lens of refractive index μl is placed in a medium of refractive index μm Such that μl>μm>μair then

NTA MOCK TESTS-JEE MOCK TEST 15-MCQS (PHYSICS)

A lens made of material of Refractive index $μ_{2}$ is surrounded by a medium of Refractive Index $μ_{1}$ . The focal length f is related as

A convex lens made up of material of refractive index $μ_{1}$ , is immersed in a medium of refractive index $μ_{2}$ as shown in the figure. The relation between $μ_{1}$ and $μ_{2}$ is

If a convex lens of refractive index $μ_{l}$ is placed in a medium of refractive index $μ_{m}$ Such that $μ_{l} > μ_{m} > μ_{a i r}$ then