The work done in carrying a charge q once round a circle of radius r with a charge Q at the centre is
qQ4πε0r
qQ4πε20r
qQ4πε0r2
none of the above
To solve the problem of finding the work done in carrying a charge q once around a circle of radius r with a charge Q at the center, we can follow these steps:
1. Understand the Setup: We have a charge Q located at the center of a circle, and we want to carry a charge q around this circle of radius r.
2. Electric Potential Due to Charge Q: The electric potential V at any point on the circle due to the charge Q is given by the formula:
V=kQr
where k is Coulomb's constant. This potential is constant at every point on the circle since the distance from the charge Q to any point on the circle is the same (equal to r).
3. Potential Difference: When moving the charge q around the circle, we need to consider the potential difference between any two points on the circle. Since the potential V is constant, the potential difference ΔV between any two points A and B on the circle is:
ΔV=VB−VA=0
4. Work Done Calculation: The work done W in moving a charge q in an electric field is given by:
W=qΔV
Since we established that ΔV=0, we can substitute this into the equation:
W=q⋅0=0
5. Conclusion: Therefore, the work done in carrying the charge q once around the circle is:
W=0
Final Answer:
The work done in carrying a charge q once round a circle of radius r with a charge Q at the center is 0.
---
V=kQr
where k is Coulomb's constant. This potential is constant at every point on the circle since the distance from the charge Q to any point on the circle is the same (equal to r).
ΔV=VB−VA=0
W=qΔV
Since we established that ΔV=0, we can substitute this into the equation:
W=q⋅0=0
W=0
The work done in carrying a charge q once round a circle of radius r with a charge Q at the center is 0.
Topper's Solved these Questions
- GAUSS LAW AND ELECTRIC POTENTIAL THEORYBook:BITSAT GUIDEChapter:GAUSS LAW AND ELECTRIC POTENTIAL THEORYExercise:BITSTAT ARCHIVES
Explore 4 Videos
- FLUID MECHANICSBook:BITSAT GUIDEChapter:FLUID MECHANICSExercise:BITSAT Achives
Explore 15 Videos
- MAGNETIC FIELDBook:BITSAT GUIDEChapter:MAGNETIC FIELDExercise:All Questions
Explore 52 Videos
Similar Questions
What is the amount of work done in moving a point charge q0 around a circular arc of radius 'r' at the centre of which another point charge q is located ?
View SolutionThree equal point charges of charge +q each are moving along a circle of radius R and a point charge -2q is also placed at the centre of circle (as shown in figure). If charge are revolving with constant and same speed in the circle then calculate speed of charges
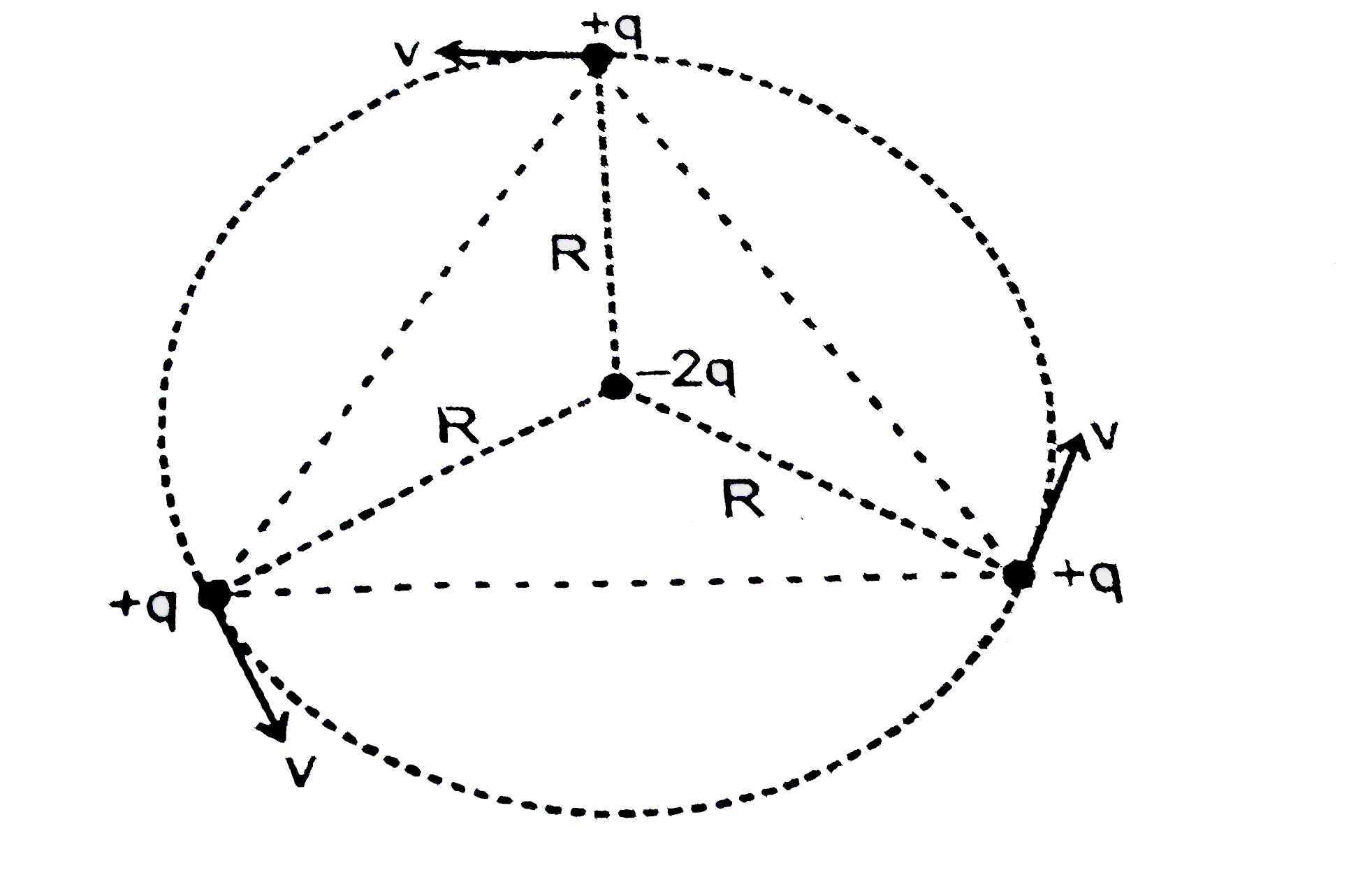
View Solution
Knowledge Check
The work done in carrying a charge q once around a circle of radius r with a charge Q placed at the centre centre will be
AQq4πε0r2BQq4πε0rCzeroDQq24πε0rThe work done in carrying a charge q once around a circle of radius r with a charge Q placed at the centre centre will be
AQq4πε0r2BQq4πε0rCzeroDQq24πε0rThe work done in carrying a charge Q1 once round a circle of radius R with a charge Q2 at the centre is
AQ1Q24π∈0R2BzeroCQ1Q24π∈0RDinfinite
A unit positive charge is moved along the circumfer-ence of a circle of radius, r with a −5μC charge at the centre at the centre of the circle. Then, the work done in the process is ______.
View SolutionIf a charge q is moving in a circle of radius r with speed v, then the equivalent current will be :
View SolutionRefer to the arrangement of charges in Fig and a Gaussian surface of radius R with Q at the centre. Then
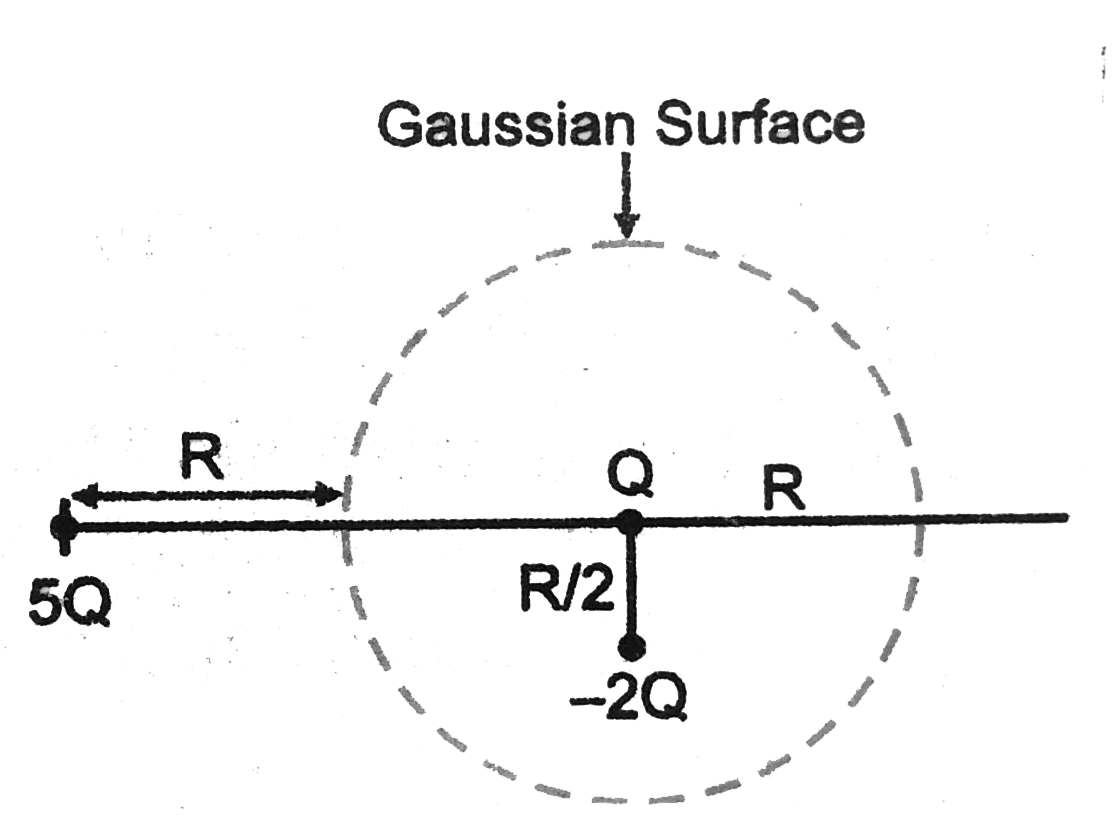
View SolutionOn rotating a point charge g around a charge Q in a circle of radius r, the work done is
View SolutionOn ortating a point charge having a charge q around a chrage Q in a circla of radius r. The work done will be
View Solution