From Ma3b3, when one b is replaced by c, the total no. of geometrical isomer possible are
The correct Answer is:3
Ma3b2c: No. of geometrical isomers 3 .
Topper's Solved these Questions
- COORDINATION COMPLEXESBOOK - NARAYNACHAPTER - COORDINATION COMPLEXESEXERCISE - FILL IN THE BLANKS
6 Videos
- COORDINATION COMPLEXESBOOK - NARAYNACHAPTER - COORDINATION COMPLEXESEXERCISE - SINGLE ANSWER QUESTIONS
29 Videos
- COORDINATION COMPLEXESBOOK - NARAYNACHAPTER - COORDINATION COMPLEXESEXERCISE - Statement Type
9 Videos
- CO-ORDINATE COMPOUNDS BOOK - NARAYNACHAPTER - CO-ORDINATE COMPOUNDS EXERCISE - Exercise-4
60 Videos
- D - BLOCK ELEMENTSBOOK - NARAYNACHAPTER - D - BLOCK ELEMENTSEXERCISE - CHECK YOUR GRASP
7 Videos
Similar Questions
Knowledge Check
If a complex, Ma4b2 (where M is a central atom and a and b are mondentate ligands ) has trigonal prismatic geometry, the total number of possible geometrical isomers would be :
A4B2C3D5If a complex, Ma4b2 (where M is a central atom and a and b are mondentate ligands ) has trigonal prismatic geometry, the total number of possible geometrical isomers would be :
A4B2C3D5A complex with the composition [MA3B]n± is found to have no geometrical isomers. The possible structure (s) of the complex is (where A and B are monodentate ligands)
AtetrahedralBSquare planarCBoth (a) and (b)Dcannot be predicted
The total count of geometrical isomers for a square planar complex [Mabcd]n± is 'x'. The number of geometrical isomers possible for octahedral complex of the type [Ma4b2]n± - is 'y'. Calculate x+y: _______ (a, b, c, d are monodentate ligands).
View SolutionA complex with the composition [MA3B]n± is found to have no geometrical isomers. The possible structure (s) of the complex is (where A and B are monodentate ligands)
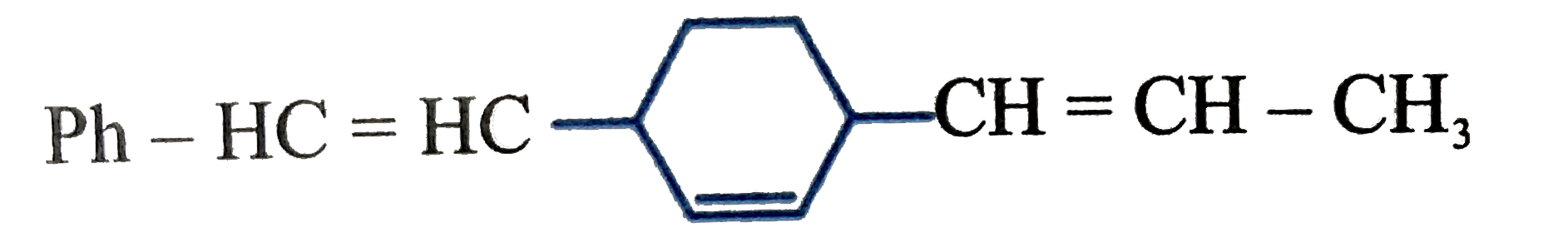
View SolutionThe total number of geometrical isomers are possible for the following compound is

View SolutionA complex with the composition [MA3B]n± is found to have no geometrical isomers. The possible structure (s) of the complex is (where A and B are monodentate ligands)
View SolutionCo-ordination complexes can show stereoisomerism square planar complexes of Ma2b2 type can show geometrical isomerism but not optical & octahedral complexes of Ma2b2C2,Ma3b3Mabcdef etc show stereoisomerism, for e.g. Mabcdef have 15 geometrical isomers & all are optically active so it has 15 pairs of enantiomers thus in totaly it has 30 stereoisomers. (a,b,c,d etc. are monodentate lignads while AB is bidenatate ligand)
How many pair of enantiomers exits for Ma2b2c2?
View Solution