Plot a graph for the equation y=ax−bx2 , where a and b are positive constants.
Video Solution
Text Solution
To find the vertex,
dydx=a−2bx=0⇒x=a2b
∴Y=a.a2b−b(a2b)2=a22b−a24b=a24b
∴ vertex is (a2b,a24b).
Also when x=0,y=0 so curve passes through origin.
[Aliter : Arrange the equation to make quadratic experssion a perfect square.
yb=−(x2−abx)=−(x2−abx+a24b2−a24b2)
=−(x2−abx+a24b2)+a24b2
1b(y−a24b)=−(x−a2b)2
∴(y−a24b)=−b(x−a2b)2 ...(1)
Putting x−a2b=0 and y−a24b=0
we get vertex (a2b,a24b)]
So it is a parabola and its axis is x=a2b.
So graph is,

|
Updated on:21/07/2023
Topper's Solved these Questions
Similar Questions
Knowledge Check
- Question 1 - Select One
If y=a+bx2, where a, b are constants, then
Ad2ydx2=2Bxd2ydx2=dydxCxd2ydx2−dydx+y=0Dxd2ydx2=2xy - Question 2 - Select One
The potential energy for a conservative system is given by
U=ax2−bx
where a and b are positive constants. The law of the force governing the system isAF = constantBF=bx–2aCF=b−2axDF=2ax - Question 3 - Select One
Order of the differential equation whose general solution is y=axbx+c, where a, b, c are arbitrary constants is
A1B2C3DNone of these
The potential energy of a conservative force field is given by
U=ax2−bx
where, a and b are positive constants. Find the equilibrium position and discuss whether the equilibrium is stable, unstable or neutral.View SolutionThe equation of motion of a projectile is y=ax−bx2, where a and b are constants of motion. Match the quantities in Column I with the relations in Column II.
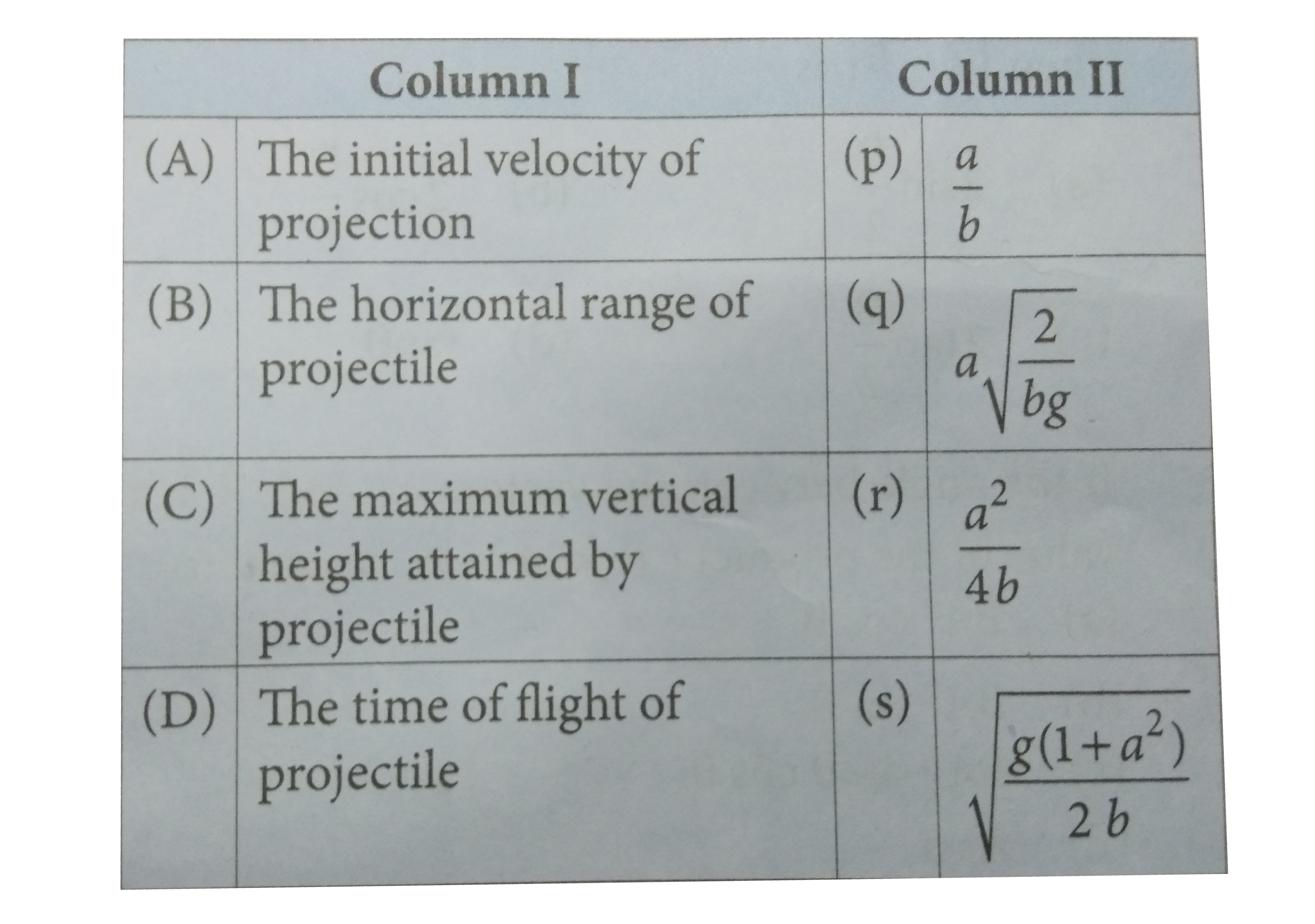 View Solution
View SolutionForm the differential equation for the curve: y=Ax2+Bx, where a and b are arbitrary constant.
View SolutionForm the differential equation not containing the arbitrary constants and satisfied by the equation y=aebx,aandb are arbitrary constants.
View SolutionFind the differential equation representing the family of curves y=aebx+5, where a and b are arbitrary constants.
View Solution

