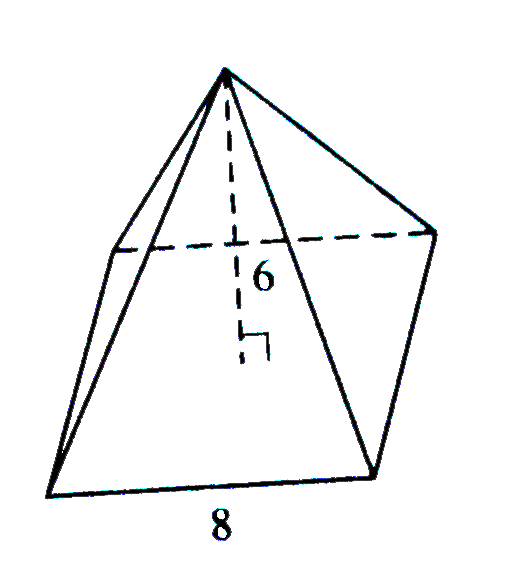
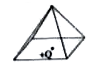
The square pyramid shown above has altitude 6 and side of square base equal to 8. What is the area of one of the triangular faces of the pyramid ?

$8 \sqrt{13}$

$16 \sqrt{3}$

Video Solution

Text Solution

Verified by Experts

The correct Answer is:A

Answer

Step by step video, text & image solution for The square pyramid shown above has altitude 6 and side of square base equal to 8. What is the area of one of the triangular faces of the pyramid ? by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.

Updated on:21/07/2023

Class 12 MATHS ADDITIONAL TOPICS

Topper's Solved these Questions

Knowledge Check

Question 1 - Select One
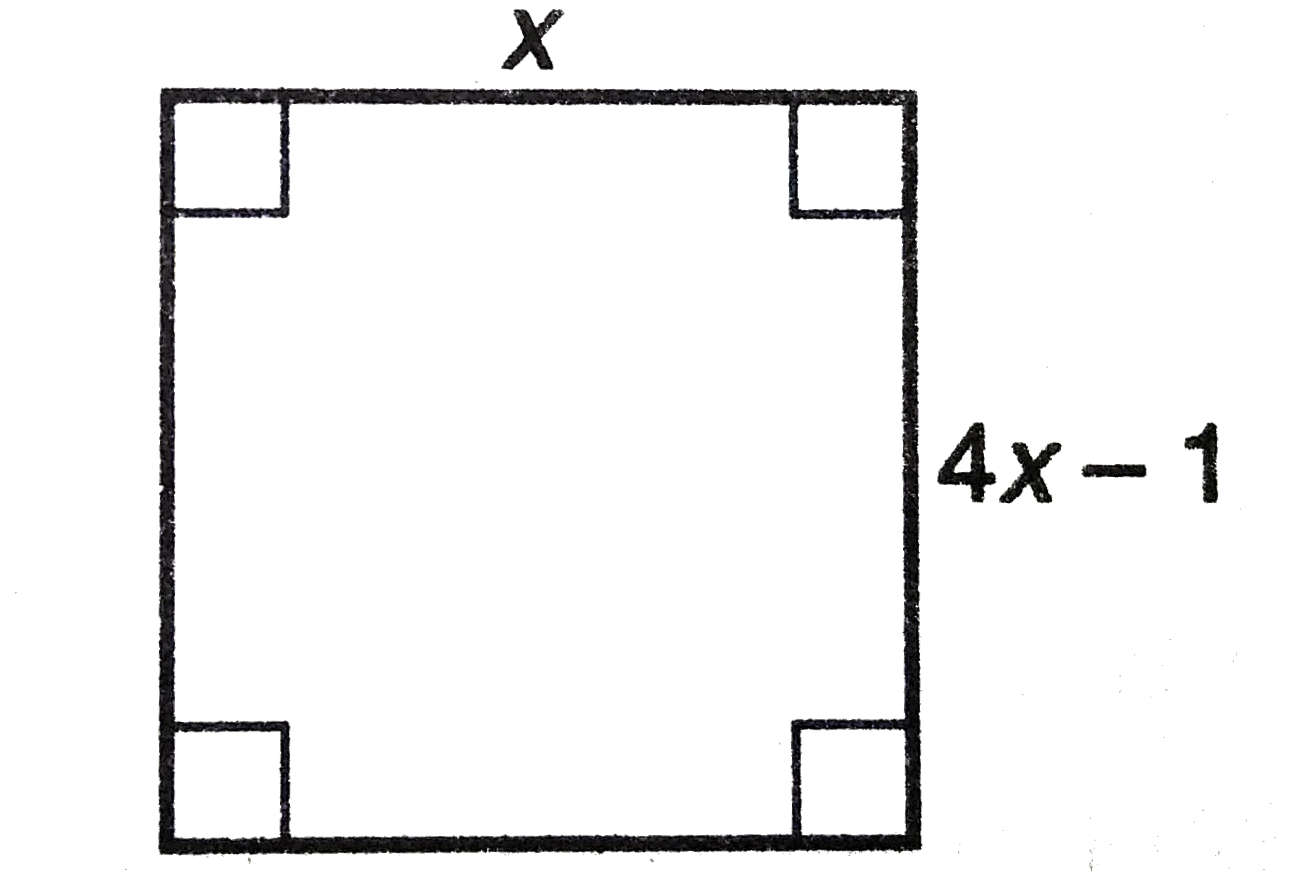
Find the surface area of a cube if the area of one of the square faces is 16.
A256
B76
C72
D96
Question 2 - Select One

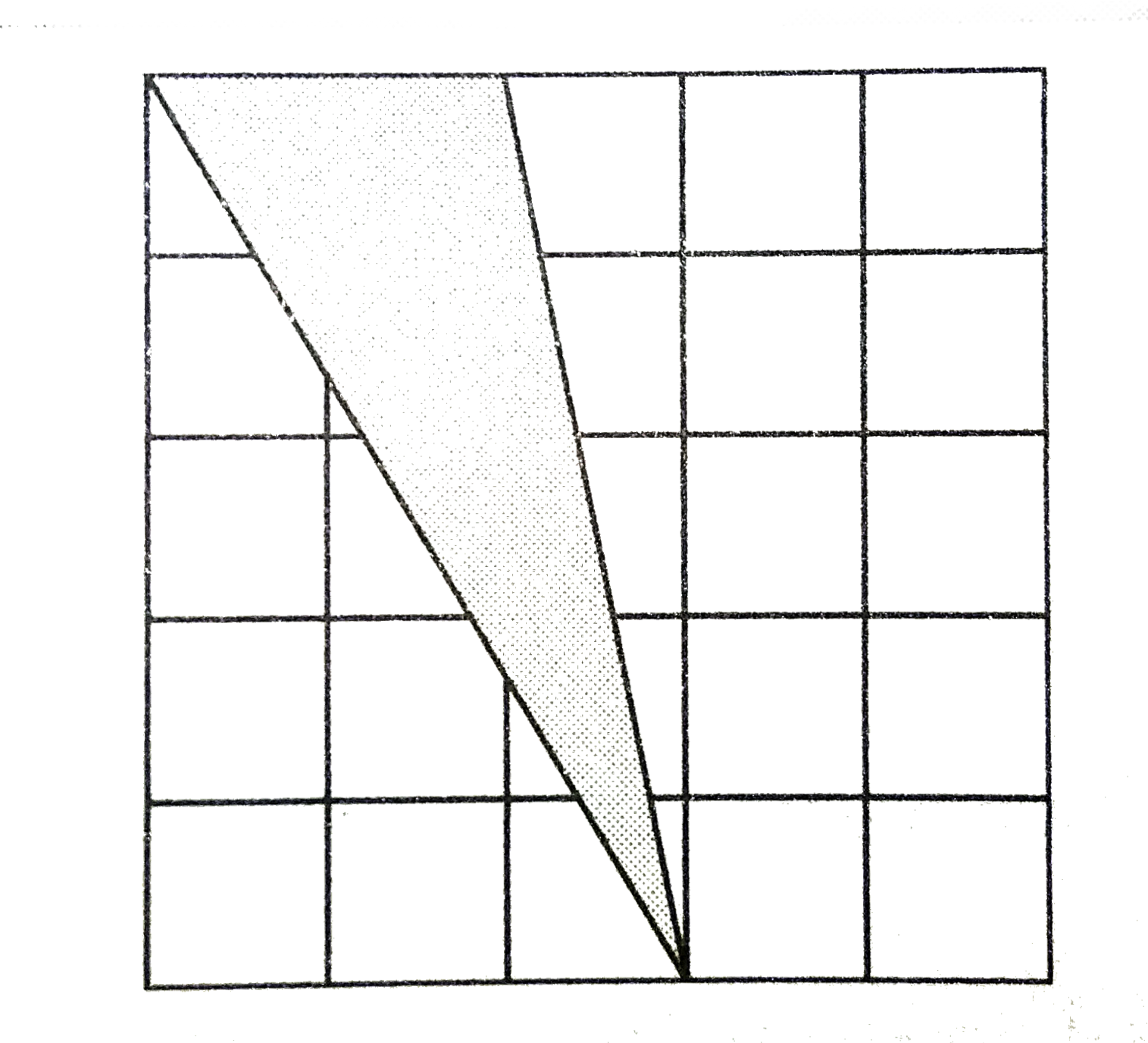
Each of the small squares in the figure above has an area of 4. If the shortest , what is the area of the shaded triangle?
A $40$
B $24$
C $20$
D $16$
Question 3 - Select One

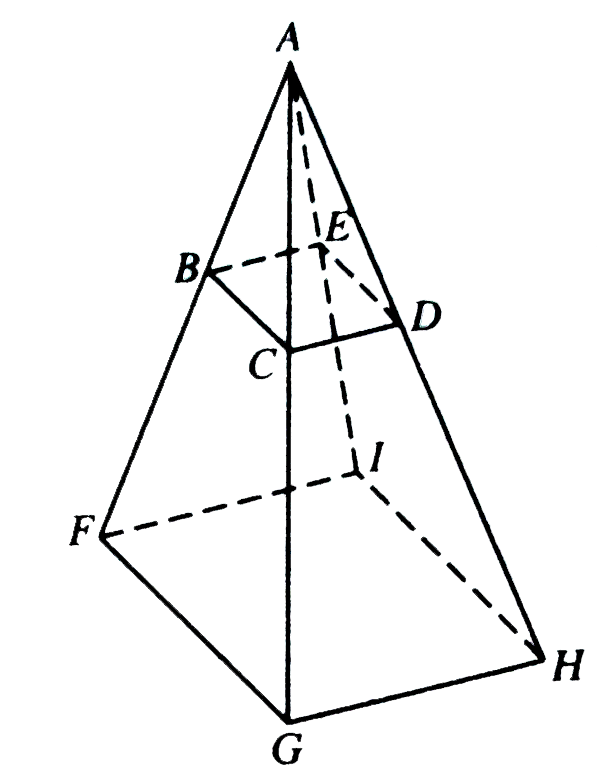
The pyramid in the figure above has square base FGHI , and all triangular faces are congruent. The base BCDE of the small pyramid is also square , and is parallel to FGHI. If the ratio of the area of square BCDE to the area of square FGHI is 1:4 , what is the ratio of the volume of the small pyramid to the volume of the large pyramid ?
A $1 : 2$
B $1 : 3$
C $1 : 4$
D $1 : 8$