In the final examinations for 9th grade students of New York Public School, 4 students failed in Mathematics Sceince and History, 16 students failed in at least two of the above - mentioned subjects and 25 students failed in at least one of the above subject . How many students failed in exactly one of the mentioned subjects ?
5
7
9
13
The correct Answer is:C
To solve the problem step by step, we will define variables and set up equations based on the information provided in the question.
Step 1: Define Variables
Let:
- f1 = number of students who failed in exactly 1 subject
- f2 = number of students who failed in exactly 2 subjects
- f3 = number of students who failed in exactly 3 subjects
Step 2: Analyze the Information Given
From the problem statement:
1. 4 students failed in Mathematics, Science, and History: This means f3=4.
2. 16 students failed in at least 2 subjects: This includes those who failed in exactly 2 subjects and those who failed in all 3 subjects. Therefore, we can write:
f2+f3=16
3. 25 students failed in at least 1 subject: This includes those who failed in exactly 1 subject, exactly 2 subjects, and exactly 3 subjects. Thus, we can write:
f1+f2+f3=25
Step 3: Set Up the Equations
From the information, we have the following equations:
1. f3=4 (from the first statement)
2. f2+4=16 (from the second statement)
3. f1+f2+4=25 (from the third statement)
Step 4: Solve for f2
Using the second equation:
f2+4=16
Subtract 4 from both sides:
f2=16−4=12
Step 5: Solve for f1
Now, substitute f2 into the third equation:
f1+12+4=25
Combine the constants:
f1+16=25
Subtract 16 from both sides:
f1=25−16=9
Conclusion
The number of students who failed in exactly 1 subject is f1=9.
Final Answer
Thus, the answer is 9 students failed in exactly one of the mentioned subjects.
---
Let:
- f1 = number of students who failed in exactly 1 subject
- f2 = number of students who failed in exactly 2 subjects
- f3 = number of students who failed in exactly 3 subjects
From the problem statement:
1. 4 students failed in Mathematics, Science, and History: This means f3=4.
2. 16 students failed in at least 2 subjects: This includes those who failed in exactly 2 subjects and those who failed in all 3 subjects. Therefore, we can write:
f2+f3=16
3. 25 students failed in at least 1 subject: This includes those who failed in exactly 1 subject, exactly 2 subjects, and exactly 3 subjects. Thus, we can write:
f1+f2+f3=25
From the information, we have the following equations:
1. f3=4 (from the first statement)
2. f2+4=16 (from the second statement)
3. f1+f2+4=25 (from the third statement)
Using the second equation:
f2+4=16
Subtract 4 from both sides:
f2=16−4=12
Now, substitute f2 into the third equation:
f1+12+4=25
Combine the constants:
f1+16=25
Subtract 16 from both sides:
f1=25−16=9
The number of students who failed in exactly 1 subject is f1=9.
Thus, the answer is 9 students failed in exactly one of the mentioned subjects.
Topper's Solved these Questions
Similar Questions
To pass an examinations a students has to pass in each of the 3 papers. In how many ways can a students fail in the examination?
View SolutionIn a class, 70 students wrote two tests wiz, test-I and test-II 50% of the students failed in test I and 40% of the students in test -II. How many students passed in both tests?
View SolutionIn a test. 90% of the students passed and 4 students failed. How many students appeared for the test?
View SolutionWrite the negation of each of the following statements.
Some students failed to qualify the entrance test.
View SolutionIn an examination, 15% students failed and 425 students passed. Find the number of students who failed in the examination, 15% students failed and 425 students passed. Find the number of students who failed in the examination.
View SolutionIn an examination of a certain class, at least 70% of the students failed in Physics, at least 72% failed in Chemistry, at least 80% failed in Mathematics and at least 85% failed in English. How many at least must have failed in all the four subjects ?
View Solution300 students took an exam. 28% failed. Calculate the number of students who passed the exam.
View SolutionIn a class of 30 students 12 take needle work, 16 take physics and 18 take history. If all the 30 students take at least one subject and no one takes all three, then the number of students taking 2 subjects is
View SolutionA class has 175 students. The following table shows the number of students studying one or more of the following subjects in this case.
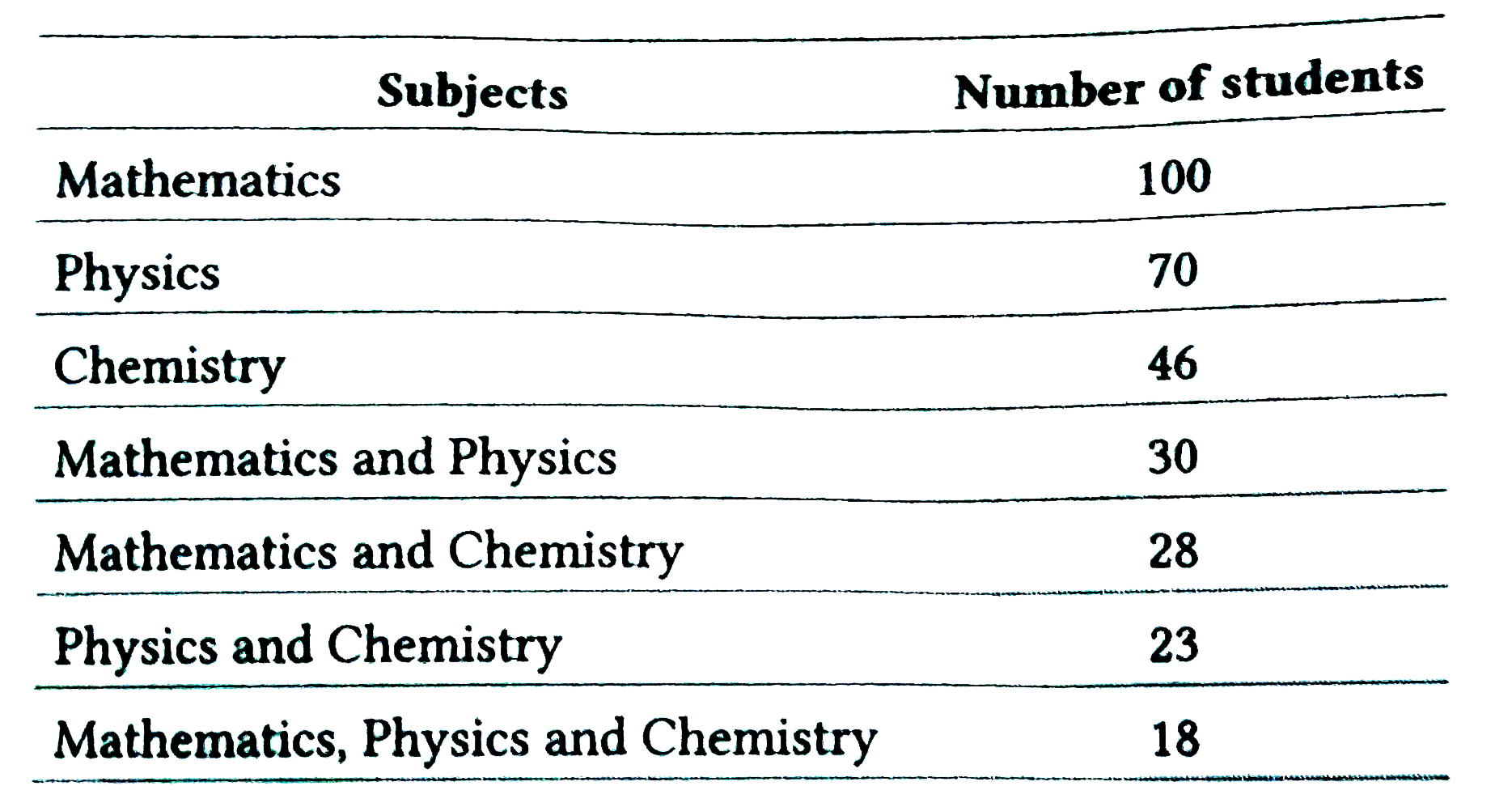
How many students are enrolled in Mathematics alone, Physics alone and Chemistry alone? Are there students who have not offered any one of these subjects?
View SolutionIn a class of 35 students, 24 like to play cricket and 16 like to play football. Also, each student likes to play at least one of the two games. How many students like to play both cricket and football?
View Solution
