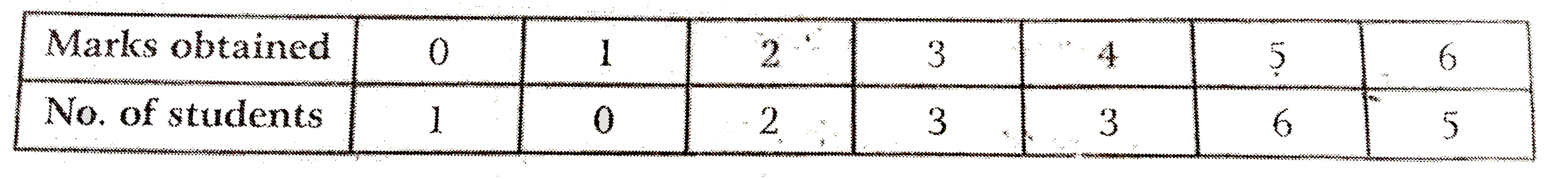The average score of S students of a class in a Physics test was 2S . If the lowest three scores were excluded, the average score of the class would have increased by three. Which of the following gives the correct expression of the average score of these three students?
S - 3
S + 3
2S -3
2S + 3
The correct Answer is:B
To solve the problem step by step, we will derive the average score of the three lowest students based on the information provided.
Step 1: Understand the initial conditions
We know that the average score of S students is given as 2S. Therefore, the total score of all students can be expressed as:
Total Score=Average×Number of Students=2S×S=2S2
Step 2: Exclude the lowest three scores
Let’s denote the scores of the students as X1,X2,X3,…,XS. The lowest three scores are X1,X2,X3. When we exclude these three scores, the total score of the remaining students becomes:
Total Score after excluding three=2S2−(X1+X2+X3)
The number of remaining students is S−3.
Step 3: Determine the new average
According to the problem, the new average after excluding the three lowest scores increases by 3. Therefore, the new average can be expressed as:
New Average=2S+3
Using the formula for average, we have:
2S2−(X1+X2+X3)S−3=2S+3
Step 4: Set up the equation
Now, we can set up the equation based on the new average:
2S2−(X1+X2+X3)=(2S+3)(S−3)
Step 5: Expand the right-hand side
Expanding the right-hand side gives:
(2S+3)(S−3)=2S2−6S+3S−9=2S2−3S−9
Thus, our equation now looks like:
2S2−(X1+X2+X3)=2S2−3S−9
Step 6: Solve for the sum of the lowest three scores
Rearranging the equation to isolate X1+X2+X3:
−(X1+X2+X3)=−3S−9
This simplifies to:
X1+X2+X3=3S+9
Step 7: Calculate the average of the three lowest scores
To find the average score of the three lowest students, we divide their total score by 3:
Average of X1,X2,X3=X1+X2+X33=3S+93=S+3
Final Answer
The average score of the three lowest students is:
S+3
We know that the average score of S students is given as 2S. Therefore, the total score of all students can be expressed as:
Total Score=Average×Number of Students=2S×S=2S2
Let’s denote the scores of the students as X1,X2,X3,…,XS. The lowest three scores are X1,X2,X3. When we exclude these three scores, the total score of the remaining students becomes:
Total Score after excluding three=2S2−(X1+X2+X3)
The number of remaining students is S−3.
According to the problem, the new average after excluding the three lowest scores increases by 3. Therefore, the new average can be expressed as:
New Average=2S+3
Using the formula for average, we have:
2S2−(X1+X2+X3)S−3=2S+3
Now, we can set up the equation based on the new average:
2S2−(X1+X2+X3)=(2S+3)(S−3)
Expanding the right-hand side gives:
(2S+3)(S−3)=2S2−6S+3S−9=2S2−3S−9
Thus, our equation now looks like:
2S2−(X1+X2+X3)=2S2−3S−9
Rearranging the equation to isolate X1+X2+X3:
−(X1+X2+X3)=−3S−9
This simplifies to:
X1+X2+X3=3S+9
To find the average score of the three lowest students, we divide their total score by 3:
Average of X1,X2,X3=X1+X2+X33=3S+93=S+3
The average score of the three lowest students is:
S+3
Similar Questions
Knowledge Check
In a class of 10 boys and 15 girls, the average score on a biology test is 90. If the average score for the girls is x, what is the average score for the boys in terms of x ?
A200−23xB225−32xC250−2xD250−3xThe average of the test scores of a group of x students is 76 and the average of the test scores of a group of y students is 90. When the scores of the two groups of students are combined, the average test score is 85. What is the value of of xy?
A47B59C23D74Caroline scored 85, 88, and 89 on three of her four history tests. If her average score for all tests was 90, what did she score on her fourth test?
A90B93C96D98
All the students of a class performed poorly in physics. The teacher decided to give grace marks of 15 to the entire class. Which of the following statistical measures will not change even after the grace marks were given?
View SolutionThe average scored by the students of a class in English is 64. The average of marks scored by boys and the girls are respectively 68 and 58. Then find the ratio of the number of boys to the number of girls.
View SolutionIn State X,Mr. Camp’s eighth-grade class consisting of 26 students was surveyed and 34.6percent of the students reported that they had at least two siblings. The average eighth‑grade class size in the state is 26. If the students in Mr. Camp’s class are representative of students in the state’s eighth-grade classes and there are 1,800 eighth-grade classes in the state, which of the following best estimates the number of eighth‑grade students in the state who have fewer than two siblings?
View SolutionJoe distributed some pens among the other students of his class. The number of students among whom he distrubted pens was 3 more than the number of pens each got . If the total number of pens distributed is less than 21,which of the following is the correct inequality for the total number of students s, in the class ?
View SolutionA group of students takes a test and the average score is 72. One more student takes the test and receives a score of 88 increasing the average score of the group to 76. How many students were in the initial group ?
View Solution