A class of 50 students belonging to the Kindergraten is divided in two sections A and B , having students in the ratio 2:3 respectively. The average weight of the students of the entire calss is 40 Ibs. The average weight of the students of section A is 2 Ibs . less than that of the students B. What is the average weight of the students of section B ?
32.6 Ibs.
36.4 Ibs .
38.8 Ibs.
40.8 Ibs.
The correct Answer is:D
To solve the problem step by step, we will break it down as follows:
Step 1: Determine the number of students in each section
The total number of students is 50, and they are divided in the ratio of 2:3 for sections A and B.
Let the number of students in section A be 2x and in section B be 3x.
Since the total number of students is 50:
2x+3x=50
5x=50
x=10
Thus, the number of students in section A is:
2x=2×10=20
And in section B:
3x=3×10=30
Step 2: Set up the average weights
Let the average weight of students in section B be x lbs. According to the problem, the average weight of students in section A is 2 lbs less than that of section B. Therefore, the average weight of students in section A is:
x−2 lbs
Step 3: Calculate the total weight of each section
The total weight of students in section A can be calculated as:
Total weight of A=Average weight of A×Number of students in A=(x−2)×20
The total weight of students in section B is:
Total weight of B=Average weight of B×Number of students in B=x×30
Step 4: Set up the equation for total weight
The total weight of the entire class is the sum of the weights of sections A and B. The average weight of the entire class is given as 40 lbs, so the total weight is:
Total weight=50×40=2000 lbs
Now we can set up the equation:
20(x−2)+30x=2000
Step 5: Simplify and solve the equation
Expanding the equation:
20x−40+30x=2000
Combining like terms:
50x−40=2000
Adding 40 to both sides:
50x=2040
Dividing by 50:
x=204050=40.8
Step 6: Conclusion
The average weight of the students in section B is:
40.8 lbs
---
The total number of students is 50, and they are divided in the ratio of 2:3 for sections A and B.
2x+3x=50
5x=50
x=10
2x=2×10=20
And in section B:
3x=3×10=30
Let the average weight of students in section B be x lbs. According to the problem, the average weight of students in section A is 2 lbs less than that of section B. Therefore, the average weight of students in section A is:
x−2 lbs
The total weight of students in section A can be calculated as:
Total weight of A=Average weight of A×Number of students in A=(x−2)×20
The total weight of students in section B is:
Total weight of B=Average weight of B×Number of students in B=x×30
The total weight of the entire class is the sum of the weights of sections A and B. The average weight of the entire class is given as 40 lbs, so the total weight is:
Total weight=50×40=2000 lbs
20(x−2)+30x=2000
Expanding the equation:
20x−40+30x=2000
Combining like terms:
50x−40=2000
Adding 40 to both sides:
50x=2040
Dividing by 50:
x=204050=40.8
The average weight of the students in section B is:
40.8 lbs
Similar Questions
The mean weight of 120 students of a school is 52⋅75 kg. If the mean weight of 50 of them is 51 kg, find the mean weight of the remaining students.
View SolutionThe mean weight of 120 students of a school is 52⋅75 kg. If the mean weight of 50 of them is 51 kg, find the mean weight of the remaining students.
View SolutionThe average weght of the students in a class of 39 students is 40 kg. If the weight of the teacher is to be included, then the average rises by 14kg. The weight of the teacher is
View SolutionThe average weight of students in a class of 32 students is 40 kg. If the weight of the teacher be included, the average rises by 13kg, then the weight of the teacher is
View SolutionThe mean weigth of 30 students of a class is 60.2kg . Two students of weights of 50 kg and 67 kg left the class . Find the mean weight of the remaining students
View SolutionFollowing table shows the weight of 12 students: Weight (in kgs) 67 70 72 73 75 Number of Students: 4 3 2 2 1 Find the mean weight of the students.
View SolutionThe mean weight of 60 students in a class is 40 kg. The mean weight of boys is 50 kg while that of girls is 30 kg. Find the number of boys and girls in the class.
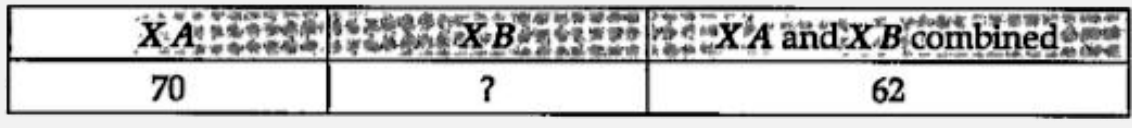
View SolutionThe number of students in section X A and X B are 30 and 35 respectively. The mean scores of students in the mathematices test are as follows:
View SolutionIn a class of 40 students, 45% are girls and the remaining are boys. If the average of the girls marks is 54 and that of the boys is 46, what is the average of the whole class?
View SolutionFollowing table shows the weights of 12 students: Find the mean weight.
View Solution