If x2−4x−32>0 and |x−2|<7 how many integer solutions of x exist ?
0
9
13
15
To solve the inequalities given in the question, we will break it down into manageable steps.
Step 1: Solve the inequality |x - 2| < 7
The inequality |x - 2| < 7 can be rewritten as two separate inequalities:
1. x−2<7
2. −(x−2)<7 or x−2>−7
Solving the first inequality:
x−2<7⟹x<9
Solving the second inequality:
x−2>−7⟹x>−5
Thus, combining both inequalities, we have:
−5<x<9
Step 2: Solve the inequality x² - 4x - 32 > 0
First, we will find the roots of the equation x2−4x−32=0 using the quadratic formula:
x=−b±√b2−4ac2a
where a=1,b=−4,c=−32.
Calculating the discriminant:
b2−4ac=(−4)2−4⋅1⋅(−32)=16+128=144
Now substituting back into the formula:
x=4±√1442⋅1=4±122
This gives us two roots:
1. x=162=8
2. x=−82=−4
Step 3: Determine the intervals for x² - 4x - 32 > 0
The roots divide the number line into three intervals:
1. (−∞,−4)
2. (−4,8)
3. (8,∞)
We need to test each interval to see where the inequality holds true.
Testing the interval (−∞,−4):
Choose x=−5:
(−5)2−4(−5)−32=25+20−32=13>0
Testing the interval (−4,8):
Choose x=0:
02−4(0)−32=−32<0
Testing the interval (8,∞):
Choose x=10:
102−4(10)−32=100−40−32=28>0
Thus, the solution to the inequality x2−4x−32>0 is:
x∈(−∞,−4)∪(8,∞)
Step 4: Combine the solutions
Now we combine the results from Step 1 and Step 2:
1. From Step 1: −5<x<9
2. From Step 2: x∈(−∞,−4)∪(8,∞)
The overlapping intervals are:
- For (−∞,−4): The range is −5<x<−4 which gives us the integer solution −5.
- For (8,∞): The range is 8<x<9 which gives no integer solutions.
Conclusion
The only integer solution that satisfies both inequalities is:
Integer solution: −5
Thus, the total number of integer solutions is 1.
---
1. x−2<7
2. −(x−2)<7 or x−2>−7
x−2<7⟹x<9
x−2>−7⟹x>−5
−5<x<9
x=−b±√b2−4ac2a
where a=1,b=−4,c=−32.
b2−4ac=(−4)2−4⋅1⋅(−32)=16+128=144
x=4±√1442⋅1=4±122
This gives us two roots:
1. x=162=8
2. x=−82=−4
1. (−∞,−4)
2. (−4,8)
3. (8,∞)
Choose x=−5:
(−5)2−4(−5)−32=25+20−32=13>0
Choose x=0:
02−4(0)−32=−32<0
Choose x=10:
102−4(10)−32=100−40−32=28>0
x∈(−∞,−4)∪(8,∞)
1. From Step 1: −5<x<9
2. From Step 2: x∈(−∞,−4)∪(8,∞)
- For (−∞,−4): The range is −5<x<−4 which gives us the integer solution −5.
- For (8,∞): The range is 8<x<9 which gives no integer solutions.
Integer solution: −5
Similar Questions
Knowledge Check
If 2x−5≤5x+4<29 and x is an integer, then the solution set of x is
A{−2,−1,0,1,2,3,4,5}B{−2,−1,0,1,2,3,4}C{−3,−2,−1,0,1,2,3,4,5}D{−3,−2,−1,0,1,2,3,4}If 2x−3≤x+1≤4x+7,x∈I (integers), then the solution set of x is
A{−2,−1,0,1,2,3,4}B{−1,0,1,2,3}C{−2,−1,0,1,2,3}D{−1,0,1,2,3,4}If 2x−3<x+1<4x+7,x∈R then the solution set of x on the number line is:
A B
B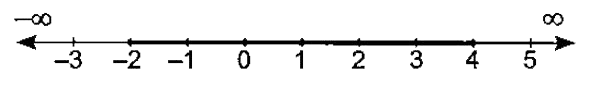 C
C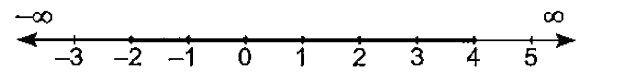 D
D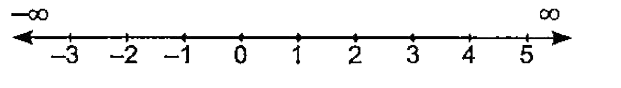
The solution of 6+x−x2>0, is
View SolutionSolve tanx−tan2x>0and|2sinx|<1 .
View SolutionHow many integer value of x satisfy 5+2|2x−3|<19 ?
View Solutionx3(x2−5)=−4x
If x>0, what is one possible solutions to the equation above?
View SolutionHow many integers are there in the solution set of |x−2|≤5?
View Solution
