How many points with integer coordinates lie on or inside a circle of radius three units centered at the origin?
16
28
29
30
The correct Answer is:C
Step by step video, text & image solution for How many points with integer coordinates lie on or inside a circle of radius three units centered at the origin? by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Topper's Solved these Questions
- ADDITIONAL TOPICS IN MATHBOOK - ENGLISH SATCHAPTER - ADDITIONAL TOPICS IN MATHEXERCISE - Grib-In
32 Videos
- ADDITIONAL TOPICS IN MATH BOOK - ENGLISH SATCHAPTER - ADDITIONAL TOPICS IN MATH EXERCISE - PRACTICE TEST
24 Videos
- COMPLEX NUMBERSBOOK - ENGLISH SATCHAPTER - COMPLEX NUMBERSEXERCISE - EXERCISE
6 Videos
Similar Questions
Where does a point having y-coordinate −2 lie?
View SolutionWhere does a point having y-coordinate −5 lie?
View SolutionThe number of points with integral coordinates that lie in the interior of the region common to the circle x2+y2=16 and the parabola y2=4x, is
View SolutionFind the number of integral points which lie on or inside the circle x2+y2=4.
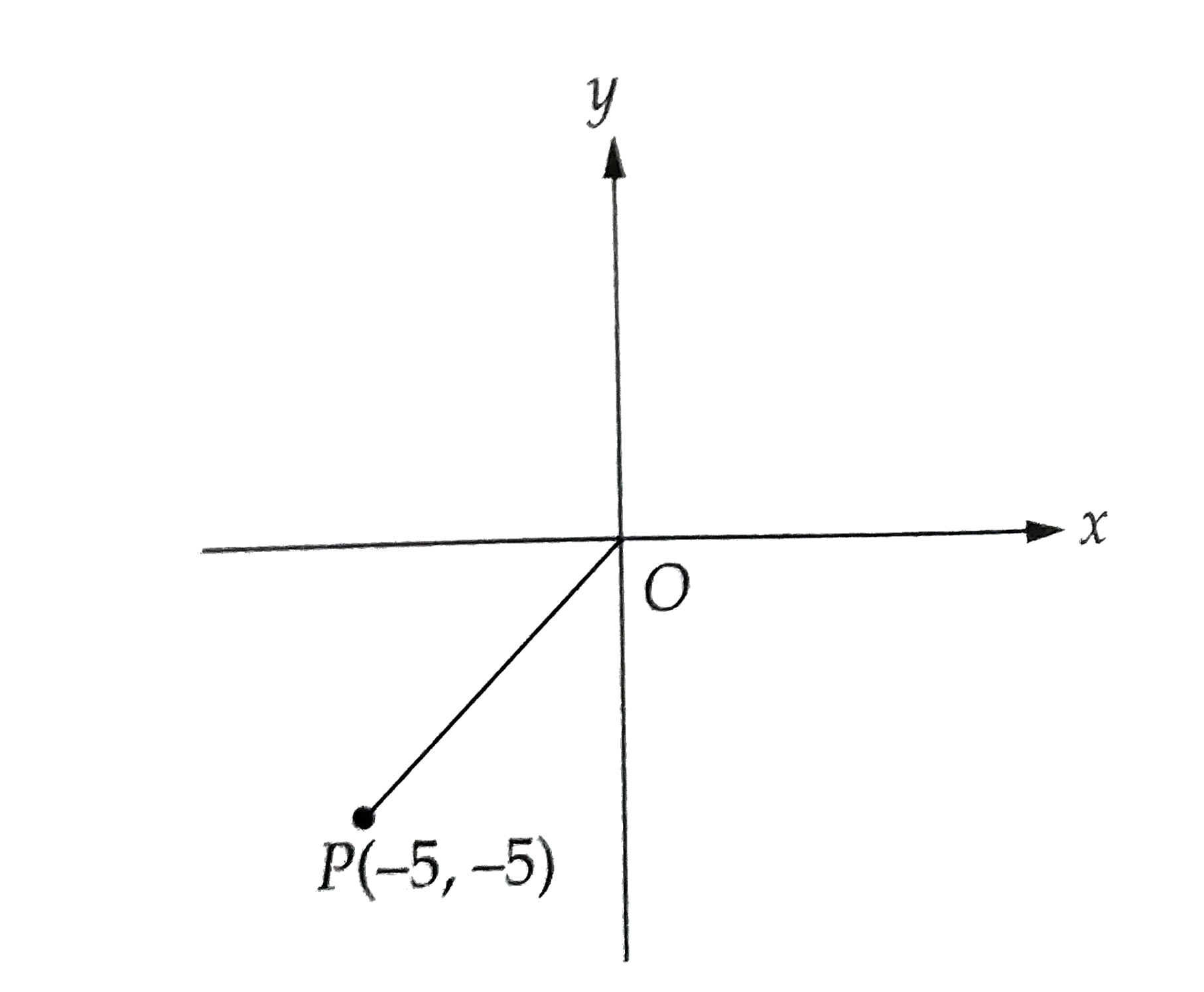
View SolutionA circle of radius 1 is placed on an incline where point P, a point on the circle, has the coordinates (−5,−5). The circle is rolled up the incline, and once the circle hits the origin, the circle is then rolled horizontally along the x - axis to the right. What is the x - coordinate of the point where P touches the incline or the x - axis for the fifth time (not including the starting point) ?
View SolutionThe locus of the midpoint of a line segment that is drawn from a given external point P to a given circle with center O (where O is the orgin) and radius r is a straight line perpendiculat to PO a circle with center P and radius r a circle with center P and radius 2r a circle with center at the midpoint PO and radius r2.
View SolutionPlot any three points such that x-coordinate of each point is equal to its y-coordinate. Join these points in pairs. Do they lie on a line passing through the origin?
View SolutionProve that the maximum number of points with rational coordinates on a circle whose center is (√3,0) is two.
View SolutionProve that the maximum number of points with rational coordinates on a circle whose center is (√3,0) is two.
View SolutionThe differential equation of circles passing through the points of intersection of unit circle with centre at the origin and the line bisecting the first quadrant, is
View Solution
