The lengths of the triangle shown below are rounded to the nearest 0.1 cm. What is the area, to the nearest 1cm2 of the triangle?
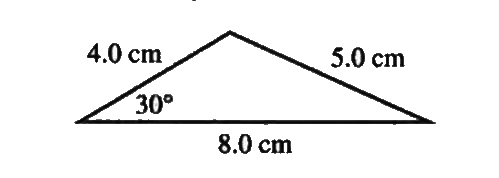
The lengths of the triangle shown below are rounded to the nearest 0.1 cm. What is the area, to the nearest 1 cm^(2) of the triangle? Note: The area of any triangle with sides of length a, b and c opposite angle of measure A, B, and C, respectively, is given by 1/2 ab sin C.
Note: The area of any triangle with sides of length a, b and c opposite angle of measure A, B, and C, respectively, is given by 12absinC.

A

4

B

5

C

8

D

10

Video Solution
Doubtnut Promotions Banner
Text Solution
Verified by Experts

The correct Answer is:C

Updated on:21/07/2023
|
Answer

Step by step video, text & image solution for The lengths of the triangle shown below are rounded to the nearest 0.1 cm. What is the area, to the nearest 1 cm^(2) of the triangle? Note: The area of any triangle with sides of length a, b and c opposite angle of measure A, B, and C, respectively, is given by 1/2 ab sin C. by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.

Updated on:21/07/2023
Class 12MATHSQUESTION BANK

Topper's Solved these Questions

Similar Questions

Knowledge Check

  • The triangle shown below has side lengths 37, 38 and 39 inches. Which of the following expression gives the measure of the largest angle of the triangle?
    (Note : For every triangle with sides of length a, b and c that are opposite ∠A,∠B,and∠C, respectively. c2=a2+b2−2abcosC.)
    ocr_image

    Acos−1(−372−382−3922(38)(39))
    Bcos−1(−392−372−3822(37)(38))
    Ccos−1(372−382−392+2(38)(39))
    Dcos−1(382−372−392+2(37)(39))