Twenty metres of wire is available for fencing off a flower-bed in the form of a circular sector. Then the maximum area (in sq.m) of the flower-bed is: 25 (2) 30 (3) 12.5 (4) 10
To solve the problem of maximizing the area of a flower-bed in the form of a circular sector, we will follow these steps:
Step 1: Define the variables
Let R be the radius of the circular sector and θ be the angle in radians of the sector. The total length of the wire available for fencing is given as 20 meters.
Step 2: Write the equation for the length of the fencing
The length of the fencing consists of two radii and the arc length, which can be expressed as:
2R+Rθ=20
This simplifies to:
R(2+θ)=20
From this, we can express θ in terms of R:
θ=20R−2
Step 3: Write the equation for the area of the circular sector
The area A of the circular sector is given by:
A=12R2θ
Substituting the expression for θ from Step 2 into the area formula:
A=12R2(20R−2)
This simplifies to:
A=12R2⋅20R−R2
A=10R−R2
Step 4: Differentiate the area with respect to R
To find the maximum area, we differentiate A with respect to R:
dAdR=10−2R
Step 5: Set the derivative equal to zero to find critical points
Setting the derivative equal to zero to find the value of R that maximizes the area:
10−2R=0
Solving for R:
2R=10⟹R=5 meters
Step 6: Verify that this is a maximum
To confirm that this critical point is a maximum, we can check the second derivative:
d2AdR2=−2
Since the second derivative is negative, this indicates that the area is maximized at R=5.
Step 7: Calculate the angle θ
Substituting R=5 back into the equation for θ:
θ=205−2=4−2=2 radians
Step 8: Calculate the maximum area
Now we can calculate the maximum area using the values of R and θ:
A=12R2θ=12⋅52⋅2=12⋅25⋅2=25 square meters
Final Answer
The maximum area of the flower-bed is 25 square meters.
---
Let R be the radius of the circular sector and θ be the angle in radians of the sector. The total length of the wire available for fencing is given as 20 meters.
The length of the fencing consists of two radii and the arc length, which can be expressed as:
2R+Rθ=20
This simplifies to:
R(2+θ)=20
From this, we can express θ in terms of R:
θ=20R−2
The area A of the circular sector is given by:
A=12R2θ
Substituting the expression for θ from Step 2 into the area formula:
A=12R2(20R−2)
This simplifies to:
A=12R2⋅20R−R2
A=10R−R2
To find the maximum area, we differentiate A with respect to R:
dAdR=10−2R
Setting the derivative equal to zero to find the value of R that maximizes the area:
10−2R=0
Solving for R:
2R=10⟹R=5 meters
To confirm that this critical point is a maximum, we can check the second derivative:
d2AdR2=−2
Since the second derivative is negative, this indicates that the area is maximized at R=5.
Substituting R=5 back into the equation for θ:
θ=205−2=4−2=2 radians
Now we can calculate the maximum area using the values of R and θ:
A=12R2θ=12⋅52⋅2=12⋅25⋅2=25 square meters
The maximum area of the flower-bed is 25 square meters.
Topper's Solved these Questions
Similar Questions
Twenty metres of wire is available for fencing off a flower-bed in the form of a circular sector. Then the maximum area (in sq.m) of the flower-bed is: 25 (2) 30 (3) 12.5 (4) 10
View SolutionThree plots having areas 110, 130 and 190 square metres are to be subdivided into flower beds of equal size. If the breadth of a bed is 2 metre, the maximum length of a bed can be (a) 5m (b) 11m (c) 13m (d) 19m
View Solution
Knowledge Check
- Question 1 - Select One
Twenty meters of wire is available for fencing a flower -bed in the form of a circular sector .Then the maximum area in square meters of the flower bed is
A12.5B10C25D30 - Question 2 - Select One
Shown below is the top view of a stadium. There is a badminton court at the centre. The stadium is surrounded by a jogging track. The track is semi-circular in shape at the top and the bottom of the court. The fountains converge at the centre of the respective semicircles. The jogging track has a uniform width of 2 m.
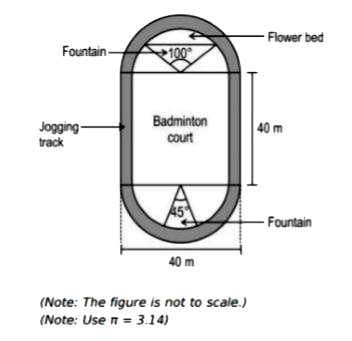
The cost of gardening is Rs 300/m2 and the area of the fountain next to the flower bed is 150 m2.
What is the cost of gardening the flower bed?ARs 39780BRs 45000CRs 59664DRs 84780
A square lawn with side 100 m long has a circular flower bed in the centre. If the area of the lawn, excluding the flower bed, is 8614 m2, the radius of the circular flower bed is (a) 21 m (b) 31 m (c) 41 m (d) None of these
View SolutionFind maximum possible area that can be enclosed by a wire of length 20cm by bending it in form of a circular sector. 10 (b) 25 (c) 30 (d) 20
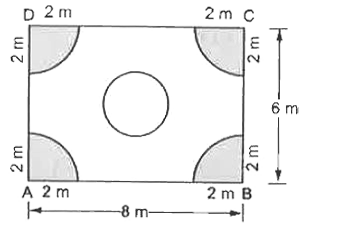
View SolutionIn each of the corners, there is a flower bed in the form of a quadrant of a circle of radius 2 m. Also, there is a flower bed in the form of a circle of radius 2 m in the middle of the plot. Find the area of the remaining plot.
 View Solution
View SolutionMaximum possible area that can be enclosed by a wire of length 20 cm by bending it in form of a circular sector is 10 b. 25 c. 30 d. 20
View SolutionA copper wire of length 36 m and diameter 2 mm is melted to form a sphere. The radius of the sphere (in cm) is (a) 2.5 (b) 3 (c) 3.5 (d) 4
View SolutionA square park has each side of 100 m. At each corner of the park, there is a flower bed in the form of a quadrant of radius 14 m as shown in Fig. 15.37. Find the area of the remaining part of the park (Use π=22/7) . (FIGURE)
View Solution

