The diameter of a ball was measured five times with the aid of a micrometer whose absolute error (Δdinst)=±0.01mm. The results of measuring the diameter of the ball are d1=5.27mm,d2=5.30mm,d3=5.28mm,d4=5.32mm and d5=5.28mm. Find (a) mean value of ball diameter (b) mean absolute error (c) result of measurement (d) relative error (e) persentage error.
(a) The mean value of the ball diameter, i.e.,
dm=5.27+5.30+5.28+5.32+5.285=5.29mm
(b) The absolute error in the measurements are :
Δd1=|dm−d1|=0.02mm
Δd2=|dm−d2|=0.01mm
Δd3=|dm−d3|=0.01mm
Δd4=|dm−d4|=0.03mm
Δd5=|dm−d5|=0.01mm
Mean absolute error,
Δdmean=0.02+0.01+0.01+0.03+0.015
≅0.02mm.
(c) Since the mean absolute error (Δdmean) is greater than the instrumental error (Δdinst), the result of measurement is
d=dm±Δdmean=(5.29±0.02)mm
(As a rule, we take either Δdmean or Δdinst, depending upon which of these errors is greater)
(d) Relative error, Δdmeandm=0.025.29=0.004
(e) Percentage error, Δdmeandm×100=0.004×100=0.4%
Topper's Solved these Questions
- BOOK - GRB PUBLICATIONCHAPTER - UNITS AND DIMENSIONSEXERCISE - Problem For Practice26videos
UNITS AND DIMENSIONS
- BOOK - GRB PUBLICATIONCHAPTER - UNITS AND DIMENSIONSEXERCISE - Objective Type Question103videos
UNITS AND DIMENSIONS
- BOOK - GRB PUBLICATIONCHAPTER - MOTION IN TWO AND THREE DIMENSIONSEXERCISE - All Questions210videos
MOTION IN TWO AND THREE DIMENSIONS
- BOOK - GRB PUBLICATIONCHAPTER - VECTORSEXERCISE - PASSAGE -33videos
VECTORS
Similar Questions
Knowledge Check
- Question 1 - Select One
The diameter of a wire is measured by using a screw gauge having least count 0.01mm. If the diameter is found to be 0.20mm, then the error in the cross-section of the wire will be
A5%B10%C1%D2.5% - Question 2 - Select One
The length of a cyclinder is measured as 5 cm using a vernier calipers of least count 0.1 mm. The percentage error in the measured length is nearly
A0.5%B2%C20%D0.2%
The diameter of a wire as measured by a screw gauge was found to be 0.026 cm, 0.028 cm, 0.029 cm, 0.027cm, 0.024cm and 0.027 cm. Calculate
(i) mean value of diameter
(ii) mean absoulte error
(iii) relative error (iv) percentage error. Also express the result in terms of absolute error and percentage error.View SolutionThe diameter of a wire as measured by screw gauge was found to be 2.620, 2.625, 2.630, 2.628 and 2.626 cm. Calculate
(a) mean value of diameter (b) absolute error in each measurement
(c) mean absolute error (d) fractional error
(e) percentage error (d) (f) Express the result in terms of percentage errorView SolutionThe circular divisions of shown screw gauge are 50. It moves 0.5 mm on main scale in one rotation. The diameter of the ball is
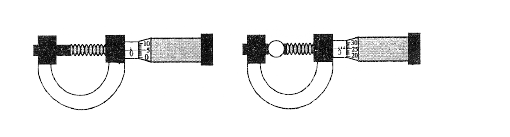 View Solution
View SolutionThe diameters of two cylinders are d1=(2.02±0.01)cm and d2=(3.21±0.03). Find the difference between their diameters along with the error limits.
View SolutionIn an experiment the refractive index of glass was observed to be 1.45,1.56,1.54,1.44,1.54,and1.53. Calculate
(a). Mean value of refractive index
(b). Mean absolute error
( c ) Fractional error
(d) Percentage error
(e) Express the result in terms of absolute error and percentage errorView SolutionThe diameter of a wire as measured by a Screw gauge was found to be 1.328, 1.330, 1.325, 1.326, 1.334 and 1.336 cm. Calculate (i) mean value of diameter (ii) absolute error in each measurement (iii) mean absolute error (iv) fractional error (v) percentage error. Also, express the result in term of absolute error and percentage error.
View Solution

