A compound formed by elements X and Y has a cubic structure in whch X atoms are at the corner of the cube and also at the face centers. Y atoms are present at the body center and at the edge center of the cube.
If all the atoms are removed from one of the plane passing through the middle of the cube, calculate total number of atoms in the cube,
8
9
12
18
Plane passing through the middle of cube (other atoms are not shown)
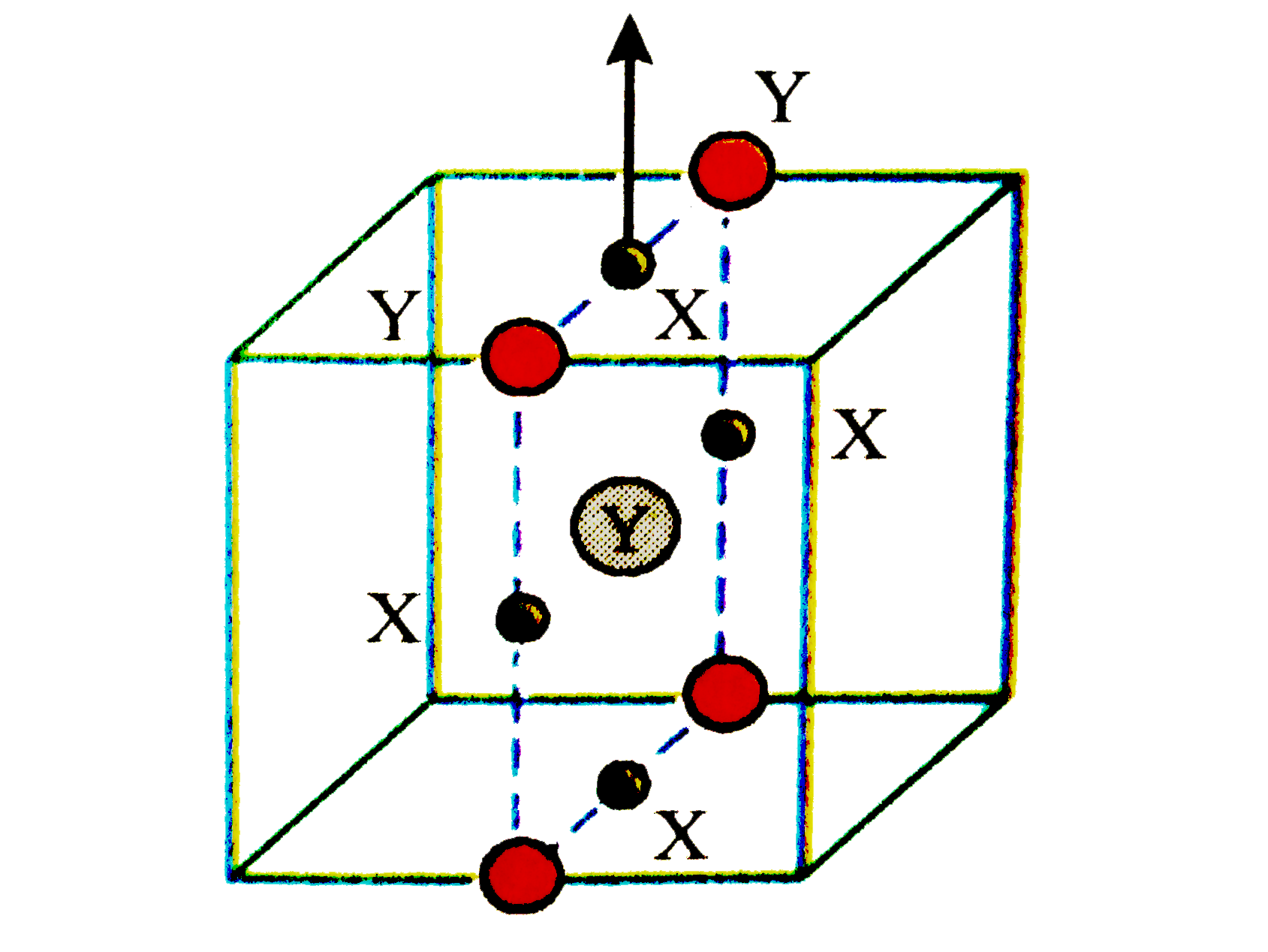
Y⇒1 body center atom is removed
Y⇒4 edge center atoms are removed
X⇒4 face center atoms are removed
Total number of atom in the cube =8 (corner)+2 (face centeres) +zero (body center)+8 (edge centers).
Topper's Solved these Questions
- SOLID STATEBOOK - NARAYNACHAPTER - SOLID STATEEXERCISE - LEVEL-II(H.W)
34 Videos
- S-BLOCK ELEMENTSBOOK - NARAYNACHAPTER - S-BLOCK ELEMENTSEXERCISE - LEVEL-4
15 Videos
- SOME BASIC CONCEPTS IN CHEMISTRY STOICHIOMETRY (PART-I)BOOK - NARAYNACHAPTER - SOME BASIC CONCEPTS IN CHEMISTRY STOICHIOMETRY (PART-I)EXERCISE - All Questions
555 Videos
Similar Questions
A compound formed by elements A and B has a cubic structure in which A atoms are at the corner of the cube and B atoms are at the face centres. Derive the fomula of the compound.
View SolutionA compound formed by elements A and B has a cubic structure in which A atoms are at the corner of the cube and B atoms are at the face centres. Derive the fomula of the compound.
View Solution
Knowledge Check
A compound formed by elements X and Y has a cubic structure in which X atoms are at the corner of the cube and also at alternate face centers. Y atoms are present at the body center and also at the alternate edge center of the cube.
If all the atoms are removed from one of the plane passing through the middle of the cube which contains atoms both on the edge center as well as on the face center, calculateA1B2C3D4A compound formed by elements X and Y has a cubic structure in whch X atoms are at the corner of the cube and also at the face centers. Y atoms are present at the body center and at the edge center of the cube.
If all the atoms are removed from one of the axis passing through one of the face centers of the cube, then formula of the compound isAXYBXY2CX2YDXY3A compound formed by elements X and Y has a cubic structure in which X atoms are at the corner of the cube and also at alternate face centers. Y atoms are present at the body center and also at the alternate edge center of the cube.
If all the atoms are removed from one of the plane passing through the middle of the cube which neither contains atoms on the edge center nor on the face center, total number of atoms in a cube.A8B12C14D18
A compound formed by elements A and B has a cubic structure in which A atoms are at the corner of the cube and B atoms are at the face centres. Derive the fomula of the compound.
View SolutionA compound formed by elements X and Y has a cubic structure in which X atoms are at the corner of the cube and also at alternate face centers. Y atoms are present at the body center and also at the alternate edge center of the cube.
If all the atoms are removed from one of the axis passing through the middle of the cube not containing face center atoms, formula of the compounds 64. If all the
View SolutionA compound formed by elements X and Y has a cubic structure in whch X atoms are at the corner of the cube and also at the face centers. Y atoms are present at the body center and at the edge center of the cube.
If all the atoms from the diagonals of one of the face of the cube are removed, then Zeff is
View SolutionA compound formed by elements A and B has a cubic structure in which A atoms are at the corners of the cube and B atoms are at the face centres. The formula of the compound will be
View SolutionA compound formed by elements A and B has a cubic structure in which A atoms are at the corners of cube and B atoms are at the face centres. The formula of the compound will be:
View Solution
