Derive the equation for effective focal length for lenses in out of contact.Solution in Tamil
When two thin lenses are separated by a distance d.
(i) Let O be a point object on the principal axis of a lens. OA is the incident ray on the lens at a point A at a height h above the optical centre. (ii) The ray is deviated through an angle δ and forms the image at I on the principal axis. (iii) The incident and refracted rays subtend the angles, ∠AOP=α and ∠AIP=β with the principal axis respectively. In the triangle △OAI , the angle of deviation δ can be written as,
δ=α+β ....(1)
If the height h is small as compared to PO and Pl the angles α,β and δ are also small. Then,
α≈tanα=PAPO, and β≈tanβ=PAPI ....(2)
Then ,δ=PAPO+PAPI ....(3)
Here, PA=h,PO=−u and PI=v
δ=h−u+hv=h(1−u+1v) ....(4)
After rearranging
δ=h(1v−1u)=hf
δ=hf ...(5)
(iv) The above equation tells that the angle of deviation is the ratio of height to the focal length. Now, the case of two lenses of focal length f1 and f2 arranged coaxially but separated by a distance d can be considered as shown in the below Figure.

(v) For a parallel ray that falls on the arrangement, the two lenses produce deviations δ1 and δ2 respectively and The net deviation δ is.
δ=δ1+δ2 ....(6)
From Equation (5),
δ1=h1f1,δ2=h2f2 and δ=h1f ....(7)
The equation (6) becomes,
h1f+h1f1+h2f2 ....(8)
From the geometry,
h2−h1=P2G−P2C=CG
h2−h1=BGtanδ1≈BGδ1
h2−h1=dh1f1
h2=h1dh1f1 ....(9)
Substituting the above equation in Equation (8).
h1f=h1f1+h1f2+h1df1f2
On further simplification,
1f=1f1+1f2+df1f2 ....(10)
(vi) The above equation could be used to find the equivalent focal length. To find the position of the equivalent lens, we can further write from the geometry,
PP2=EG=GCtanδ
PP2=EG=GCtanδ=h1−h2tanδ=h1−h2δ
From equations (7) and (9)
h2−h1=dh1f1 and δ=h1f
PP2=(dh1f1)×(fh1)
PP2=(dff1)
Similar Questions
Two lenses of focal lengths f_1 and f_2 , are placed in contact. Obtain a general expression for effective focal length in terms of f_1 and f_2
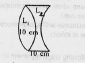
View SolutionTwo lenses L1 and L2 are placed in contact as shown in figures. The focal length of each lens is 10cm.What is effective focal length of combination
View SolutionDerive the expression for the equivalent focal length of a combination of two thin convex lenses in contact.
View SolutionDerive an expression for the equivalent focal length for two thin convex lenses in contact.
View SolutionFind the effective power and focal length of two lenses of focal lengths 50 cm and -200 cm when put in contact. What is the nature of the combined lens?
View SolutionTwo thin lenses of focal length 20 cm and 25 cm are in contact. The effective power of the combination is :
View SolutionTwo thin convex and concave lenses, each of focal length 20 cm are placed in contact. The effective focal length (in cm) is
View SolutionDerive an expression for the effective focal length of two thin lenses in contact.
View Solution
