If λ be the ratio of the roots of the quadratic equation in x, 3m2x2+m(m−4)x+2=0, then the least value of m for which λ+1λ=1, is k−3√2. The value of k is __________.
The correct Answer is:4
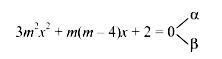
αβ=λ
Sum of roots =βλ+β=−m(m−4)3m2=−(m−43m …..(1)
Product of roots =βλ×β=23m2 …….(2)
From (1) and (2) β2(λ+1)2β2λ=(m−4)26
(λ+1)2λ=(m−4)26p∧q
λ2+2λ+1λ=(m−4)26
λ+1λ=(m−4)26−2
λ+1λ=1⇒(m−4)26−2=1
(m−4)2=18
m−4=±3√2
m=4±3√2.
Similar Questions
Knowledge Check
- Question 1 - Select One
The values of λ such that sum of the squares of the roots of the quadratic equation x2+(3−λ)x+2=λ has the least value is
A2B49C158D1
The value of m for which the roots of the equation x2+(m−2)x+m+2=0 are in the ratio 2:3 is
View SolutionIf ratio of the roots of the quadratic equation 3m2x2+m(m−4)x+2=0 is λ such that λ+1λ=1 then least value of m is (A) −2−2√3 (B) −2+2√3 (C) 4+3√2 (D) 4−3√2
View SolutionIf x=2 andx=3 are roots of the equation 3x2−2kx+2m=0, find the value of k and m.
View Solutiona and b are the roots of the quadratic equation x2+λx−12λ2=0 where x is the unknown and λ is a real parameter. The minimum value of a4+b4 is:
View SolutionFind the value of m for which the quadratic equation , 3x2+10x+(m−3)=0 has roots which are reciproal to each other.
View SolutionIf the roots of the equation x2+3x+2=0 and x2−x+λ=0are in the same ratio then the value of λ is given by
View SolutionMinimum possible number of positive root of the quadratic equation x2−(1+λ)x+λ−2=0 , λ∈R
View Solution

