A cubical block of side 0.5 m floats on water with 30% of its volume under water. What is the maximum weight that can be put on the block without fully submerging it under water?
[Take, density of water =103kg/m3]
87.5 kg
30.1 kg
46.3 kg
65.4 kg
The correct Answer is:A
To solve the problem, we need to determine the maximum weight that can be placed on a cubical block of side 0.5 m that floats on water with 30% of its volume submerged. We will use the principles of buoyancy and Archimedes' principle to find the solution.
1. Calculate the Volume of the Cubical Block:
The volume V of a cube is given by the formula:
V=side3
For a side length of 0.5 m:
V=(0.5m)3=0.125m3
2. Determine the Volume of Water Displaced:
Since 30% of the block is submerged, the volume of water displaced Vd is:
Vd=0.3×V=0.3×0.125m3=0.0375m3
3. Calculate the Weight of the Water Displaced:
The weight of the water displaced Wd can be calculated using the density of water (ρ=1000kg/m3) and the gravitational acceleration (g≈9.81m/s2):
Wd=ρ⋅Vd⋅g=1000kg/m3⋅0.0375m3⋅9.81m/s2
Wd=1000⋅0.0375⋅9.81=368.25N
4. Determine the Weight of the Block:
The weight of the block Wb when it is floating is equal to the weight of the water displaced:
Wb=Wd=368.25N
5. Calculate the Maximum Additional Weight:
Let W be the maximum weight that can be added to the block without fully submerging it. When the maximum weight is added, the total weight of the block plus the additional weight should equal the weight of the water displaced when the block is fully submerged (100% submerged):
W+Wb=ρ⋅V⋅g
The weight of the water displaced when fully submerged:
Wfull=1000⋅0.125⋅9.81=1226.25N
6. Set Up the Equation:
W+368.25=1226.25
7. Solve for W:
W=1226.25−368.25=858N
8. Convert Weight to Mass:
To find the mass m that corresponds to the weight W:
W=m⋅g⟹m=Wg=8589.81≈87.5kg
Thus, the maximum weight that can be placed on the block without fully submerging it under water is approximately 87.5 kg.
The volume V of a cube is given by the formula:
V=side3
For a side length of 0.5 m:
V=(0.5m)3=0.125m3
Since 30% of the block is submerged, the volume of water displaced Vd is:
Vd=0.3×V=0.3×0.125m3=0.0375m3
The weight of the water displaced Wd can be calculated using the density of water (ρ=1000kg/m3) and the gravitational acceleration (g≈9.81m/s2):
Wd=ρ⋅Vd⋅g=1000kg/m3⋅0.0375m3⋅9.81m/s2
Wd=1000⋅0.0375⋅9.81=368.25N
The weight of the block Wb when it is floating is equal to the weight of the water displaced:
Wb=Wd=368.25N
Let W be the maximum weight that can be added to the block without fully submerging it. When the maximum weight is added, the total weight of the block plus the additional weight should equal the weight of the water displaced when the block is fully submerged (100% submerged):
W+Wb=ρ⋅V⋅g
The weight of the water displaced when fully submerged:
Wfull=1000⋅0.125⋅9.81=1226.25N
W+368.25=1226.25
W=1226.25−368.25=858N
To find the mass m that corresponds to the weight W:
W=m⋅g⟹m=Wg=8589.81≈87.5kg
Similar Questions
A body is floating in water with 3/5th of its volume below the water surface. What will be density of the body?
View SolutionA block of wood floats in water two-thirds of its volume submerged. In oil the block of floats with 0.90 of its volume submerged. Find the density of (a) wood and (b) oil, if density of water I 103kg/m3.
View Solution
Knowledge Check
- Question 1 - Select One
A block of wood floats in water with 23 of its volume submerged. Its relative density is
A13B23C43D19 - Question 2 - Select One
Find the density of a block of wood that floats in water with 0.1 of its volume above water
A0.9 g/ccB0.9 kg/ccC0.1 g/ccD0.1 kg/cc - Question 3 - Select One
Find the density of a block of wood that floats in water with 0.1 of its volume above water
A0.9g/ccB0.9g/c cC0.1g/ccD0.1g/c c
A block of wood floats with34th of its volume submerged in water. The density of wood is ________
View SolutionA cubical block of wood of edge 3 cm floats in water. The lower surface of the cube just touches the free end of a vertical spring fixed at the bottom of the pot. Find the maximum weight that can be put on the block without wetting it. Density of wood =800kgm−3 and spring constant of the spring =50Nm−1Takeg=10ms−2.
 ,View Solution
,View SolutionA block of wood floats with 1//4 of its volume under water. What is he density of wood? (Density of water =1000kg/m3)
View SolutionA cubical block of side 10 cm floats at the interface of an oil and water. The pressure above that of atmosphere at the lower face of the block is
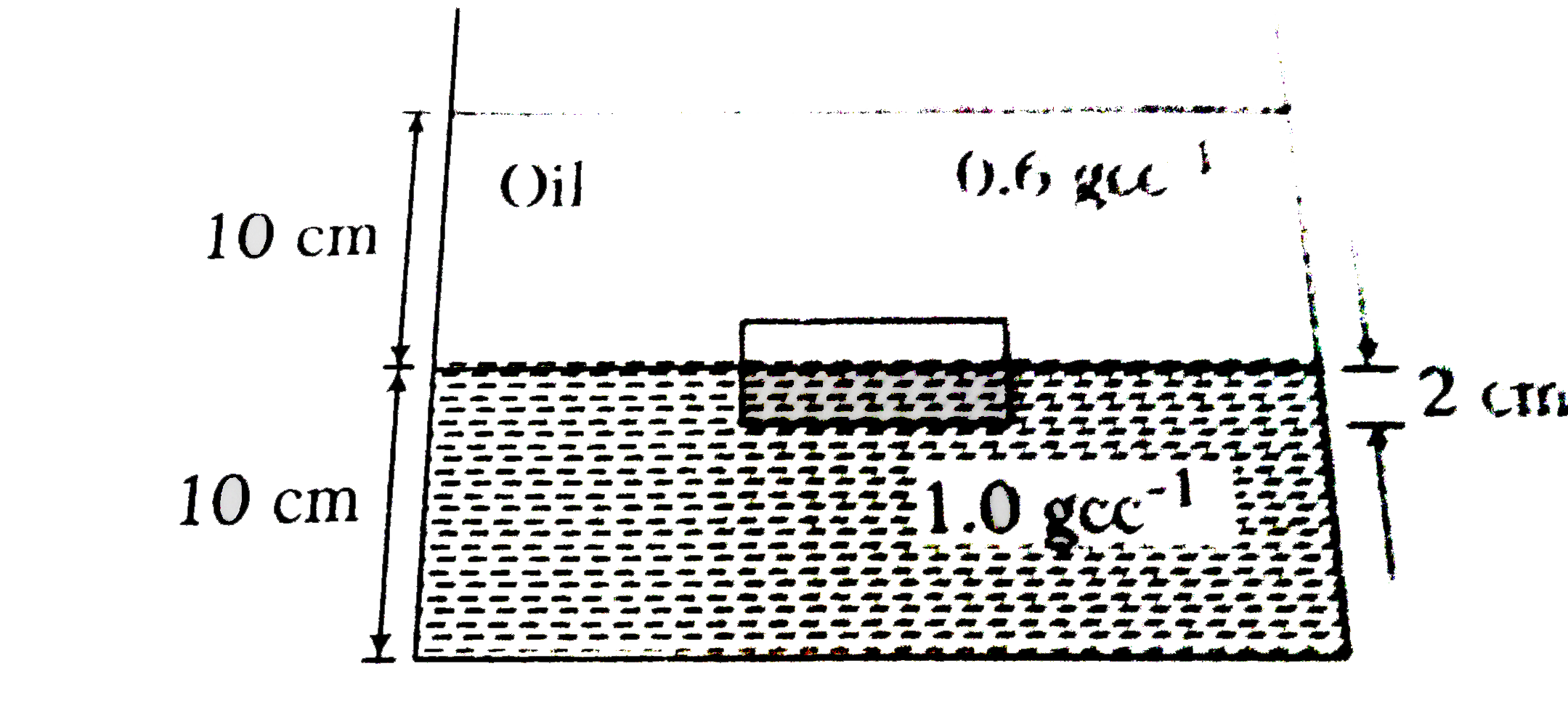 View Solution
View SolutionIf the bulk modulus of water is 2100 Mpa, then speed of sound in water is (take density of water, ρ=1000kg/m3)
View Solution

