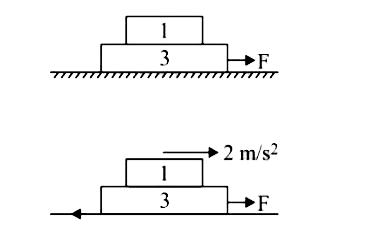
Two blocks A and B of masses mA=1kgandmB=3kg are kept on the table as shown in figure. The coefficient of friction between A and B is 0.2 and between B and the surface of the table is also 0.2. The maximum force F that can be applied on B horizontally, so that the block A does not slide over the block B is ________ (in N): [Take g=10m/s2]
![Two blocks A and B of masses m(A) = 1kg and m(B) = 3kg are kept on the table as shown in figure. The coefficient of friction between A and B is 0.2 and between B and the surface of the table is also 0.2. The maximum force F that can be applied on B horizontally, so that the block A does not slide over the block B is (in N): [Take g = 10 m//s^(2)]](https://d10lpgp6xz60nq.cloudfront.net/physics_images/VMC_JEE_REV_TST_13_E01_025_Q01.png)
The correct Answer is:16
max. acceleration of 1 kg block
=fmax1=μ×1×g1=2m/s2
When maximum F is applied on 3 kg
fk=0.2×4×10=8
F−8=(3+1)×2
F=16N
Similar Questions
Consider two blocks A and B of mass 3 kg and 9 kg respectively. Now, block A is placed on block B which is at rest on a table where the coefficient of friction between A and B is 0.4 and between B and the surface of the table is also 0.4. A force F is applied on B horizontally such that the block A does not slide over B. The maximum value of force F is ________N . (Take g=10m/s2)
View SolutionTwo block "A&B" of mass MA=1kg&MB=3kg are kept on a smooth table as shown in the figure.The coefficient of friction between "A&B" is "0.2" .The maximum force "F" that can be applied on "B" horizontally,so that the block "A" do not slide over the block "B" is Take g=10ms2andk=400Nm)
View Solution
Knowledge Check
Two blocks A and B of mass 10 kg and 20 kg respectively are placed as shown in figure .Coefficient of friction between all the surface is 0.2 (g=10m/s2) Then,
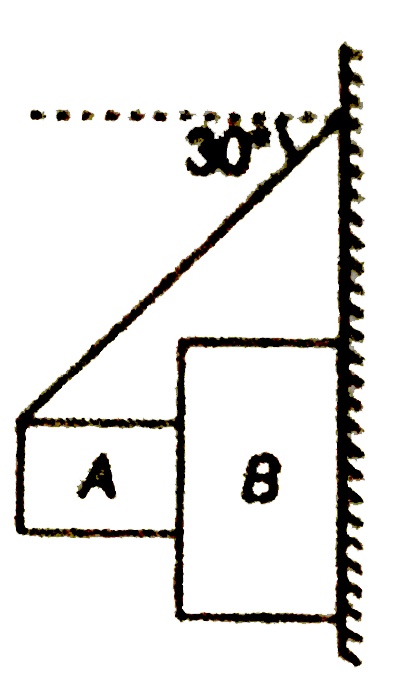 ATension in the string is 306 NBTension in the sr=tring is 132 NCaccelerration of block B is 2.6m/s2Dasseleration of block B is 4.7m/s2
ATension in the string is 306 NBTension in the sr=tring is 132 NCaccelerration of block B is 2.6m/s2Dasseleration of block B is 4.7m/s2A block A of mass 100 kg rests on another block B of mass 200 kg and is tied to a wall as shown in the figure. The coefficient of friction between A and B is 0.2 and that between B and ground is 0.3. The minimum force required to move the block B is (g=10ms−2)
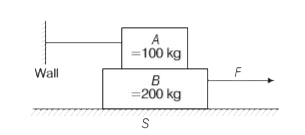 A900 NB200 NC1100 ND700 N
A900 NB200 NC1100 ND700 NTwo blocks A and B are lying on the table as shown in the figure. The coefficient of friciton between block A and block B is μ. The coefficient of friction between block B and ground also μ. An external force F is applied in the direction shown. The maximum value of the force F that can be applied without moving the blocks.
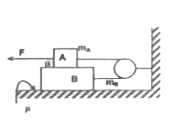 Aμ(2mA+mB)gBμ(mA+mB)gCμ(2mA+mB/2)gDμ(3mA+mB)g
Aμ(2mA+mB)gBμ(mA+mB)gCμ(2mA+mB/2)gDμ(3mA+mB)g
The coefficient of static friction between the two blocks shown in figure is μ and the table is smooth. What maximum horizontal forced F can be applied to he block of mass M so that the block move together?

View SolutionA block A of mass 3kg and another block B of mass 2kg are connected by a light inextensible string as shown in figure. If the coefficient of friction between the surface of the table and A is 0.5 What maximum mass C is to be placed on A so that the system is to be in equlibrium
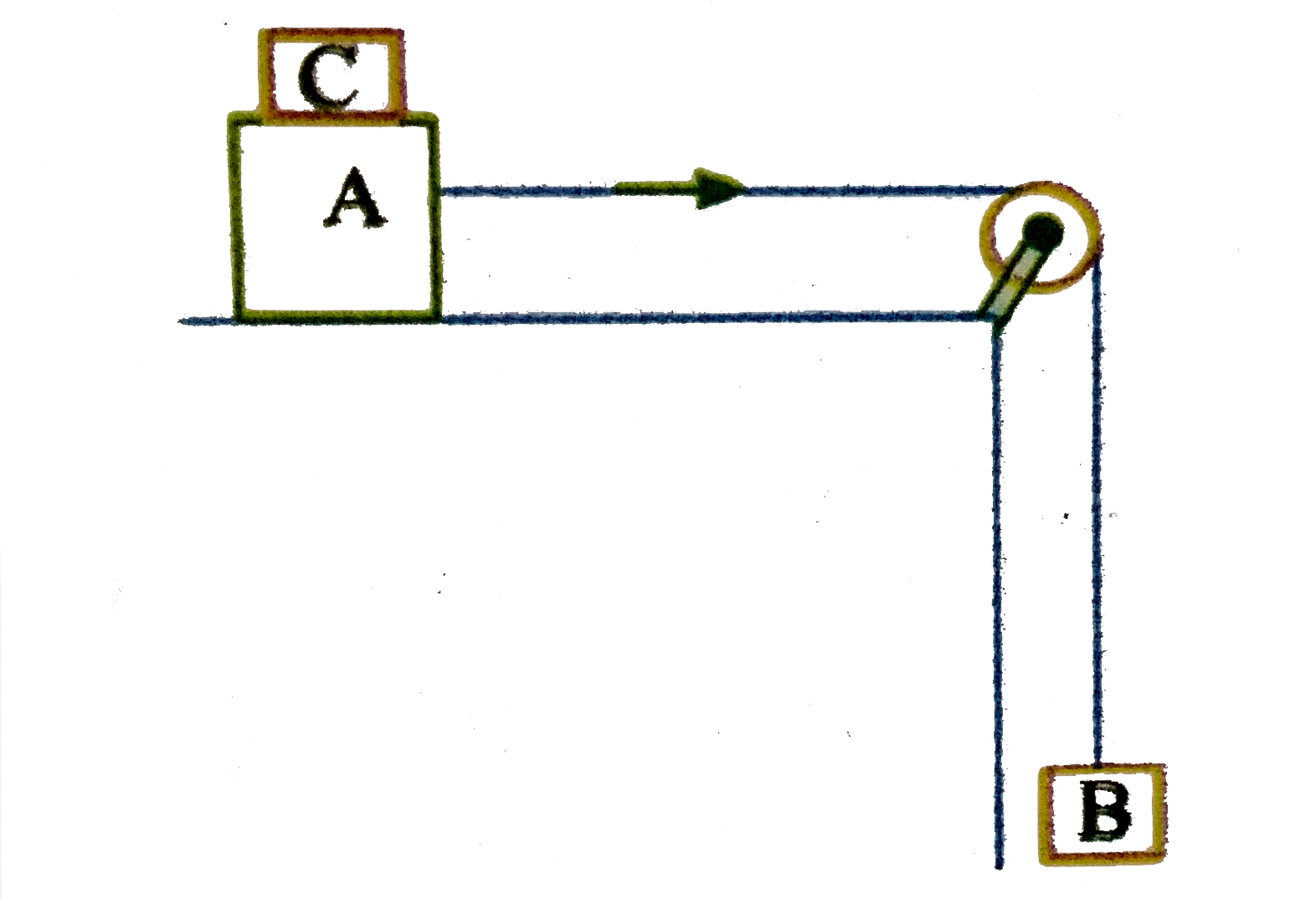 .
.
View SolutionA block A of mass 3kg and another block B of mass 2 kg are connected by a light inextensible string as shown in figure. If the coefficient of friction between the surface of the table and A is 0.5. What maximum mass C is to be placed on A so that the system is to be in equilibrium ?
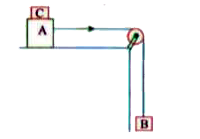
View SolutionTwo block A and B of masses 5 kg. and 3 kg. respectively rest on smooth horizontal surface with B over A. The coefficient of friction between A and B. 0.5 the maximum horizontal force that can be applied to A so that there will be motion of A and B without separation is :-
View SolutionA block A of mass 2 kg rests on another block B of mass 8 kg which rests on a horizontal floor. The coefficient of friction between A and B is 0.2 while that between B and floor is 0.5. when a horizontal force of 25 N is applied on the block B. the force of friction between A and B is
View Solution
