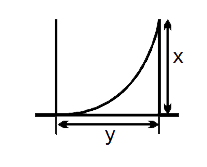
A positively charged particle of charge 'q' enters in a uniform magnetic field B directed inward and is deflected a distance x after travelling a distance Y as shown in the figure. The magnitude of linear momentum of the particle is:
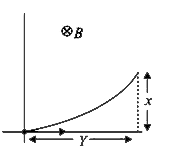
qBY2
qB2(x2Y+x)
qB2x(x2+Y2)
qB2Y(x2+Y2)
The correct Answer is:C
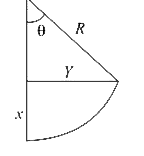
Rsinθ=Y
R(1−cosθ)=x
⇒(1−xR)2+(YR)2=1⇒R=12x(x2+Y2)
⇒p=mv=qB2x(x2+Y2)
Similar Questions
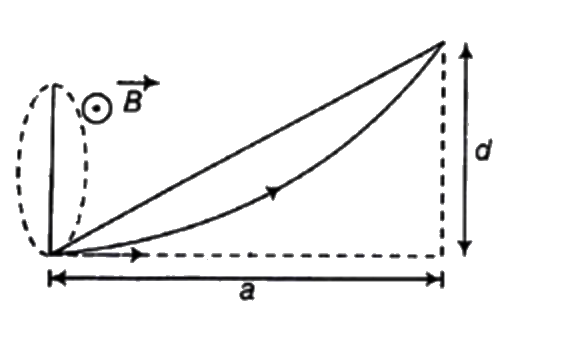
A charged particle q enters a region of uniform magnetic field B (out of page) and is deflected distance d after travelling a horizontal distance a. The magnitude of the momentum of the particle is
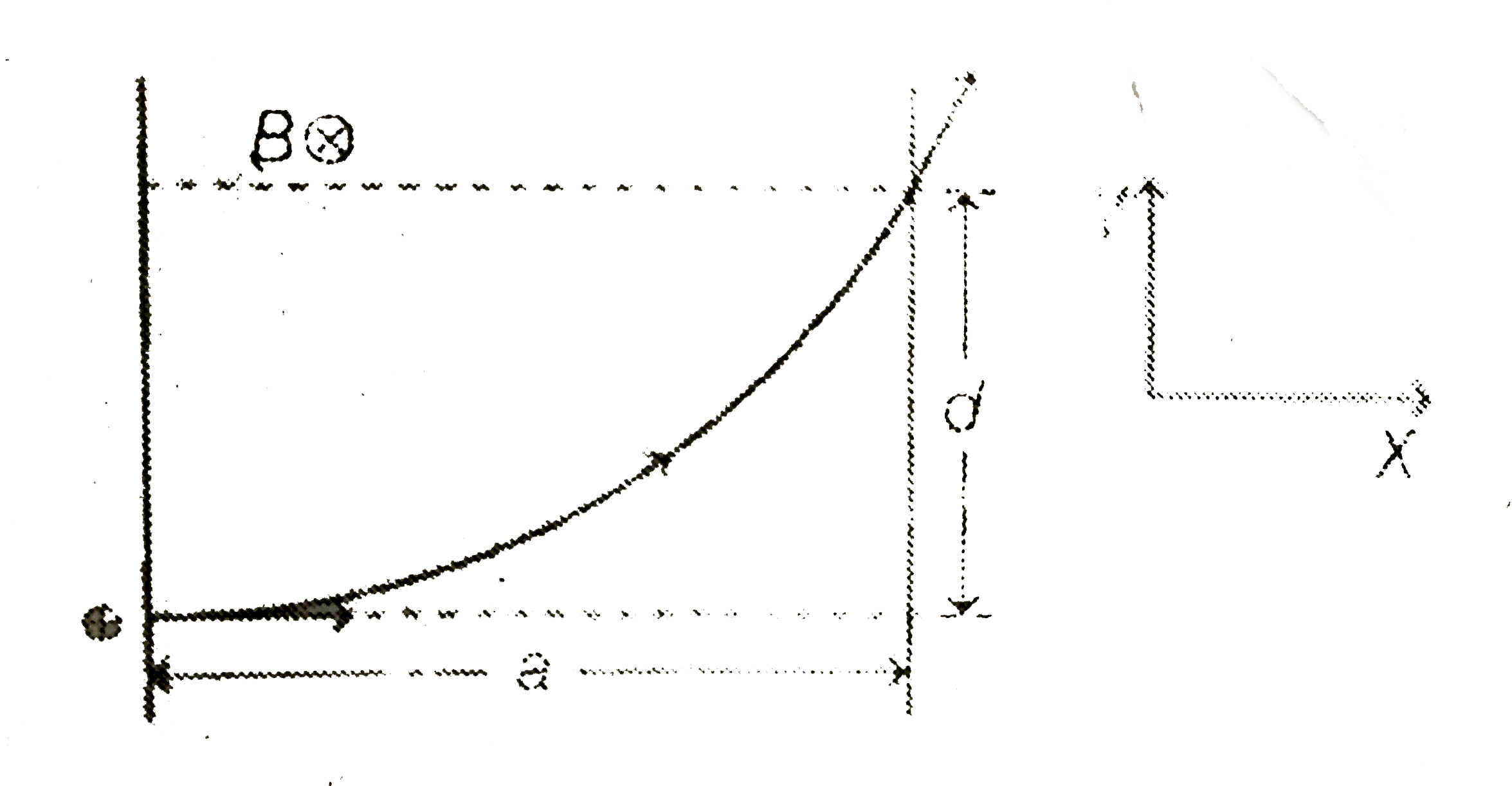
View SolutionA particle of charge+q eneters a region of uniform magnetic field B (directed into the plane of paper) as shown in the figure. Particle is deflected by a distance d along y-axis after travelling a distance of a along x axis. Find magnitude of linear momentum of particle in Newton-sec. (Given: a=3m, d=4m, Bq=032c-Tesla)
View Solution
Knowledge Check
A particle having charge q enters a region of uniform magnetic field →B (directed inwards) and is deflected a distance x after travelling a distance y. The magnitude of the momentum of the particle is
 AqBy2BqByxCqB2(y2x+x)DqBy22x
AqBy2BqByxCqB2(y2x+x)DqBy22xA charged particle enters into a uniform magnetic field the parameter that remains constant is
AvelocityBmomentumCkinetic energyDangular velocityIf a charged particle enters perpendicular in the uniform magnetic field then
AEnergy remains constant but moment changesBEnergy and momentum both remains constantCMomentum remains constant but energy changesDNeither energy nor momentum remains constant
A positively charged particle moving due east enters a region of uniform magnetic field directed vertically upwards. The partical will be
View SolutionWhen a charged particle enters a uniform magnetic field its kinetic energy
View SolutionWhen a charged particle enters a uniform magnetic field its kinetic energy
View SolutionA charged particle enters a uniform magnetic field perpendicular to it. The magnetic field
View SolutionA charge q is placed in an uniform electric field E. If it is released, then the K.E of the charge after travelling distance y will be :
View Solution
