Find the length of latus rectum L of ellipse $\frac{x^{2}}{A^{2}} + \frac{y^{2}}{9} = 1, (A^{2}) > 9)$ where $A^{2}$ is the area enclosed by quadrilateral formed by joining the focii of hyperbola $\frac{x^{2}}{16} - \frac{y^{2}}{9} = 1$ and its conjugate hyperbola

A

$\frac{18 \sqrt{2}}{5}$

B

$\frac{5}{18} \sqrt{2}$

C

$\frac{18}{5 \sqrt{2}}$

D

$\frac{9}{5 \sqrt{2}}$

Video Solution

Text Solution

Verified by Experts

The correct Answer is:C

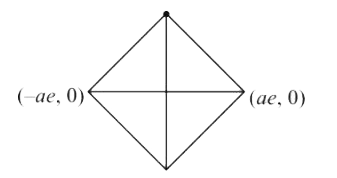
Area of quadrilateral by joining focii of hyperbola and its conjugate hyperbola is $2 {(a e)}^{2}$
i.e., $2 (5^{2}) = 50$
$∴$ i.e., $A^{2} = 50 \Rightarrow A = 5 \sqrt{2}$
Now length of laths rectum $= \frac{2 b^{2}}{a}$
$= \frac{2.9}{5 \sqrt{2}} = \frac{18}{5 \sqrt{2}}$

|

Updated on:21/07/2023

Class 12 MATHS QUESTION BANK

Similar Questions

Knowledge Check

Question 1 - Select One
The foci of the hyperbola $\frac{x^{2}}{16} - \frac{y^{2}}{9} = 1$ is :
A(0,5)
B(5,0)
C $(\pm 5, 0)$
D $(0, \pm 5)$
Question 2 - Select One
The foci of the hyperbola $\frac{x^{2}}{9} + \frac{y^{2}}{16} = 1$ are-
A $(0, \pm 3)$
B $(\pm 3, 0)$
C $(\pm 5, 0)$
DNone of these
Question 3 - Select One
The length of latus rectum of the ellipse $4 x^{2} + 9 y^{2} = 36$ is
A $\frac{4}{3}$
B $\frac{8}{3}$
C6
D12

Recommended Questions

Home
Ask Doubt
Profile