Two particles of mass m & 2m have velocities uˆj & uˆi respectively . They collide completely inelastically. Find the loss in kinetic energy of the system.
mu2
23mu2
mu24
mu24
For the system 1Pi=2muˆi+muˆj
As all the impulses are internal , 1Pi=1Pf
1Pf=3mruf . Where ruf is the velocity of the combined mass after inelastic collisoion
Thus 3mruf=2muˆi+muˆj, ruf=2u3ˆi+13ˆj⇒∣∣ruf∣∣=√59u
Thus final energy of the system =12×3m×∣∣ruf∣∣2=12×59u2=5mu26
Initial energy of the system =m1v212+m2v222=12mu2+12×2m×u2=32mu2
Loss in K.E. =[ Initial K.E. ) - ( Final K.E. )]
Loss =32mu2−56mu2=23mu2
Similar Questions
Two particles of mass 2m and m moving with speed v and 2v respectively perpendicular to each other collides perfectly inelastically, then
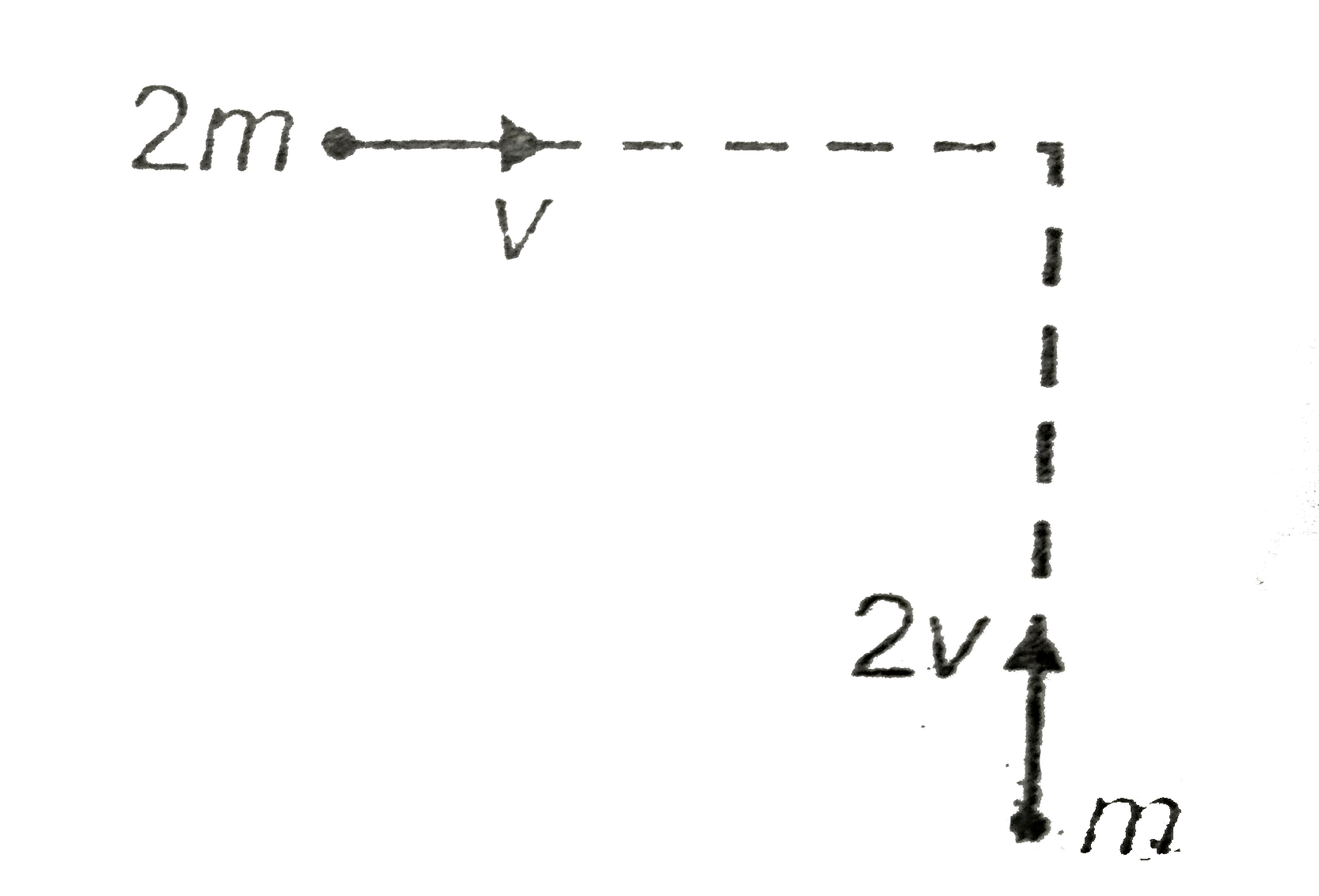
Loss in kinetic energy of the system
View SolutionA particle of mass m moving with velocity 2v collides with another particle of mass 3m moving with velocity v in the same direction.If it is perfect inelastic collision,the loss of kinetic energy of the system is
View Solution
Knowledge Check
Two particles of equal mass m are moving with velocities vˆi and v(ˆi+ˆj2) respectively. If the particles collide completely inelastically, then the energy lost in the process is
A3mv24B2mv23Cmv23Dmv28Two identical mass M moving with velocity u1andu2 collide perfectly inelastically. The loss in kinetic energy is
AM2(u2−u1)2BM2(u21−u22)CM4(u2−u1)2DM4(u21−u22)Two perfectly elastic balls of same mass m are moving with velocities u1 and u2. They collide elastically n times. The kinetic energy of the system finally is:
A12m∪21B12mu(u21+u22)C12m(u21+u22)D12mn(u21+u22)
A particle of mass m moving with speed u collides perfectly inelastically with another particle of mass 2m at rest. Find loss of kinetic energy of system in the collision.
View SolutionA bullet of mass m moving with velocity u on smooth surface strikes a block of mass M kept at rest. The collision is completely inelastic. Find the common velocity and the fractional loss in kinetic energy.
View SolutionTwo particles of mass 1 kg and 2 kg are moving with speed 2 m/s and 5 m/s as shoen in the figure, they collides perfectly inelastically. Find
(a) Their final velocity
(b) Loss in kinetic energy
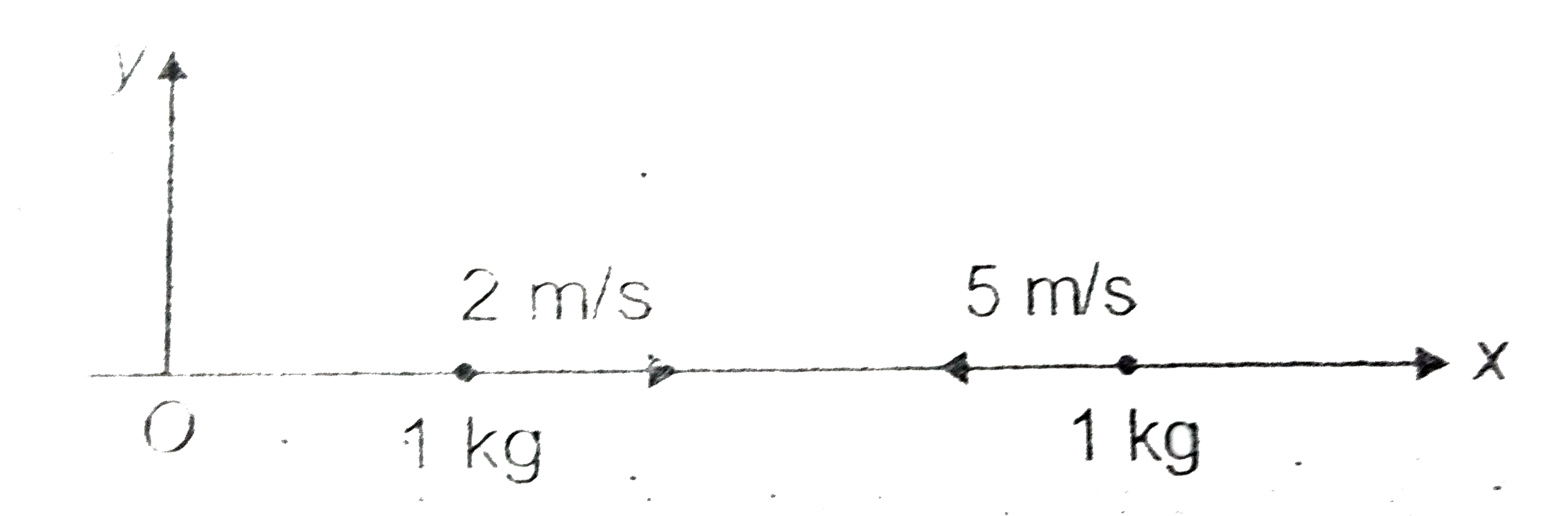
View SolutionTwo particles of same mass m moving with velocities u1 and u2 collide perfectly inelastically. The loss of energy would be :
View SolutionA ball of mass '2m' moving with velocity uˆi collides with another ball of mass 'm' moving with velocity −2uˆi. After the collision, mass 2m moves with velocity u2(ˆi−ˆj). Then the change in kinetic energy of the system ( both balls ) is
View Solution
